Section Summary: 7.1 - Inverse functions

1. Definitions
   • one-to-one function: A function f is called a one-to-one function if it never takes on the same value twice; that is,

     

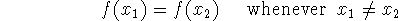
     This means that each point in the range has a unique pre-image (element of the domain).

   • inverse function: Let f be a one-to-one function with domain A and range B. Then its inverse function 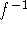has domain B and range A and is defined by

     

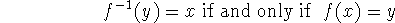
     for any y in B.

2. Theorems
   • If f is a one-to-one function defined on an interval, then its inverse function 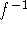is also continuous. Because the graph of 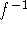is simply the mirror reflection of the graph of f, the continuity of f is also reflected in the mirror.
   • If f is a one-to-one differentiable function with inverse function and , then the inverse function is differentiable at a, and

     

   Differentiability is really about smoothness, and this too is reflected in the mirror of the line y=x. The only problem that can arise is if the slope of f is 0 at a point, because this will be reflected as an infinite slope of the inverse function (alternatively, an infinite slope of f turns into a 0 slope of ).

3. Properties/Tricks/Hints/Etc.
   • Horizontal line test: A function is one-to-one if and only if no horizontal line intersects its graph more than once. This is of course akin to the vertical line test. It simply says that a graph could represent a function either of x as a function of y, or y as a function of x.
   • domain of 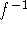= range of f; range of 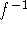= domain of f. We can think of these two functions as just ways of passing back and forth between two sets.
   • Caution: do not mistake the -1 in 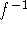for an exponent: 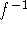does not mean .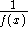

   • 

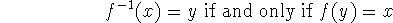
   • Cancellation equations:

     

     

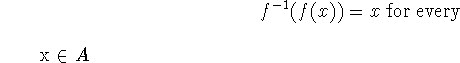
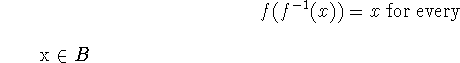
   • Method for finding the inverse of a function:
     1. Write y=f(x).
     2. Solve this equation for x in terms of y (if possible).
     3. To express 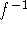as a function of x, interchange the roles of x and y: that is, write the resulting equation as .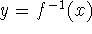
   • The graph of 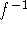is obtained by reflecting the graph of f about the line y=x.
   • Functions that are not one-to-one can be made so by restricting the domain. For example, is not one-to-one (since 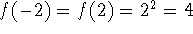, but we can restrict its domain to positive values of x and then write the inverse as .

4. Summary

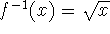   In this section we introduce the inverse function, which is the mirror image of f about the line y=x. Hence, properties of are also mirror reflections of those properties of f. It's easy to graph , for example, once we have the graph of f, and properties of continuity and differentiability are also easy to deduce. Even asymptotes are reflected! It's even easy to compute slopes at a point on the inverse function if we know the derivative of the function f.

   One can think of f and as ways of passing between two sets (the domain and range of f, which are the range and domain of ). A secret code is an example of a function and its inverse: if I give you a secret message, it's tranformed from an ordinary expression (the domain) by a function and passed into a strange form (in the range). The secret agent on the other end needs a method (the inverse function) that turns strange forms into ordinary expressions.

Problems:

Problems, pp. 414-416, #2-10, 12, 14, 16, 22, 23, 34, 38

In class: #7-10, 13, 15, 24, 33, 36