Section 3.10 Worksheet:

Assigned problems: Exercises pp. 211-212, #2-6, 10, 11, 12, 18, 44, 47 (due Wednesday).

1. What is the linearization of function f at a? Give a graphical description, too.

2. In Example 2, p. 207, how do we determine that an approximation is either an under-estimate, or an over-estimate?

3. Describe the distinction between the differential dy and the increment .

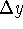4. What is the difference between the error of an approximation and the relative error?

5. What reasons do you find in this section for why approximation is necessary, or useful? Why not just use the exact formula for a function?

Notes:

1. We often find it necessary to approximate complicated functions by simpler functions, and the simplest sort of useful functions are linear. Approximating a function by a linear function simplifies things considerably, but at the cost of introducing errors, of course: it is an approximation we obtain, after all, rather than the exact answer.