![]()
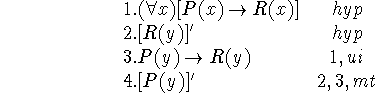
and find the flaw in the ``proof'' given.
Let x and y have domain the reals, and Q(x,y) be the condition that
Find the flaw in the ``proof'':
In step 4, the student attempted universal generalization from step 3, which
was deduced by existential instantiation of step 2, in which y was free.
Exercise #11, p. 57
![]()
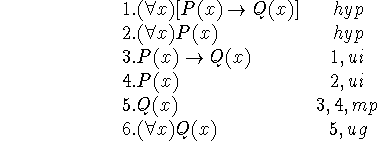
Exercise #3, p. 56
![]()
![]() . Then the antecedent above says that for every real y, there is
another real x such that
. Then the antecedent above says that for every real y, there is
another real x such that ![]() - every real has a square in the real
numbers. However, the consequent says that there is a real number x which is
the square of all reals! False.
- every real has a square in the real
numbers. However, the consequent says that there is a real number x which is
the square of all reals! False.

Practice 24, p. 47
![]()
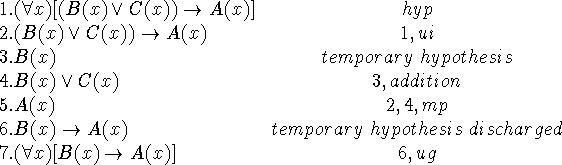
LONG ANDREW E
Thu Jan 17 18:59:37 EST 2002