Selections from Julia E. Diggins, String, Straightedge, and Shadow Viking Press, New York , 1965. (Illustrations by Corydon Bell)

14. THE UNSPEAKABLE TRAGEDY

Before the Secret Brotherhood was disbanded, its members really thought they had grasped the key to the cosmos.

Then everything collapsed. Their whole scheme was destroyed by a fatal discovery, and the Order itself was destroyed by traitors and mob violence. Yet as we retell the somber tale, we will find that it was not a complete tragedy after all, for the Pythagoreans did enjoy their cosmic key briefly. This key was not found in abstract shapes alone nor in music, nor in the stars, but in one factor that-they believed-linked all of these: number.

"Himself" had said it: "Everything is number!"

So they followed Pythagoras teaching that the universe was ruled by whole numbers That did not mean numbers for ordinary counting or calculating. What interested them was the nature of a number itself odd even, divisible, indivisible and the relations between numbers. This was their arithmetike. And they applied it to their other three fields, and found startling number patterns in each.

In music, for instance, a sensational discovery about the relations of whole numbers and musical intervals was attributed to Pythagoras himself.

115

One legend said that on his long voyages he listened to the music of flapping sails, and the wind whistling and whining through the ship's rigging and playing a melody on the ropes. And that he decided then and there to investigate the connection between the tempest of sounds and the vibrating strings.

Another version said that he was strolling through the village of Croton, deep in thought, listening to the musical sounds of hammers striking anvils in a blacksmith's shop; when suddenly, tripping on a taut string that some children had stretched across the street, he got the inspiration for an experiment.

But the most popular story told that the idea came to him straight from the stringed lyre of his "father" Apollo, who was also the god of music.

Anyway, Pythagoras experimented with stretched strings of different lengths placed under the same tension. Soon he found the relation between the length of the vibrating string and the pitch of the note. He discovered that the octave, fifth, and fourth of a note could be produced by one string under tension, simply by "stopping" the string at different places: at one-half its length for the octave, two-thirds its length for the fifth and three-fourths its length for the fourth!

Other musical innovations were credited to him, such as a one-string apparatus for the study of harmonics. But his great discovery was the tetrachord, where the most important harmonic intervals were obtained by ratios of the whole numbers:

1, 2, 3, 4. The Secret Brotherhood gave this fourfold chord mystical significance and used to say: "What is the oracle at Delphi? The tetrachord! For it is the scale of the sirens."

And the Pythagoreans even used it for their astronomy. In the relation of number and music, they believed they had found

116

the pattern that guided the "wandering" planets through the heavens. They pictured the sun and the planets as geometrically perfect spheres, moving through the visibly circular sky on perfect circular orbits, separated by harmonic ratios-musical intervals! Theirs was a vision of time and space revealed in lines, tones, and mathematical ratios. And they even imagined the brilliant planets emitting harmonious tones, the so-called "music of the spheres."

But it was in the connection of number and geometry, their two completely mathematical subjects, that the Pythagoreans were on surest ground.

117

Numbers, they had discovered, whole numbers, actually had geometric shapes. There were triangular numbers, square numbers, pentagonal numbers, rectangular numbers, and so on.

This was no wild fantasy, like the singing planets. It was a real mathematical discovery, and came from the circumstance that they did not do their number work by writing the numbers at all. Instead, they placed pebbles on the sand, like the reckoners. But the Pythagoreans placed their pebbles in patterns, adding extra rows for each number. Their two most important series were the square numbers and the triangular numbers.

The most important number of all, to the Pythagoreans, was the fourth triangular number, 10. For it was made up of 1 + 2 + 3 + 4. They called it the "Sacred Tetractys, " swore by it in their oaths, and attached marvelous properties to it, as "the source and root of eternal nature."

118

Everything fitted perfectly: the Tetractys, the tetrachord, the four regular solids representing the four "elements," inscribed in a dodecahedron representing the celestial sphere. But it was all too pat a jumble of luck, imagination, serious mathematical experiments, and old number magic from the East. just as the Pythagoreans thought they were getting more and more evidence that number was everywhere, the whole system broke down. The entire connection between geometry and number-the foundation of their thinking-was shattered by one disastrous experiment.

Presiding was Hippasus of Metapontum, whose name was to loom dark in their future of the Brotherhood. The idea was simply to find the numbers that matched the sides of the two right triangles with which Pythagoras had first demonstrated his theorem-the Egyptian triangle and the one from the tiled floor.

119

Of course, the Egyptian rope-triangle worked perfectly: its 3-4-5 sides made a beautiful Pythagorean series. They indicated the intervals with pebbles. Now what about the right triangle from the Greek tile design, where the two sides were equal?

Suppose each side had a length of 1 unit-that would require 1 pebble. Then for the hypotenuse-how many pebbles should they put there? Well, the sum of the squares on the sides would equal the square on the hypotenuse. Therefore,

12 = 1 (square on one side)

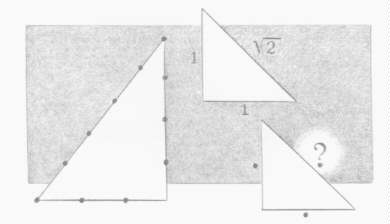
and 12 = 1 (square on other side)

and 1 + 1 = 2,

so 2 is the square on the hypotenuse. And the hypotenuse is the square root of 2.

But what was the square root of 2?

It couldn't be a whole number, since there is no whole number between 1 and 2.

120

Then was it a ratio of whole numbers between 1 and 2? Hopefully, they tried every possible ratio, multiplying it by itself, to see if the answer would be 2. There was no such ratio.

After long and fruitless work, the Pythagoreans had to give up. They simply could not find any number for the square root of 2. We write the answer as 1.4141..., a continuing decimal fraction, but they couldn't do that since they had no concept of zero and of decimals. They could draw the hypotenuse easily, but they could not express its length as a number. It was "unutterable"-"unspeakable"!

Horrified, the Pythagoreans called the square root of 2 an irrational number. After that, they found other irrationals and swore to keep them secret, for the discovery of these "irrationals" wrecked their entire beautifully constructed system of a universe guided by whole numbers. The breakdown in their mystical morale was followed by the breakup of the Secret Brotherhood itself.

In this final demolition, Hippasus played a decisive role, though his own fate is shrouded in mystery. The Order was already in trouble. Bitter resentment had grown up against its secrecy and exclusiveness, and riots of villagers had driven it out of Croton. Pythagoras himself had died on a neighboring island. And now mobs of "democrats" began to attack the aristocratic Pythagorean societies everywhere.

Against this background, Hippasus took a step that was regarded by the conservative members as sheer betrayal. He broke the oath of secrecy and revealed their most closely guarded discoveries-the dodecahedron and the irrationals. When they promptly expelled him, he set himself up as a public teacher of geometry.

The traitor's punishment was swift and terrible. He was very

121

shortly drowned in a mysterious "accident" at sea, and strange rumors circulated. Some said that a storm had struck his ship as a direct vengeance from the gods; others, that he had been pushed overboard by agents of the Secret Brotherhood. But Hippasus' death was to no avail. The harm was already done to the Order of Pythagoreans, though the discovery of irrational numbers eventually worked for the good of mankind.

The remaining secret groups soon collapsed, torn by outer violence and inner dissensions. And more and more "mathematicians" followed Hippasus' example and came out to earn a living as teachers. Pythagoras' idea had been demolished: no longer was there a closed Brotherhood of followers, bound together by a mystical belief in a cosmos ruled by number. Yet his ideals lived on in this broader field. He had pursued knowledge for its own sake, loving wisdom for itself. He knew learning could be shared without diminishing, that it lasts through life and immortalizes the learned after death. And the destruction of the Order gave his legacy to the world.

Geometry was now out in the open-and it was the new Pythagorean geometry. True, mathematics was still mixed with some magic: number mysticism, cosmic ideas about the regular solids. Burt there was, in addition, the famous theorem and its applications, the careful study of shapes, the theory of numbers, and the discovery of irrationals.