Section 1.2 Worksheet:

Assigned problems: Exercises pp. 35-37, #1, 2, 3 , 4, 6, 9, 13, 14, 16 (use regression line y = 4.8567x -220.967; no need to find it yourself). For exercises #3 and 4, use your calculator to plot the graphs as a check. (Due Friday.)

1. What is a mathematical model?

2. What are the four steps in the modeling process?

3. The most important model mathematicians use is the linear model,

   

   What is the graphical significance of m and b?

4. What is the objective of an investigator using linear regression? (This is a very common method used in many applications.)

5. Polynomials are fundamentally important: how do the terms degree and coefficient relate to polynomials?

6. Is a power function necessarily a polynomial? Sometimes a polynomial?

7. How does an empirical model differ from a theoretical model?

8. What are the periods of the functions , , 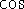and 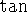? What identities tell us?

Notes:

1. Don't fret about algebraic versus transcendental functions.
2. Properties of trig functions are important! You do yourself a favor to familiarize yourself with them (especially , , 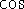and ).
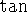3. In this class avoid root notation (e.g. 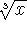); use power notation instead ( ).
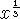4. Become acquainted with the general shapes of the various functions.