- Return Quiz on Series
- Homework on sequences is returned today. From the grader:
- #2: There were a lot of people who couldn't rewrite the sequences.
- #22 Nobody showed that
(so I gave full credit if they wrote down the correct limit).
- #27: most did not use the limit definition
- #30: Most did not use the fact that
to get x's in the denominator.
- #34: most did not show how
- #50: Most just invoked limits without showing anything
- #60: Need to use the squeeze theorem!
- Your exam (next time -- this Thursday) will cover through 11.1. There are hence only four sections covered: 8.1, 8.6, 9.4, and 11.1.
- Your homeworks will serve as a guide, but you should also be ready
for some questions that check your understanding of the theory. Be sure
that you're familiar with the definitions, theorems, and corollaries
("hammers") of each section.
The closest exam I could find is from a section of 229 from last year. You might want to look at that for the style of question you may find.
I suggest that you visit our web homepage, and go through the days from day 1. That's a good way to recall just what we've done.
- Your homework on series will be due 10/8 (I pushed it back a couple of days), after the first exam.
- Read Section 11.3 for next week, Tuesday, responding to the worksheet for section 11.3.
- A handout concerning series.
- Once we have some series in hand, we might want to start operating with them. In particular, as we saw in our very first series, we might want to add up the terms:
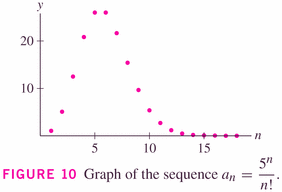
In particular, we expect that, if we throw in the
term, we should get
Such as sum is called an infinite series.
- The question which presents itself is this: what do we mean by this sum of an infinite number of terms? This is indeed curious.
- Here's how we make sense of that infinite sum:
- From the sequence
we define a new sequence
:
These are called partial sums. If
Then we say that
exists, and is equal to L. More formally,

Now geometric series are sufficiently important that it's useful to include that special case:

There's a really fun proof of the first part above, based on a trick. Let's have a look at it.

This theorem tells us that if a sequence isn't asymptotic to the x-axis, we can forget about its partial sums converging.
Finally, series behave the way we'd hope:
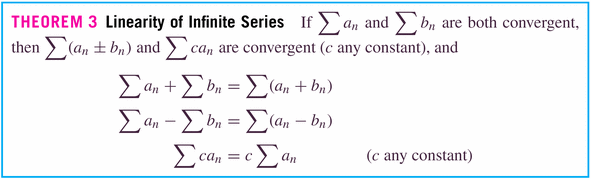
- Examples:
- #7, p. 554
- #8, p. 554 (compare to Taylor series error bound)
- Reflect on #36, p. 555. Recursive actions and definitions give rise to these types of geometric sums.
- Along the same lines: #45, p. 555
- #52, p. 556
- Beautiful spiral "proofs" of some infinite series
- From the sequence