Day 26 in MAT227

Today:

• Announcements:
  • Assignment 8.2 not yet graded -- sorry....
  • Exam is graded, however:
    • Curve: +7 points, and you can get 40% of total missed points back if you'll correct the exam. This correction is due 12/1.
    • Mean (with curve): 73.7/100
    • SD: 16.6
    • I want to apologize for an error on Problem 4 -- my question to you is, why did no one bring it to my attention?

• Last time we talked about differential equations, albeit briefly.
  • You've already solved a differential equation:
    

    The solution is -- so there's an unknown constant k (in this case, it corresponds to the "initial value"). If we tell what y equals at t=0, then we'll get the particular solution, (and that's often very important, that we get the exact solution and unique solution given the data).

    We know that exponential functions have the property that the derivative is proportional to its function value (and is the special function for which the constant of proportionality is exactly 1).

  • As indicated on page 505, differential equations can be a little different:

    

    or

    

    The first doesn't involve y, and the second involves the second derivative of y.

  • The general method for solving differential equation is to stare at them until the solution comes to you!;): what are solutions to the two differential equations above?

  • Today we'll do a lab in which we consider "Newton's Law of Cooling". Before we start, however, let's make up a few differential equations of our own: consider the following functions, and figure out differential equations that they solve:
    • 
    • 
    • 
    We could play this game forever, and get lots of interesting differential equations. But where do differential equations appear in real life?

  • Here's one place: Newton's Law of Cooling (Lab)
  • and

• Section 12.1: parametric curves
  • And now for something completely different....

    A good introduction to parametric curves is given by ballistics. If we shoot a bullet into the air with speed v horizontally, and we neglect all forces but gravity, then the bullet will trace out a parabola:

    

    Now how might we characterize the path of the bullet? The answer is a parametric curve, of the form C(t)=(x(t), y(t)).

    or
    If we wish, we can solve for t in the equation for x and use that to eliminate the parameter t from the equation for y, hence getting an equation for the parabola traced out:

    Orbits of planets in the heavens, movements of ants on a hill, a robotic arm in an assembly plant: all these can be described by parametric curves.

  • A couple of famous examples involving the cycloid:
    
    • The Brachistochrone Problem (1696, solved by Newton in a single day after he heard the challenge)
    • The Tautochrone Problem (1673)

  • Parametrizing a line:
    

  • Parametrizing a circle:
    

• There are two distinctively different kinds of derivatives that we're going to have to consider here:
  • There are the time-derivatives, which give us the actual speeds of the "particles" as they zip along in time. For the bullet, the speed will be
    

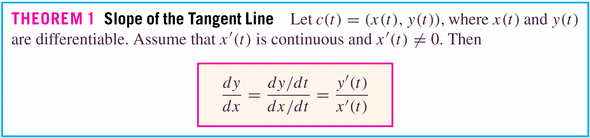
  • There is the slope of the tangent line to the curve traced out by the particle, which one can obtain using the time-derivatives:
    

• One of the most important applications of parametric curves is in the Bezier curve section, p. 609. There are lots of important instances where we need to be able to design custom curves, and this is one of the most common ways of creating them. For example, you can use them to create your own "sigmac", using control points. (I had all my students create their own in numerical analysis.)

• Examples:
  • #11, p. 611
  • #14, p. 611
  • #19, p. 611
  • #22, p. 611
  • #50, p. 612

Links:

• Derivation of Newton's Law of Cooling