- Homework 7.8 returned.
- 3
- 47
- 51
- Want to study in
Belize next summer? I would, but I'm not a student! Please go for
this....
- Please read section 10.4 for next time, on polar coordinates.
- And now for something completely different....
A good introduction to parametric curves is given by ballistics. If we shoot a bullet into the air with speed v horizontally, and we neglect all forces but gravity, then the bullet will trace out a parabola (some bullets are larger than others):
Now how might we characterize the path of the bullet? The answer is a parametric curve, of the form C(t)=(x(t), y(t)).
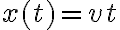
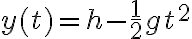
or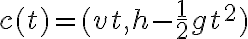 
If we wish, we can solve for t in the equation for x and use that to eliminate the parameter t from the equation for y, hence getting an equation for the parabola traced out: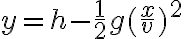 
According to this parameterization, where is the "bullet" at time t=0? In which direction is the motion occuring -- left to right, or right to left? (You have a parametric graphing mode on your calculator -- let's try it out.)
Orbits of planets in the heavens, movements of ants on a hill, a robotic arm in an assembly plant: all these can be described by parametric curves.
- A couple of famous examples involving the cycloid:
- The Brachistochrone Problem (1696, solved by Newton in a single day after he heard the challenge)
- The Tautochrone Problem (1673)
- Parametrizing a line:
- Parametrizing a circle:
- There are two distinctively different kinds of derivatives that
we're going to have to consider here:
- There are the time-derivatives, which give us the actual
speeds of the "particles" as they zip along in time. For the bullet,
the speed will be
- Then there's the standard "derivative to the curve",
dy/dx, which, as we will see in the next section, works out to
- There are the time-derivatives, which give us the actual
speeds of the "particles" as they zip along in time. For the bullet,
the speed will be
- One of the most important applications of parametric curves is in
the Bezier curve lab at the end of 10.2. There are lots of important instances
where we need to be able to design custom curves, and this is one of
the most common ways of creating them. For example, you can use them to
create your own "sigmac", using control points. (I had all my
students create their own in numerical analysis.)
- Examples:
- #21, p. 665
- #14
- #27
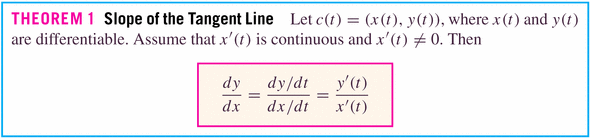
- We start with the the chain rule, and the derivative
dy/dx. We must express this derivative in terms of time, which
is the actual independent variable in parametric curves:
- Tangent lines and Arc Length
-
Suppose a particle has followed the parametric curve
- Examples:
- #1, p. 675
- #3
- #20
- #41
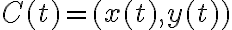: then we
can compute how far the particle has travelled during the
interval 
easily
using the dirt formula,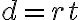 
(in its modified
form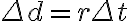 
).
In this case, the rate is just the speed. So we compute the integral
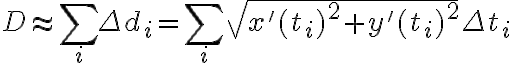
This is actually just a re-expression of the arc length formula:
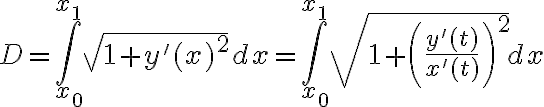
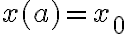and
.
But arc length may be different from the distance the particle travelled: a particle can revisit many sections of the curve y(x) -- so once again we need to be careful to distinguish between the independent variable of interest (whether x or t).
Let's calculate how far a particle travelled when parameterized by
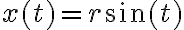and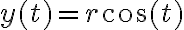 
, with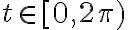 
What happens to our integral if we double the time interval?
- Examples:
If you look over the pictures in this section, you will see that there are many beautiful curves that one can create using polar coordinates and polar plots.
See, for example, p. 685 -- which should remind you of a spirograph, if any of you are that old....
The polar coordinate system was introduced by Newton, an alternative to
the Cartesian coordinate system. In the polar coordinate system every point in
the plane is expressed by its distance and direction (angle) from the origin
(called the pole). The polar axis plays the role formerly played
by the positive x-axis. The polar
coordinates are often given as , where
- r is the distance from the pole, and
-
is the angle between the polar axis and the ray passing through the point of interest.
