- Reminder: turn off your rectangles!
- You do have a homework due today
- I've also given you an assignment for next Tuesday, to prepare yourself for the exam.
- We have our first test next week, through Fibonacci numbers. We'll have a review next time -- come with questions. We'll start by going over your homework -- so submit it at the beginning of class (not accepted afterwards).
- Before we get to our question, however, let's recall a couple of things about Fibonacci numbers:
- They're named after Leonardo
Pisano Fibonacci, of 13th century Italy: they earned his name by
being featured in a story problem about bunnies.
- Start with a new-born pair of "immortal" bunnies (they never die).
- A new-born pair requires a month to mature.
- The following month, the pair produces a new pair, which is subject to the same rules.
- Do it again, do it again, do it again!

- As usual, they were known before Fibonacci, by the Indians prior to Fibonacci's time.
- Fibonacci number decomposition and Fibonacci Nim.
- If you looked at some special cases, what can we conclude?
We concluded that, if the starting number was a Fibonacci number, then it was best to go second (be player 2); if not Fibonacci, try to be player 1.
- What's the winning strategy in Fibonacci Nim?
We concluded that it comes down to this fact:
Every natural number is either
- Fibonacci, or
- can be written as a sum of non-consecutive Fibonacci's in a unique way.
Two things:
- If the number of counters starts as a non-Fibonacci (more common), try to go first. Otherwise, if Fibonacci, go second if you can. Then you're in the driver's seat.
- If at any time you can apply this strategy, you are guaranteed to win:
Write the number of counters as a sum of non-consecutive Fibonacci numbers, and take the smallest in the sum.
- 26
- 34
- 98
- If you looked at some special cases, what can we conclude?
- How Fibonacci numbers are defined: The Fibonacci numbers are given as a recurrence relation
(do it again, do it again, do it again!). - Fibonacci
Numbers and Nature:
- From the link above:
The English puzzlist, Henry E Dudeney (1857 - 1930, pronounced Dude-knee) wrote several excellent books of puzzles (see after this section). In one of them he adapts Fibonacci's Rabbits to cows, making the problem more realistic in the way we observed above. He gets round the problems by noticing that really, it is only the females that are interesting - er - I mean the number of females!
He changes months into years and rabbits into bulls (male) and cows (females) in problem 175 in his book 536 puzzles and Curious Problems (1967, Souvenir press):
If a cow produces its first she-calf at age two years and after that produces another single she-calf every year, how many she-calves are there after 12 years, assuming none die?
This is a better simplification of the problem and quite realistic now.
- dandelions (Here's a scanned dandelion -- a scandelion)
- Spirals in daisies:
- Similar things happen in artichokes, pineapples, etc. I've even got a cactus skeleton that shows Fibonacci spirals....
- Let's look at some pine cones....
Why does it happen? Some folks believe that it's because of the way things grow, suggesting something like this.
Let's revisit Pascal's triangle, and discover Fibonacci numbers therein:
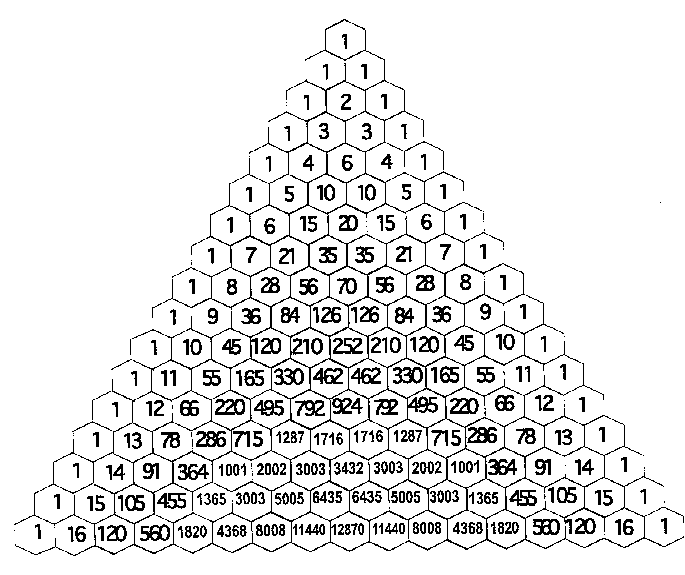
- From the link above:
- As we move toward the so-called "golden mean" and "golden rectangle", we'll start with a nice logarithmic spiral (see this site)
- They're named after Leonardo
Pisano Fibonacci, of 13th century Italy: they earned his name by
being featured in a story problem about bunnies.