Day 36, Mat227

Last time

Next time

Announcements:
- Your Exam 3 is graded.
  - Curve: add 4.5 points to your score.
  - Key (except for 3)
  - Comments:
    1. You all generally thought that the second integral was another integration by parts. It's not. It's by logs....
    2. Some of you didn't like either choice!
    3. The limits were indeterminate, determinate, and indeterminate. The first thing you check before doing L'Hopital's rule is whether the limit is actually indeterminate.
      In fact, the two indeterminate limits do not exist (and the determinate limit was 0).
      Some of you did a first stage of L'Hopital's correctly, then got a determinate limit -- but pushed on anyway!
    4. Many of you seem to have forgotten your properties of logs.

Section 10.1: Parametric Equations

And now for something completely different....

A good introduction to parametric curves is given by ballistics. If we shoot a bullet straight from shoulder height into the air with speed v horizontally, and we neglect all forces but gravity, then the bullet will trace out a parabola (some bullets are larger than others: start this one from the top of the parabola):

Now how might we characterize the path of the bullet? The answer is a parametric curve, of the form C(t)=(x(t), y(t)).

${x(t)=vt}$		${y(t)=h-\frac{1}{2}gt^2}$
or	${c(t)=(vt, h-{\frac{1}{2}gt^2})}$
If we wish, we can solve for t in the equation for x and use that to eliminate the parameter t from the equation for y, hence getting an equation for the parabola traced out:
	${y=h-\frac{1}{2}g(\frac{x}{v})^2}$

According to this parameterization, where is the "bullet" at time t=0? In which direction is the motion occuring -- left to right, or right to left? (You probably have a parametric graphing mode on your calculator -- you might like to try it out.)

Orbits of planets in the heavens, movements of ants on a hill, a robotic arm in an assembly plant: all these can be described by parametric curves.

A couple of famous examples involving the cycloid:
- The Brachistochrone Problem (1696, solved by Newton in a single day after he heard the challenge)
- The Tautochrone Problem (1673)
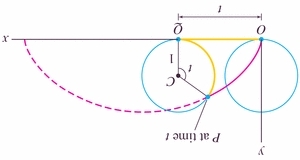
Parametrizing a line:
Parametrizing a circle:
There are two distinctively different kinds of derivatives that we're going to have to consider here:
- There are the time-derivatives, which give us the actual speeds of the "particles" as they zip along in time. For the bullet, the speed will be
  
  ${s(t)=\sqrt{x'(t)^2+y'(t)^2}=\sqrt{v^2+(gt)^2}}$
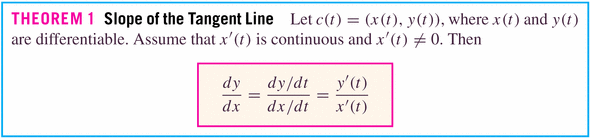
- There is the slope of the tangent line to the curve traced out by the particle, which one can obtain using the time-derivatives:
  
  ${y'(x)=\frac{y'(t)}{x'(t)}=\frac{-gt}{v}=\frac{-gx}{v^2}}$
One of the most important applications of parametric curves is in the Bezier curve section. There are lots of important instances where we need to be able to design custom curves, and this is one of the most common ways of creating them. For example, you can use them to create your own "sigmac", using control points. (I had all my students create their own in numerical analysis.)
Examples:
- #1, p. 665
- #3
- #7
- #15

Website maintained by Andy Long. Comments appreciated.