Day 4, Mat129: Calculus I

• Remember to come to the computer lab on Friday.

• What questions do you have after your encounter with Mathematica?

• Any problems getting it set up, for those who've tried?

• I'll collect your homework problems -- pp. 61--, #38, 44, 46. I'm going to be gentle in grading these -- I just want to see what you did in this first assignment!

• We'll have our first quiz at the end of the hour, at around 3:05.

1. The most important definition in calculus is the derivative (here is the derivative of $f$ at $x$) and it's a limit. This is why limits are so important in calculus:
   $f'(x)=\lim_{h\to 0}{\frac{f(x+h)-f(x)}{h}}$

2. We saw that limits are pretty easy to determine if you have a graph; What's $\lim_{x\to 1}f(x)$?

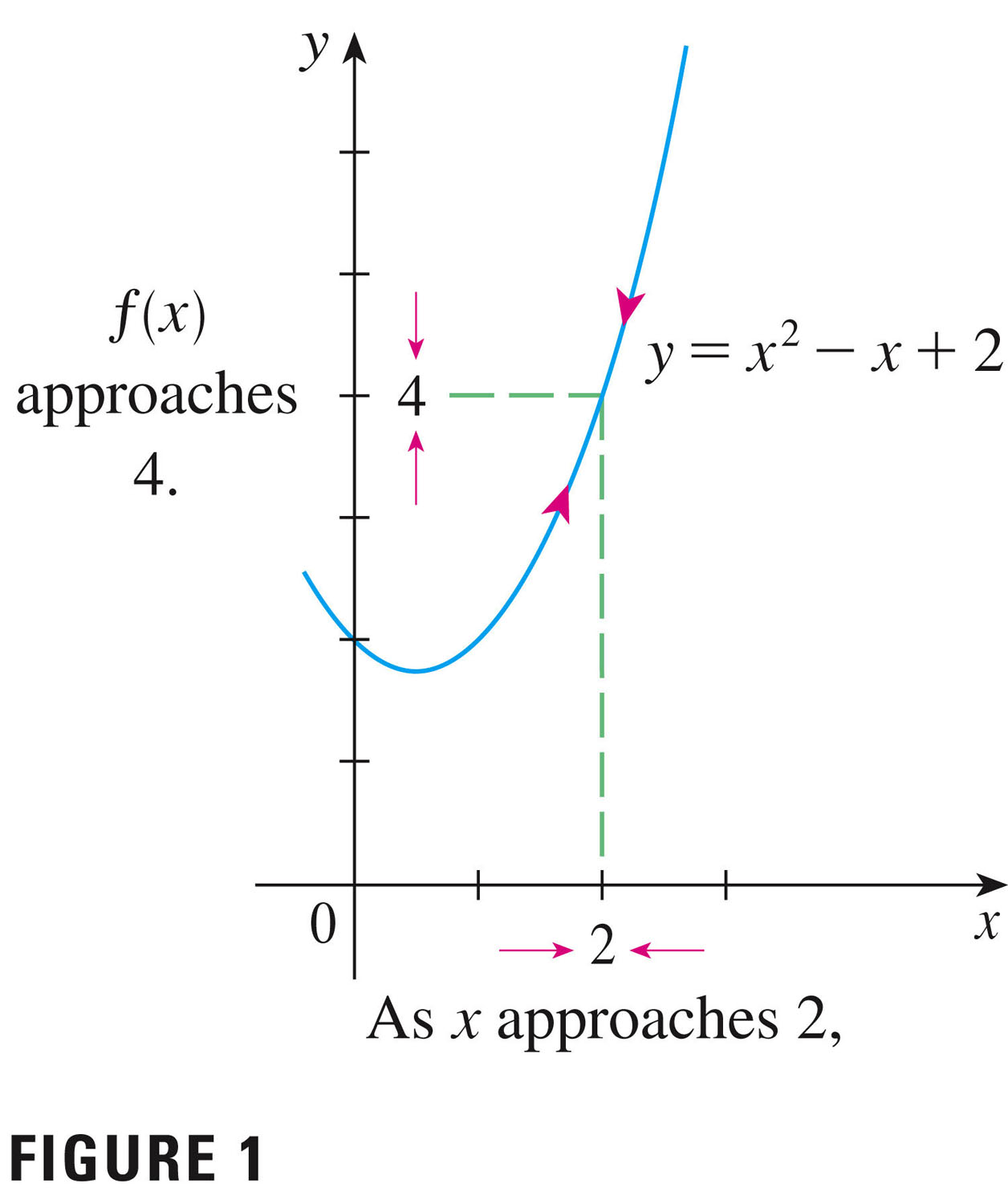
3. We noted that
   • $x$ is independent, and under our control -- we let $x$ approaches value $a$ along the domain (the $x$-axis), from right and from left; then we ask
   • What's $y$ approaching (if anything) along the $y$-axis? $y$ is dependent on what $x$ is doing.

4. We discussed the fact that limits could be infinite,
   • vertical asymptotes representing $y$ heading for $\pm\infty$ as $x$ approaches a finite $a$; and
   • horizontal asymptotes represent $x$ approaching $\pm\infty$ as $y$ approaches a finite $b$.

By the way, the limit is a hungry, hungry operator: it has to eat something -- it's not okay to leave it floating out there alone.

$\lim_{x\to{a}}$

doesn't make any sense: it must eat something:

$\lim_{x\to{a}}f(x)$

or, better yet,

$\lim_{x\to{a}}\left(f(x)\right)$

The limit is gobbling up that $f(x)$!

• Limit laws:
  • We start with two especially trivial special limits:
    • Constant rule $(f(x)=c)$:

      Here the function $f(x)=1$ is plotted. As $x \to 0$, the $y$-values head to 1. Well, more to the point, they never vary from 1! Quite a boring function....

    • Identity rule $(f(x)=x)$:

      Here the function $f(x)=x$ is plotted. As $x \to 0$, the $y$-values head to 0. And the closer $x$ gets to 0, the closer $y$ gets to 0. That's the way it's supposed to work! Also a rather boring function: so predictable!

    What can we do with those? We need more horsepower....

  • Sum law: "the limit of a sum is the sum of the limits"

    So what is this limit:

    

    This sum (and many other operations) satisfy the same pattern:

    "The limit of a STUFF is the STUFF of the limits."

    So, in particular,

    "The limit of a sum is the sum of the limits."
    

  • Difference law
  • Product law
  • Constant multiple law
  • Quotient law (slight caveat here -- no zero denominators)

  • Power law
  • Root law -- again, a caveat: why can't we compute

    \[ \lim_{x\to{0}}\sqrt{x} \]

    Because the function isn't even defined as x approaches 0 from the left (square roots of negative numbers are imaginary). So we have only a one-sided limit, that \[ \lim_{x\to{0^+}}\sqrt{x}=0 \] Of course we can say that \[ \lim_{x\to{1}}\sqrt{x}=1 \] Limits exists everywhere else on the domain of the sqrt function.

  • Putting these laws together
    • Now that we've got the sum and product and constant multiple rules, what can we deduce?
    • "Affine" rule, for one:
      \[ \lim_{x\to{a}}(mx+b)=ma+b \]

    • Then we can proceed to deduce that all polynomials have limits, and furthermore that
      

      This is the conclusion of Exercise #55, p. 71, which says that polynomials are continuous, which we'll consider more in section 1.8.

      Exercise #56 says it's also true for rational functions, for every element of their domain.

  • Take a look at a couple of the examples in the text, examples 5 and 6 on page 66. These both illustrate the limiting process applied to the most important definition in calculus:
    $f'(x)=\lim_{h\to 0}{\frac{f(x+h)-f(x)}{h}}$

    In this case, both show examples of a slightly different version of this most important definition, the derivative at a point $x=a$:

    $f'(a)=\lim_{h\to 0}{\frac{f(a+h)-f(a)}{h}}$

  • Other Laws
    • The squeeze theorem What can we say about the limit of g as x approaches a?

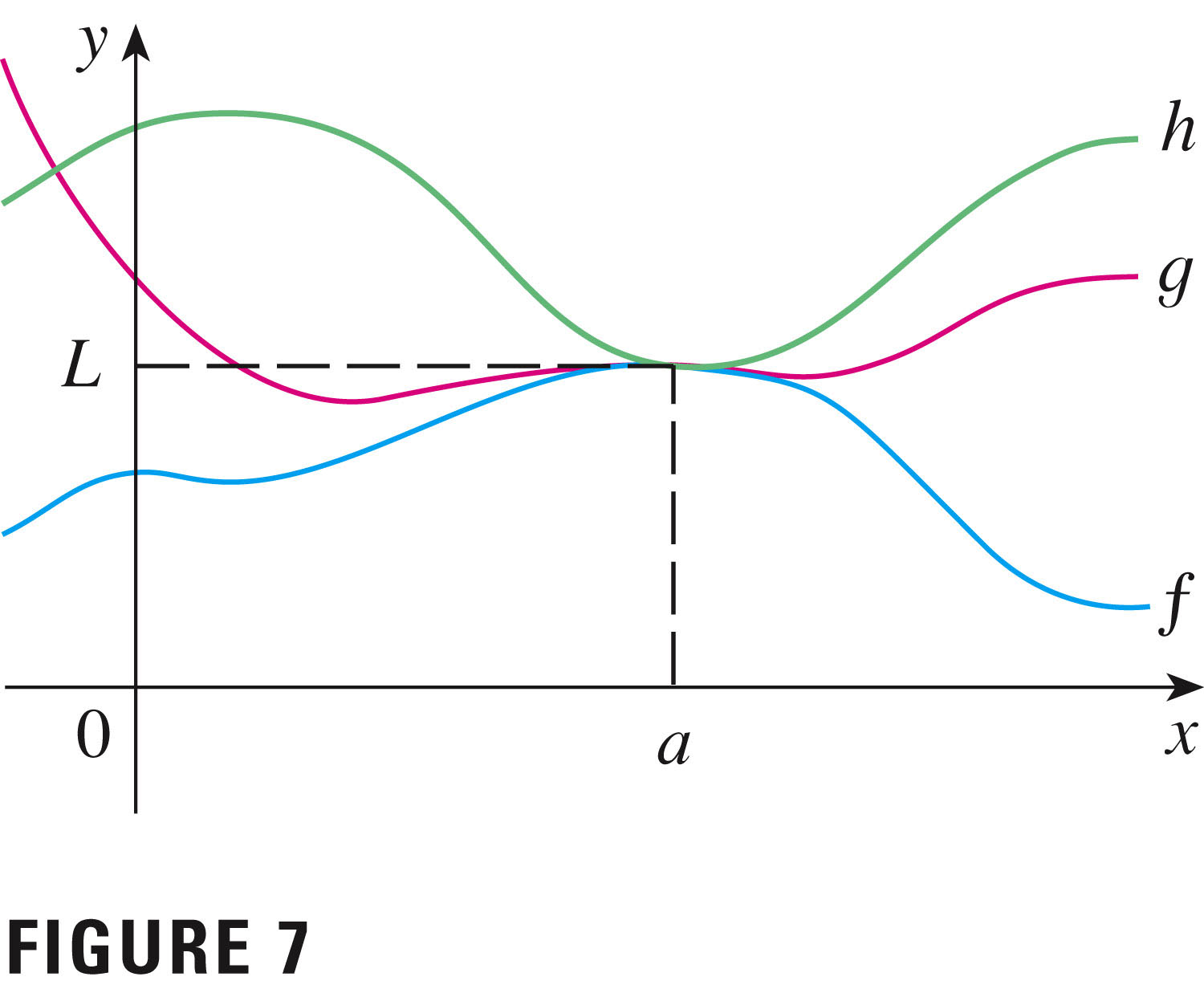
      What's happening to the $y$-values?

  • Now let's take a look at some of the examples from the section exercises.
    • Example 3, p. 65
    • #7, p. 69
    • #10
    • #25
    • #33
    • #47
    • #54

  • Additional examples:
    • #63, p. 71
    • #59, p. 71 (squeeze it!)

• What is the difference between continuous and discontinuous things?
  • A new administration?
  • A sidewalk?
  • Coffee poured into a cup?
  • Global Temperature?

• Definition: continuity at a point

  Note: three things have to happen:

  1. The limit of $f$ exists at $a$,
  2. The function value $f(a)$ exists at $a$, and
  3. The two values are equal.

  Otherwise is discontinuous at

  There are various kinds of discontinuity (which we've already seen):

  • Removable discontinuities (e.g. holes)
  • Jump discontinuities
  • Infinite discontinuities

• Maybe a better definition: a function is continuous on an interval if its graph can be traced there without lifting the pencil from the paper.

• Many of the functions we've considered so far satisfy this, although not all do: consider the "holy function"

  This function has a limit at zero (-.5), but is not defined there.

• Since continuity is wrapped up with limits, we have the same kinds of results that we did in relation to limits:
  • We define one-sided continuity
  • we have sum laws, product, and quotient laws. We've already used these to demonstrate that polynomials are continuous on all real numbers:
    

• Classes of functions that are continuous on their domains:
  • Polynomials
  • Rational functions: (ratios of polynomials)
  • Trigonometric functions: sine, cosine, tangent
  • Root functions: $f(x)=x^{1/n}$
  • Exponential functions: $f(x)=b^x$

• For all these functions one can evaluate limits using the so-called "substitution method": simply plug in for .

• An interesting new function (the greatest integer function) has an infinite number of discontinuities on its domain (Example 10, p. 68). For you computer science types, this function is often called the floor function -- can you see why? How would the ceiling function differ?

• An important theorem (p. 88), RE composite functions (remember that I told you how important compositions are? here's one place they show up....):
  • Roughly: A composition of continuous functions is continuous.

  • Let $F(x)=f(g(x))$ be a composite function.

    If $g$ is continous at $x=c$ and $f$ is continous at $g(c)$ then

    $F(x)$ is continuous at .

• Examples: pp. 90-93, #3, 10, 12, 19, 23, 36, 46 (with Mathematica)

• The Intermediate Value Theorem is an important result of continuity:
  • Theorem: Suppose that f is continuous on the closed interval [a,b] and let N be any number between f(a) and f(b), where $f(a)\ne{f(b)}$. Then there exists a number $c$ in $(a,b)$ such that $f(c)=N$.

  • Example: pp. 93, #69