Day 22, Mat129: Calculus I

• We have a quiz at the end of the hour.
• I'm sorry, I didn't get your homework graded yet. I'll have it for you on Friday.
• You have another one to hand in on Friday (section 3.1). We'll go over that one in class, as you need it for the exam.
• Class this Friday will held in here, rather than in the computer lab. Actually it will be review, because we have an exam on the Monday upon return from spring break.

  Also a reminder that the math/calc lab will be open from 9:00am - 3:00pm that day, for anyone who needs extra help just before the exam.

• The exam will cover up through max/mins, section 3.1. You can email me with questions over spring break, but I may be a little slow to respond.

• We've already seen that derivatives are related to slopes. If the slope is positive (i.e. the derivative is positive) at a point, then the function's graph is increasing there. Similarly, if the derivative is negative at a point, then the function is decreasing there.

  This is the relationship that we explore in this section.

• Similarly the second derivative has much to tell about the shape of a graph.

• Let's work through an example and see how the derivatives are related to the function itself. We'll need a smooth curve....

  Let's look at the graph of #5, p. 220. This is a graph of the derivative (at left):

  

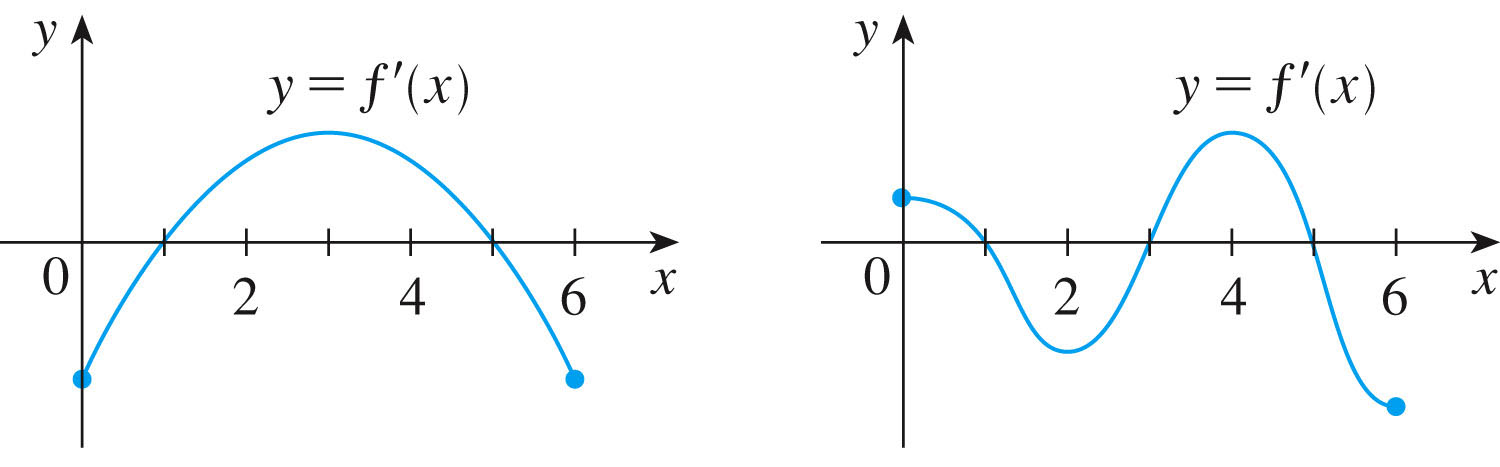
  Questions:

  1. On what intervals is $f$ increasing or decreasing?
  2. At what values of $x$ does $f$ have a local max or min?
  You might notice that the derivative on the left looks quadratic, and hence think to yourself that the function $f$ itself probably looks cubic....

• As we work through the example above, we should see how the first and second derivatives are related to the function itself. So doing, we discover the first and second derivative tests:
  • 1st: When a function is differentiable at a point x=a and has zero derivative (tangent line slope) there, then if the first derivative changes sign from
    • positive to negative, there's a max at x=a;
    • negative to positive, there's a min at x=a;
    • no change in sign, there's neither max nor min at x=a.

  • 2nd: When a function is differentiable at a point x=a and has zero derivative (tangent line slope) there, then if the second derivative is
    • positive at x=a, there's a min at x=a;
    • negative at x=a, there's a max at x=a;
    • zero, we're not sure what's going on....
      • $x^3$
      • $x^4$

  • If you'll just remember the two functions
    • $x^2$
    • $-x^2$
    You'll be able to keep these two rules straight.

    $f'(x)$: - to 0 to +	$f'(x)$: + to 0 to -
    $f''(0)$: +	$f''(0)$: -

• Inflection points:
  1. A graph is concave up if it's "bowlish"; concave down if it's "umbrellaish" (see Figures 5, 6, p. 216)
     

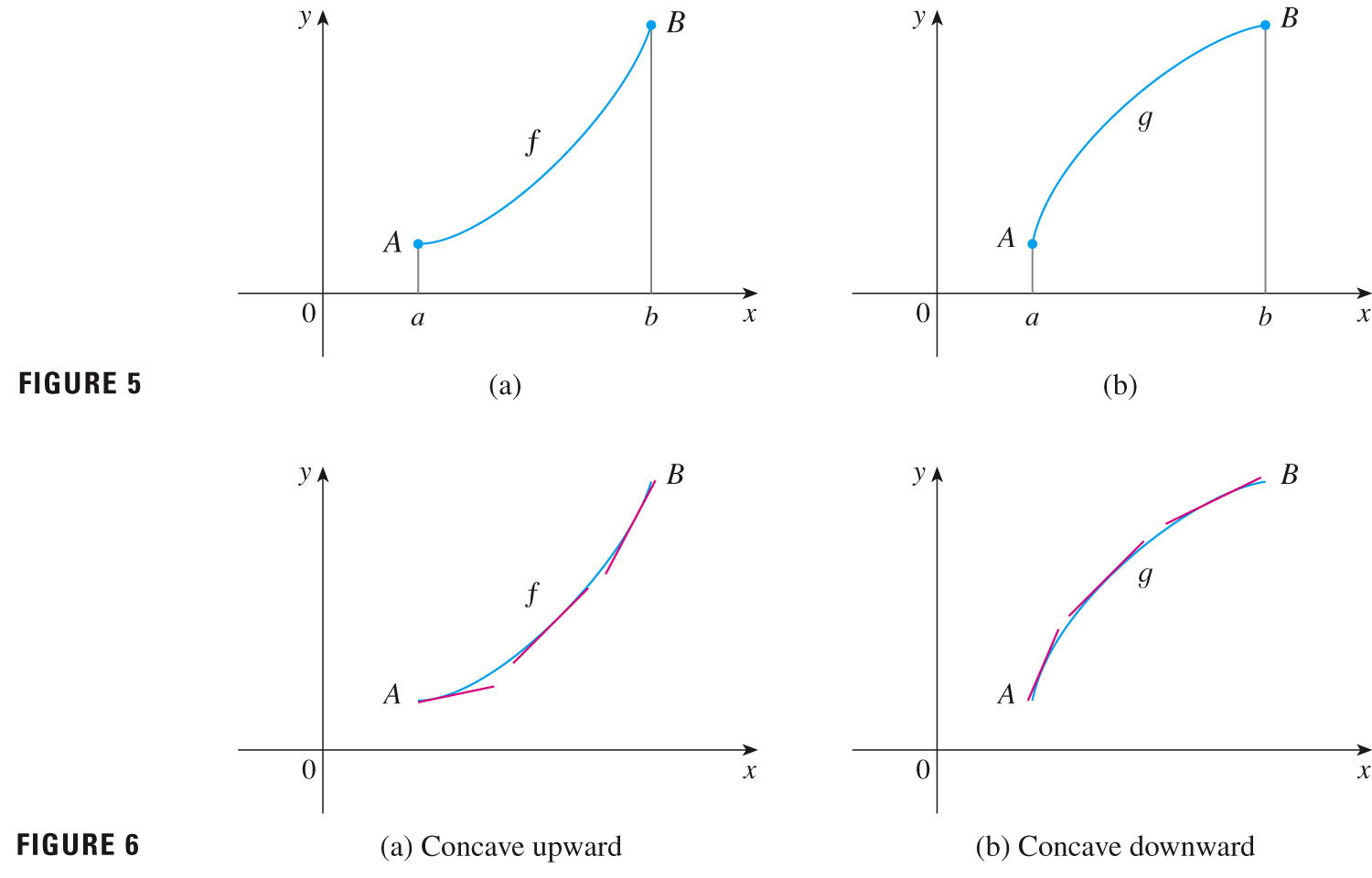
  2. inflection point: Point P on a curve is an inflection point if the curve changes concavity at P.
  3. ``There is a point of inflection at any point where the second derivative changes sign.'' (p. 218)
     

     You might notice that the function itself looks cubic, and hence think to yourself that the derivative probably looks quadratic....

• Examples:
  • #6, p. 220
    

  • #27
    

  • #23
  • #39
  • #50
  • #51

So now we know how to handle closed, bounded intervals; now let's talk about what happens when we let $x$ become unbounded. How will a function behave as $x$ races off to $\infty$ or $-\infty$?

• Examples of functions without finite limits at infinity:
  • non-constant polynomials (go to infinity, in the positive or negative sense)
  • the usual trig functions (while sine and cosine are bounded, but don't settle down to some nice limiting value)

• But rational functions may have horizontal asymptotes, as do exponential functions.

  Another example with a horizontal asymptote is knowledge as a function of time -- #51, p. 222. We might guess that accumulated knowledge in studying for an exam looks something like this:

  

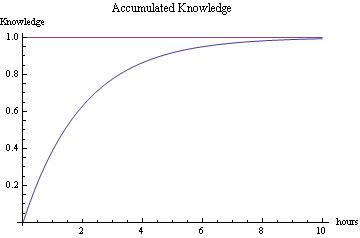
  We might imagine that this physical process becomes less productive from hour to hour as the evening wears on (the law of diminishing returns).

  Other Examples:

  • The hyperbola: $f(x)=\frac{1}{x}$

    More generally, If $r>0$ is a rational number, then

    $\lim_{x \to \infty} \frac{1}{x^r}=0$ If, furthermore, $x^r$ is defined for negative numbers, then $\lim_{x \to -\infty} \frac{1}{x^r}=0$

  • The most general form of an hyperbola is this: $f(x)=\frac{ax+b}{cx+d}$
    • What is its horizontal asymptote?
    • Where is its vertical asymptote?

  • A rational function will have a horizontal asymptote any time that the degree of the denominator equals or exceeds that of the numerator: if
    $r(x)=\frac{p(x)}{q(x)}$

    then if the degree of q exceeds that of p, there is a horizontal asymptote, and the value of the asymptote is given by examining the approximating function given by the ratio of leading terms alone.

  • Interestingly enough, if the degree of the numerator ($n$) exceeds that of the denominator ($m$), then the rational function ultimately looks like a polynomial of degree $n-m$: for example, consider the function $f(x)=\frac{x^2-1}{x}$. Its graph will ultimately look a lot like the function $g(x)=x$ (which one can determine by (gasp!) long division....

    This is called a "slant asymptote" (not a horizontal asymptote, for obvious reasons! If you get far from the origin, then the difference between the two functions falls away.

    We can then replace the more complicated with the simpler.

    We use this idea in physics all the time: we assume that gravity is constant at the surface of the Earth. In fact, it varies as distance to the center of the Earth, but we're so far away that we can take this as a constant (its value at about 4000 miles -- our distance to the center of the Earth). We're far enough from the origin (the center of the Earth) that we treat acceleration due to gravity as locally constant.

  • The usual rules of limits apply, with the caveat that one must be careful when dealing with $\infty$. It is not a number, and cannot be added to anything else (e.g. it is not valid to write that $\infty-\infty=0$ -- for example, $x^2$ and $x$ both go to infinity, but their difference, $x^2-x$, also goes to infinity).

    We can say, however, that $\infty*\infty=\infty$, that $\infty*1=\infty$, that $\infty/1=\infty$, etc. So some of the usual rules apply (and hopefully make sense!).

• Examples:
  • #7, p. 234
  • #11
  • #16
  • #44 (re-express the function first, and use symmetry!)
  • #49 (factor first, and use symmetry!)