- We played a few "games" involving planar graphs and Euler paths (and circuits).
- When we have an Euler path (two odd vertices -- need to start at one, and end at the other).
- When we have an Euler circuit (zero odd vertices -- start and end at the same vertex).
- Euler's formula (for planar graphs): $V-E+F=2$
- Today I emphasized that a graph is planar, no matter how it is drawn. So we can draw $K_4$ (the graph of the tetrahedron) so that it has false intersections, and we can draw it so that it doesn't have false intersections. But, because we can draw it without false intersections, it's planar.
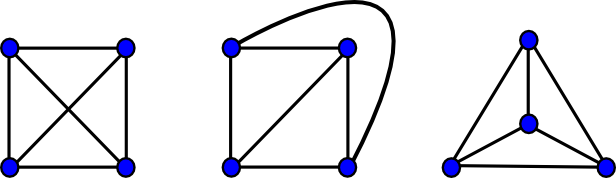
These are all the same graph -- $K_4$ -- the complete graph with four vertices. But in the two cases at right, it's drawn so that you can count the faces: F=4 (don't forget the face on the outside!).
As you can see, $4-6+4=2$.
It's generally easy to count the vertices and edges; in order to count the faces, we have draw it without the false intersections -- then we count.
Today we didn't have answers -- we had conjectures: "theories" about how things worked. Then we would test those conjectures, and see if they held up.
- I began our discussion of these other civilizations with this
shirt from our civilization:

Hopefully you now get the joke. And I'm hoping that we can come up with some more joke shirts, based on these other civilizations and their number systems.
- For those of you who weren't able to be with us today, in person,
I would encourage you to operate this way:
- Examine carefully, for five minutes each, the two
artifacts that we studied today, starting with this Babylonian
tablet:

Prof. Buck says this: "Confronted with an artifact from an ancient culture, one asks several questions:
- What is this and what are its properties?
- What was its original purpose?
- What does this tell me about the culture that produced it?
Your job: to describe the markings, and investigate the patterns on this tablet.
Then there's the Mayan calendar:
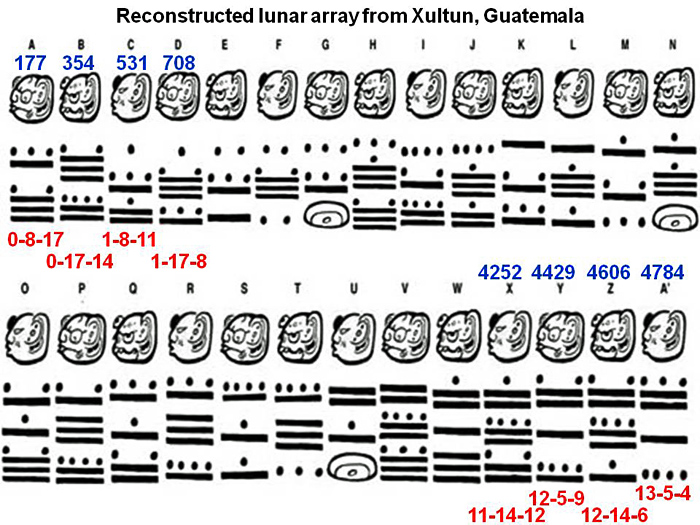
- What the students and I did today was to look for
patterns (which is what mathematicians do), and try to
answer questions like these:
What can we deduce about
- the purpose of the table?
- the way (and reason) Babylonians wrote their numbers the way they did?
- the Babylonians and place value?
- the Babylonian's missing number(s)?
- the Babylonian influence in our world today?
- See if you can solve these mysteries on your own,
before actually listening in on what the
students were saying during class today.
It might be worthwhile listening
in on the classroom discussion, as we consider some
of the conjectures the students made (their hypotheses
as to what's going on).
- Note that after studying the Babylonians, we listened to
the Schoolhouse Rock video -- Zero, My Hero
-- and that was intentional (that it would follow the
Babylonians, and precede the Mayans).
- Students did a great job of finding patterns, and
discovering what is actually going on in the Babylonian
clay tablet, and on the Mayan mural (the calendar was
on the wall of a temple).
- We figured out, together, what the symbols represent, and
we now understand the bases that the two different
civilizations use. Our next step is to actually write
numbers of our own using their systems.
- But there's more work to be done! We'll continue working on these on Friday.
- Examine carefully, for five minutes each, the two
artifacts that we studied today, starting with this Babylonian
tablet: