Chapter 4
Differential Calculus and Its Uses
4.1 Derivatives and Graphs
4.1.5 Completion of the Minimal Cost Calculation
We return to our calculation of the derivative of the weighted surface area function. Here was our progress when we digressed to find the derivative of the inverse function:
Now we continue:
This is the function we graphed in Figure 10, which we repeat here as Figure 11:
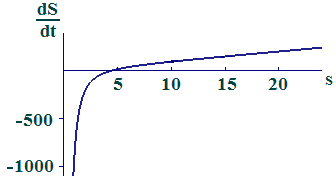 |
| Figure 11 The graph of |
The minimum cost occurs when this derivative is zero:
Solving for s, we find
so inches. The corresponding height is approximately 11.3 inches.
Activity 2
For the calculation just completed, the ratio of the cost of the top and bottom (per unit area) to the cost for the sides (per unit area) was `2.5`. Let's call this ratio `r`. The Orlando Juice Company finds that r has been going up recently.
Find a formula, with `r` as a parameter, for the length `s` that yields the least expensive box.
-
The Sales Department has decreed that `s` must be restricted so that . (Their research shows that customers don't buy boxes with values of `s` outside this range.) If the cost ratio `r` increases to `3.4`, what size box should the company manufacture to minimize cost and stay within the Sales Department restrictions?



