Chapter 17 Summary (Section 17.10)
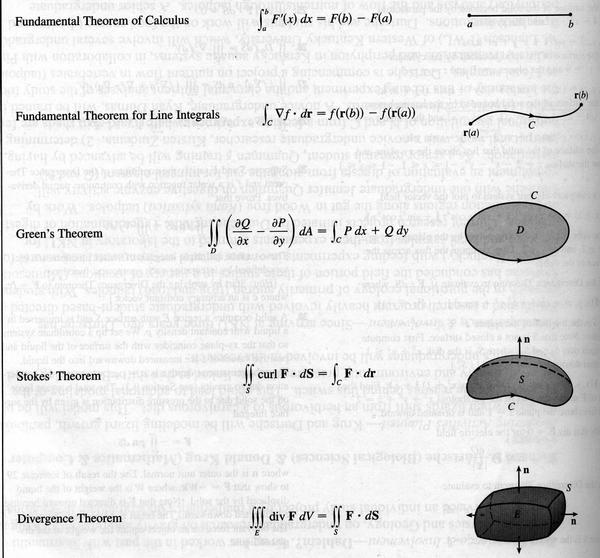
- We start with the fundamental theorem of calculus and generalize it in several different ways.
- The Fundamental theorem of line integrals says as much about gradient
fields as it does anything: they're path independent. It's only the departure
location and destination that count. But in a way such a vector field is "a
derivative" of a (scalar) function, so it does make sense analogously.
- Green's theorem is starting from any old double integral, and says that we
can evaluate double integrals by simply evaluating related functions on the
boundary. In the reverse sense it says that we can evaluate a "work integral"
F .dr by evaluating the curl of F
(which, for a planar vector function, is in the z-direction) dotted with
the oriented surface area (which is just kdA): i.e., it's a special case
of Stokes's theorem!
- Alternatively, we can say that Stokes's theorem generalizes Green's
theorem. Think of
F .dr
as evaluating circulation, and the curl of F is in charge of making
things circulate!
- The divergence theorem states simply that the amount of stuff being
created inside the volume (represented by the divergence) has got to ooze out
from the surface along lines of F, and so will be quantified by the dot
product of F with the oriented surface element dS.
Website maintained by Andy Long.
Comments appreciated.

