A test for time clustering in a single time series or in several time series simultaneously. The empty cells test is based on E, the number of empty ‘cells’ (time intervals) in a sequence of consecutive time intervals. It is sensitive to a temporal clustering of cases such that one or more of the time periods have several cases while other time periods have none.

Data Requirements

Counts of cases within consecutive time periods

may provide cutoff for maximum number of cases with respect to total number of time periods suggested to run test

this method should be used to detect clusters of rare events, so that some of the time periods should reasonably be expected to have 0 cases.

Analysis

H₀: The cases occur at random across the time periods

H_a: Cases cluster in one or more time periods

Test Statistic: The test statistic, E, is the count of the number of cells with 0 cases. When more than one area (time series) is analyzed an overall Chi square statistic tests for time clustering over all the areas simultaneously

Here the i subscript indicates a statistic for the i^th time series, the summations are over the cells/time periods within each series, E is the sum of the number of empty cells, and E(E) and Var(E) are the mean and variance of E under the assumption of a random allocation of disease cases among the cells.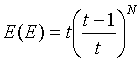

E((E)₂)=(t)₂t^-N(t-2)^N

Var(E)=E(E)(1-E(E)+E((E)₂)

Here t is the number of time cells, N is the total number of cases and (a)_k is a falling factorial. When cases cluster the test statistic will be large, when equal numbers of cases tend to occur in all the cells E will be smaller than its expectation and the test statistic will be small. The significance of E is evaluated using the exact p-value

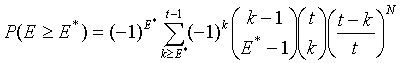
The notation indicates a binomial coefficient. When several time series are tested simultaneously, p-values are combined using the Bonferroni aproach or when at least 20% of the areas have an expected number of empty cells of 5 or more the results can be combined as a continuity-corrected chi-square with one degree of freedom.

Output

E, E(E), and Var(E)

p-value for one-tailed test

Plot of E on its expectation E(E) with 45 degree line through origin

Reference

Jacquez, G.M. 1994, User manual for Stat!: Statistical software for the clustering of health events, BioMedware, Ann Arbor, MI.