- Exam 1 Key is in place
- 9.1 homework due today.
- Homework for 11.1: To be handed in (due 10/14): pp. 543-545, #2, 12, 14, 15, 22, 27, 30, 34, 37, 50, 60, 71
-
Here's our first example of a sequence:
- Definition: A sequence is a function f(n) whose
domain is a subset of the integers. The values
are called the terms of the sequence and n is called the index. For most of our sequences, the domain will be the natural numbers: {1, 2, 3, ....}.
- In the example sequence above, what do you suppose is the limit of
the sequence as
?
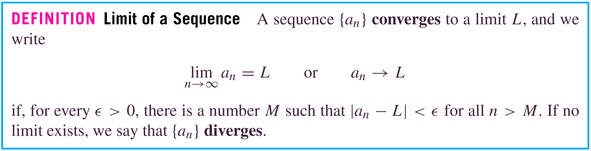
- There are lots of other sequences that we might encounter
naturally: for example, there's one in probability and statistics that
is closely related to the one we just looked at: the poisson
distribution, which has as its probabilities
(what do you notice about the sum of the terms?).
- Other sequences might be defined in terms of a function. For
example,
Examples:
- #4, p. 543
- #11, p. 543
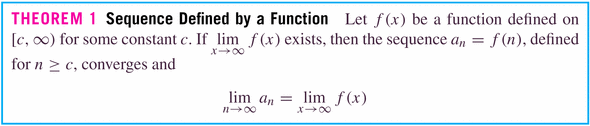
Here's an especially interesting historical limit:
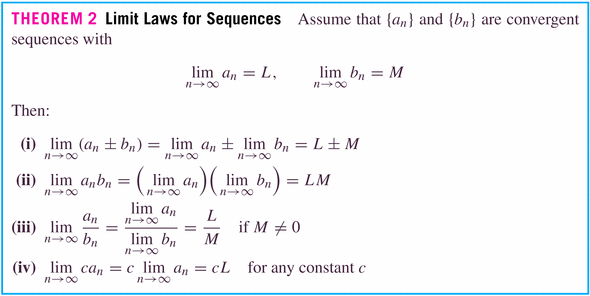
- One piece of good news: the obvious rules for limits are working:
Examples:
- #12, p. 543
- #43, p. 544
- Some vocabulary: bounded sequences....
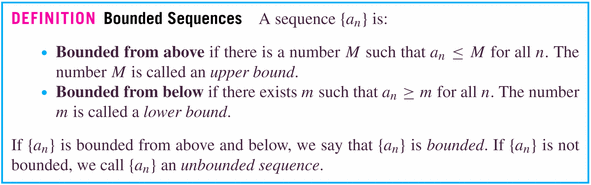
And some theorems related to this notion:
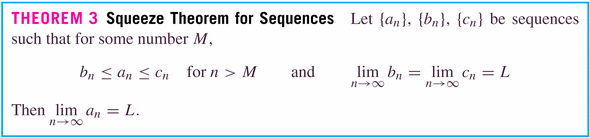
(especially useful for alternating sequences)

(note that the converse is false)
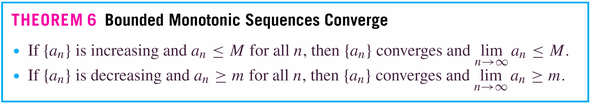
Let's use Theorem 6 to show that the sequence we initially considered is convergent:
- Geometric sequence: a sequence of the form
where r is called the common ratio.
- Examples:
- #19, p. 543
- #28, p. 544
- #43-63, p. 544

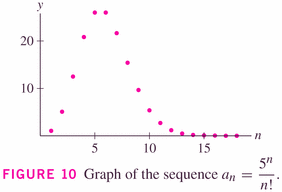
What would we get if we could add up all these (infinite!) terms? 148.4131591025766....
By the way, what's wrong with that figure?