- Sorry if I wasn't available yesterday for some of my office hours. A bit of a medical issue with my son.
- Exam revisions graded: come in to discuss it and get 100% on the
redo. Email me to schedule a meeting (Please use subject line
REDO).
Only 5 out of 14 have contacted me about this! What are the rest of you waiting for?
- Homework 11.4 returned.
- Homework for 11.5 due today.
- Quiz for 11.6 returned.
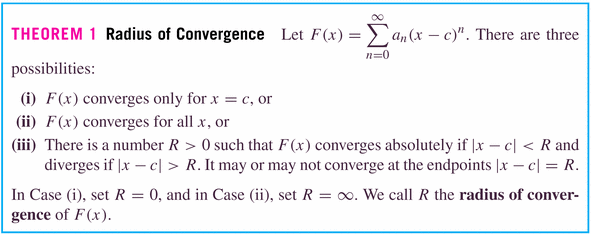
- Now, rather than simply infinite sums of numbers, we're
going to consider an infinite sum of functions in what
are known as power series. They're like
polynomials, but they don't have finite degree.
The terms will be monomials -- constants times powers of x.
We've been sneaking up on these: we started by talking about Taylor series polynomials, which are generalizations of the linearization, or linear approximation (which has the tangent line as its graph).
Then we seemed to switch gears, and began talking about adding up infinite sequences of numbers.
Now we realize that, with functions for terms, each value of x specified gives rise to an infinite series, and we might immediately wonder if the series is convergent.
So what kind of function has an infinite number of terms? How do you evaluate such a thing? What kinds of function are these? It turns out that a lot of our old friends can be expressed this way --
, for example, as we've seen.
Now what did we assert about
? That
For what values of x would this converge?
Apply the ratio test for arbitrary x, and what do you discover?
So
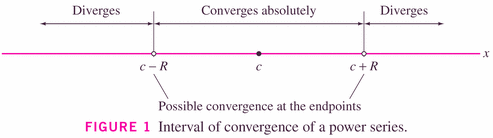
presents us with a case where we have an "infinite radius of convergence".
Let's look at an example where our radius of convergence is not so nice: consider
If we construct the terms of the Taylor series polynomial as we did in section 9.4, via the equation
And then set a=0 (MacLaurin series), and note that all the derivatives evaluated at 0 are n!, then we see that
Now: for what values of x do you think that this will converge? And why does it make sense?
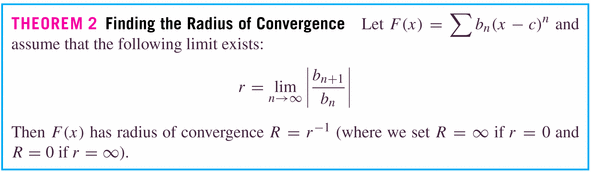
Note that this is a variant on the theme of the ratio test: we don't consider the power terms, but only the coefficients.
- Examples:
- What does Theorem 2 say will be the radius of
convergence of the series for
?
- What about the endpoints?
- #3, p. 585
- #8, p. 585
- #21, p. 585
- What does Theorem 2 say will be the radius of
convergence of the series for
- Examples:
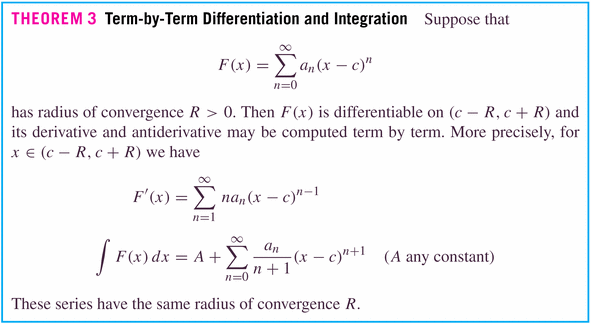
- What do we get when we differentiate the series
for
term-by-term?
- What do you get when you integrate
? How can we find a power series for this function?
- We can use one series to find the power series
expansion for another series. What would be the power series
for
? What would be the power series of its anti-derivative(s)?
- #40, p. 586
- #47, p. 586 (a harder one! But good practice for manipulating power series, and to show why they're useful).
- What do we get when we differentiate the series
for
- We've basically seen all this material before: we're now just
summarizing:
The coefficients akare "designed" so that after k differentiations of the series, when we evaluate the series we pick off only the kth derivative evaluated at x=c:
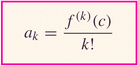

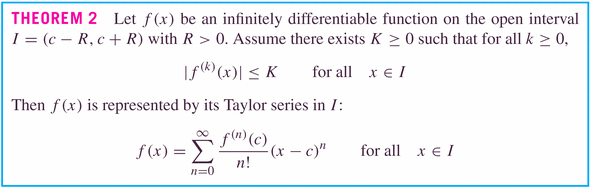
If we can bound all derivatives of a function on an interval about x=c, then the Taylor series represents the function there:
The expansion for the binomial series is a classic: it's another of the great achievements of Sir Isaac Newton, one of the founders of calculus:
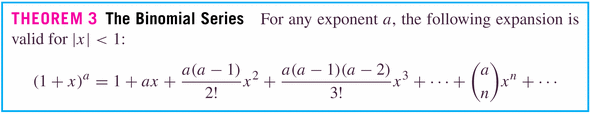
- Examples:
- #1, p. 596
- #4, p. 596 (remember that there are several different ways to do these! You don't have to work from the definition....)
- You choose some....