Day 11 in Mat129

Today:

• Announcements
  • New assignment:

    Thu

    2/4

    Section 3.2/3.3

    Read 3.4 Begin on-line homework assignments 3.3 (due Thursday, 2/11) By hand: pp. 118, #61, 65, 66 (due Tuesday, 2/9)

• Roll

• Section 3.1: One more example:
  • #29, p. 104

• Section 3.2: The Derivative Function:
  • The definition:
    

  • Here's an example of an important function:
    

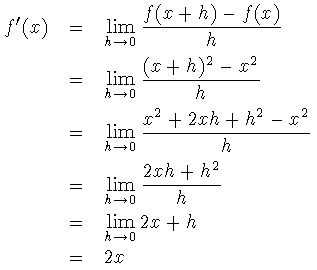
  • A little trickier one: #6, p. 116

  • Derivatives of
    • constant functions
    • the identity function f(x)=x

  • Rules that help us avoid the definition! (with Proofs)

    • The sum rule

      works as we'd hope: the derivative of a sum is the sum of the derivatives

    • The constant multiple rule (makes sense)

    • The power rule (via dominoes -- i.e., mathematical induction)

    • NOTE: at this point we have all the rules necessary to differentiate all polynomials, without needing to resort to the definition!

  • #63 and 64, p. 118

  • Note Theorem 3 (p. 113): differentiability implies continuity (we know that already!)

  • But continuity does not imply differentiability -- examples?

• Section 3.3

  • The product rule

    Rats! "The derivative of the product is not the product of the derivatives."

  • The quotient rule (Rats again!)

• Javamath