- Your homework and quizzes are returned.
- #20, p. 724
- Some of you forgot to raise (-1)^n
- Is the graph of the sequence continuous?
- Yes, the limit is 2 -- but how do you know?
- #32
- Drew
- But how do you find the limit?
- #79
- Franky
- But how do you find the limit?
- #20, p. 724
- You have a homework due tomorrow.
- Your quiz opportunity tomorrow is over 11.5: Alternating Series
- concerns several different means of testing for convergence of
series with all positive terms (obviously, if we're dealing with series
of all negative terms, similar results are going to apply).
- We're not so concerned with the actual limits (that is the values
of the series) as we are with the convergence (or divergence) of the
series.
- Theorems:
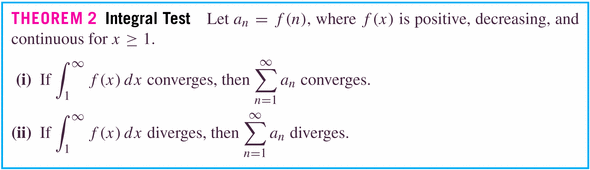
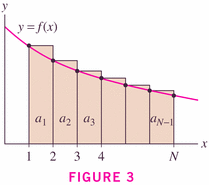
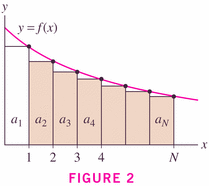
Theorem 3 is just a corollary of Theorem 2, where the integrals are the obvious power functions:
Let
, where f is a positive, decreasing function. If
converges by the integral test, and we define the remainder by
, then
(this gives us a bound on the error we're making in the calculation of a series). This is useful, for example, in the calculation of digits of
(now, you might ask "and what's the use of that?!";).
- Examples:
- Last time we used this method to demonstrate conclusively that the harmonic series is non-convergent.
- Dominoes (here's my page) -- and here's a more elegant page in Mathematica: Divergence of the harmonic series (and dominoes!)
- #22
- #36
- Theorems:
- if the series of the
if finite, so is the smaller series of
;
- if the series of the
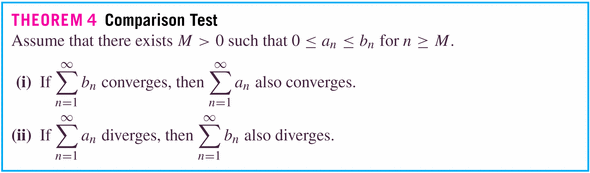
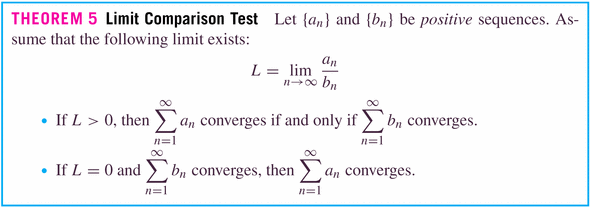
This theorem says that, in the long run, one series has terms which are simply a constant times the terms in the other series.
- if the series of the
- Examples:
- #6, p. 750
- #16
- #28
- #34
- #45
- #46