Day 7: Linear Algebra

Last Time

Next Time

Today:

Announcements:
We'll begin by finishing Section One.II.2: Linear systems. Linear Geometry of n-Space.Vectors in Space
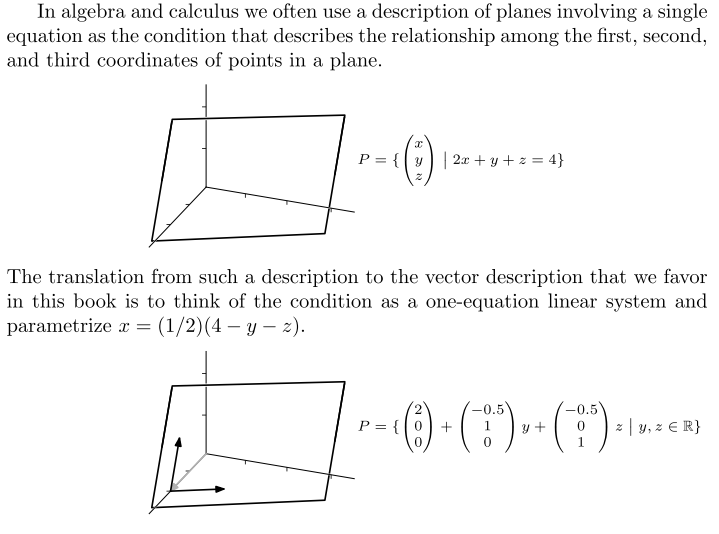
- Let's think about other ways to characterize this plane, perhaps some from your own experience.
- "We finish this subsection by restating our conclusions from the first section in geometric terms. First, the solution set of a linear system with n unknowns is a linear surface in ${\R^n}$ . Specifically, it is a k-dimensional linear surface, where k is the number of free variables in an echelon form version of the system. Second, the solution set of a homogeneous linear system is a linear surface passing through the origin. Finally, we can view the general solution set of any linear system as being the solution set of its associated homogeneous system ofset from the origin by a vector, namely by any particular solution."
- Let's try some problems:
  1. 1.1ac
  2. 1.3
  3. 1.6
Now we turn to Section One.II.2: Linear systems. Linear Geometry of n-Space.Length and Angle Measures
- Definitions of this section: rely on the dot product:
  
  ${\overline{u}\cdot\overline{v}=u_1v_1+u_2v_2+\ldots+u_nv_n}$
  - angle between two vectors
  - orthogonal (perpendicular) vectors
  - length of a vector
  - dot product
    - aka inner product, scalar product
    - Give us a test for when two vectors are perpendicular -- if the dot product is zero.
    - Here's how the angle between two vectors is defined:
      
      ${\overline{u}\cdot\overline{v}=||{\overline{u}}||||{\overline{v}}||\cos(\theta)}$
- Theorems:
  - Triangle Inequality
  - Cauchy-Schwartz Inequality (corollary)
  We are going to prove this result.
- Examples:
  - 2.10c
  - 2.11b
  - 2.13
  - 2.24
  - 2.27

Website maintained by Andy Long. Comments appreciated.