Day 25: Linear Algebra

Last Time

Next Time

Today:

Announcements:
- Reminder: if your test score was 60% or below, I expect you to make an appointment to see me.
- Sorry, I don't have your homework graded yet.
- Your first project is due exactly one week from today.
- New homework assignment (due Monday, 3/19) -- notice the "hurry-up due date":
  1. 2.8, p. 172
  2. 2.9
  3. 2.11
  4. 2.22
  5. 1.19, p. 179
  6. 1.20
  7. 1.23
  8. 1.25
  9. 1.27
Do you have any questions about the material of Chapter II?
In chapter three we're thinking about mappings, and now we're looking at homomorphisms between spaces (rather than isomorphisms).
- Maps Between Spaces.Homomorphisms.Definition
  - Definitions:
    1. homomorphism: A function between vector spaces ${h: V\rightarrow{W}}$ that preserves the operations of addition ${h(\overline{v}_1+\overline{v}_2)=h(\overline{v}_1)+h(\overline{v}_2)$ and scalar multiplication: ${h(r\cdot\overline{v}_1)=r\cdot{h}(\overline{v}_1)$ is a homomorphism or linear map.
    2. linear transformation: A linear map (homomorphism) from a space into itself: ${t:V\rightarrow{V}}$ is a linear transformation.
      An automorphism is an example of a linear transformation.
  - Theorems:
    1. 1.6 Lemma: A homomorphism sends a zero vector to a zero vector.
    2. 1.7 Lemma: Each of these is a necessary and sufficient condition for to be a homomorphism:
      1. ${f(c_1\overline{v}_1+c_2\overline{v}_2)=c_1f(\overline{v}_1)+c_2f(\overline{v}_2)$
      2. ${f(c_1\overline{v}_1+\ldots+c_n\overline{v}_n)=c_1f(\overline{v}_1)+\ldots+c_nf(\overline{v}_n)$
    3. A homomorphism is determined by its action on a basis. That is, if ${\langle\overline{\beta}_1,\ldots,\overline{\beta}_n\rangle}$ is a basis of a vector space V and ${\overline{w}_1,\ldots,\overline{w}_n}$ are (perhaps not distinct) elements of a vector space W, then there exists a homomorphism from V to W sending ${\overline{\beta}_i}$ to ${\overline{w}_i}$ , and that homomorphism is unique.
    4. For vector spaces V and W, the set of linear functions from V to W is itself a vector space, a subspace of the space of all functions from V to W. It is denoted ${L(V,W )}$ .
      This is truly something to think about, to reflect on: we have a new vector space, whose vectors are mappings from one vector space to another. We have to define how to combine mappings (sums and scalar products), but -- Whoa!
  - Examples:
    1. 1.17, p. 179
    2. 1.18
    3. 1.21
- Maps Between Spaces.Homomorphisms.Rangespace and Nullspace
  - Overview:
    In this section we discover that a homomorphism ${h:V\to{W}}$ induces ("generates") two special subspaces: one in the domain space, V, and one in the "codomain" space W.
    The important new space generated in the domain is the space of vectors that get mapped to the zero vector of space W (vectors that are "annihilated" by the homomorphism -- that sounds pretty exciting!).
    The important new space generated in the codomain W is the space of vectors that are images of vectors of space V.
  - Definitions:
    1. rangespace: The rangespace of a homomorphism ${h:V\to{W}}$ is ${\mathcal{R}(h)=\{h(\overline{v})|\overline{v}\in{V}}}$ sometimes denoted ${h(V)}$ . The dimension of the rangespace is the map's rank. (See example 2.5, p. 183.)
    2. nullspace: The nullspace (or kernel) of a linear map ${h:V\to{W}}$ is the inverse image of ${\overline{0}_W}$ : ${\mathcal{N}(h)=\{\overline{v}\in{V}|h(\overline{v})=\overline{0}_W}}$ . The dimension of the nullspace is the map's nullity.
    3. nonsingular: A linear map that is one-to-one is nonsingular.
  - Theorems:
    1. 2.1 Lemma: Under a homomorphism, the image of any subspace of the domain is a subspace of the codomain. In particular, the image of the entire domain space, which is the range of the homomorphism, is a subspace of the codomain. [Hence a homomorphism generates lots of subspace!]
    2. 2.14 Theorem: A linear map's rank plus its nullity equals the dimension of its domain vector space V. [Everything goes somewhere, essentially.]
    3. 2.17 Corollary: The rank of a linear map is less than or equal to the dimension of the domain. Equality holds if and only if the nullity of the map is zero. [In this case the map is one-to-one.]
    4. 2.18 Lemma: Under a linear map, the image of a linearly dependent set is linearly dependent.
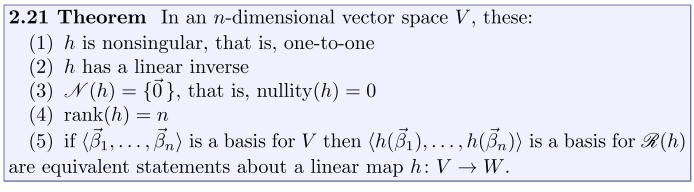
    5. 2.21 Theorem:
  - Examples:
    1. 2.22, p. 190
    2. 2.23
    3. 2.24

Website maintained by Andy Long. Comments appreciated.