- Homework for 16.4 is assigned, due Thursday, 12/3, and is your
last homework to submit.
- Your quiz is returned. Half of you get it, and half of you
don't. Let's talk.
Daniel K. mentioned that gradients point in the direction of greatest increase; he also mentioned Escher:

- Your exams are returned.
- Please evaluate things like "$\sin(\frac{\pi}{2})$", or even "$\sin(\frac{\pi}{4})$".
- Some of you still have issues. If you need to see me to correct those, please do!
- I haven't had a visit from everyone who had a score below
35. Remember that I'll only count your correction if you see
me. I'm holding you hostage! Actually, I'm holding this dog:

Come see me or the dog gets it....
- (some) Mathematica for the exam
Note, in particular, that we can imagine defining a multivariate "Simpson's rule" using the trapezoidal and midpoint methods (univariate: simp=(2mid+trap)/3).
- Please evaluate things like "$\sin(\frac{\pi}{2})$", or even "$\sin(\frac{\pi}{4})$".
- Homework for 16.3 is due today. Let's take another look at #36.
- Green's Theorem:
Let $C$ be a positively oriented, piecewise-smooth, simple closed curve in the plane and let $D$ be the region bounded by $C$ ($C$ is sometimes denoted $\partial D$ in this case, as the boundary of $D$). If $P$ and $Q$ have continuous partial derivatives on an open region that contains $D$, then \[ \oint_{\partial D} Pdx + Qdy = \int_{D}\int_{} \left( \frac{\partial Q}{\partial x} - \frac{\partial P}{\partial y} \right) dA \]
Requiring an open region to contain $D$ means that the derivatives will exist on the boundary. That's why that's included.
- Let's look at the proof in the simple case of a Type I and II
region (#30).
- The reason that this theorem is useful is because sometimes the line
integral is easier, sometimes the area integral is easier -- so we can
choose to make our lives easier! Let's do....
Green's theorem is simply a calculation of a rather special integral on a two-dimensional region $D$: \[ \oint_{\partial D} {\bf{F}} \cdot d{\bf{r}} = \oint_{\partial D} Pdx + Qdy = \int_{D}\int_{} \left( \frac{\partial Q}{\partial x} - \frac{\partial P}{\partial y} \right)dA \] It shows one way to handle the ``work problem'' $\int_{C} {\bf{F}} \cdot d{\bf{r}}$ when the field is not conservative. It can also be seen as a generalization of the Fundamental Theorem of Calculus to area integrals, in the sense that the integral defined on a region can be evaluated by considering only its boundary.
- Green's theorem can be used in a sneaky way to calculate areas with a
line integral: if we find functions $P$ and $Q$ so that
\[
A
=
\int_{D}\int_{}
dA
=
\int_{D}\int_{}
1
dA
=
\int_{D}\int_{}
\left(
\frac{\partial Q}{\partial x} - \frac{\partial P}{\partial y}
\right)
dA
= \oint_{\partial D} Pdx + Qdy
\]
- Examples
- #30, p. 1115
- #1
- Example 3, p. 1113
- #19
- #22
- #23
Today I'd just be happy if we could get a lot (a boat load!) of terminology down. There are also some important concepts, such as cross-products, that you may be unfamiliar with. Curl and divergence rely on two vector products (cross and dot), and an understanding of operators (which may be unfamiliar).
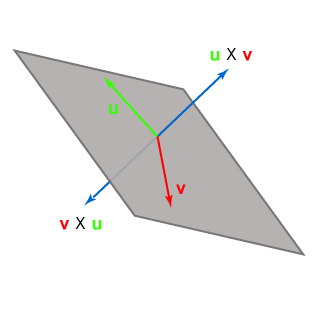
- We'll start with the cross-product:

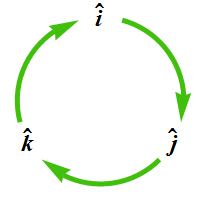

There are a few other properties of the cross-product that you should know:
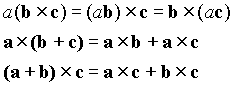
- Let's begin getting serious by introducing the del operator:
\[
\nabla = \frac{\partial}{\partial x} {\bf{i}} +
\frac{\partial}{\partial y} {\bf{j}} +
\frac{\partial}{\partial z} {\bf{k}}
\]
We've seen $\nabla$ before, of course: when it acts on a scalar function $f$,
it returns the gradient of $f$, $ \nabla f$.
There are three ways that we use $ \nabla$:
- as an operator (which is a type of function, which takes functions as arguments, rather than numbers). This is exemplified by the gradient, but also by the Laplacian.
- since we've represented del as a vector, we might use it to
multiply vector functions -- applying both of the two types of
vector multiplication (dot- and cross-products) -- and returning
functions.
- applied with the dot-product, $\nabla$ creates a scalar function;
- applied with the cross-product, $\nabla$ creates a vector function.
- Curl of vector field ${\bf{F}}=\langle P,Q,R \rangle$:
\[
{\textrm{curl}}{\ \bf{F}} =
\left(
\frac{\partial R}{\partial y} - \frac{\partial Q}{\partial z}
\right)
{\bf{i}}
+
\left(
\frac{\partial P}{\partial z} - \frac{\partial R}{\partial x}
\right)
{\bf{j}}
+
\left(
\frac{\partial Q}{\partial x} - \frac{\partial P}{\partial y}
\right)
{\bf{k}}
\]
If curl ${\bf{F}} = 0$ at a point, then ${\bf{F}}$ is said to be
irrotational at that point:
Making use of $\nabla$, then, and the cross-product $\times$, we have
${\textrm{curl}}{\ \bf{F}} =\nabla \times {\bf{F}}$ We can use Green's theorem to try to understand the curl as rotation:
Start by switching to 2D (so F has no $z$-component). Then imagine a contour around a point, say a circle centered at the point, which is shrinking down to nothing. The picture is in your book, on page 1118 (without the shrinking part):

In the limit as the size of the circle shrinks to zero,
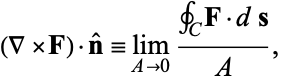
(Think about our quiz.)
Q: What if $\bf{F}$ is conservative, i.e. a gradient field?
If $f$ is a function of three variables that has continuous second-order partial derivatives, then \[ {\textrm{curl}}\ {\bf{F}} = {\textrm{curl}}(\ \nabla f) ={\bf{0}} \] (note that that's a vector ${\bf{0}}$). This says that if ${\bf{F}}$ is conservative, then curl ${\bf{F}} = {\bf{0}}$.
The proof is by Clairaut's theorem (generalized to 3D). Recall: Suppose $f$ is defined on a disk $D$ that contains the point $(a,b)$. If the functions $\frac{\partial^2 f}{\partial x \partial y}$ and $\frac{\partial^2 f}{\partial y \partial x}$ are both continuous on $D$, then \[ \frac{\partial^2 f}{\partial x \partial y} = \frac{\partial^2 f}{\partial y \partial x} \]
Theorem 4, p. 1117: If ${\bf{F}}$ is a vector field defined on all of $\Re^3$ whose component functions have continuous partial derivatives and ${\textrm{curl}}{\ \bf{F}} = {\bf{0}}$, then ${\bf{F}}$ is a conservative vector field.
- Divergence:
\[
{\textrm{div}\ \bf{F}} =
\frac{\partial P}{\partial x} +
\frac{\partial Q}{\partial y} +
\frac{\partial R}{\partial z}
= \nabla \cdot {\bf{F}}
\]
Note that this is a scalar function, which measures the tendency of the vectors of $\bf{F}$ to flow out of (or into) a point.
If ${\textrm{div}\ \bf{F}} = 0$, then ${\bf{F}}$ is said to be incompressible.
Examples:
- #7, p. 1121
- #9
- The Laplacian operator is designated by $\nabla^2$, and creates a
scalar function from a scalar function. We can think of this as the dot
product -- a scalar -- of two vectors:
\[
\nabla^2 f = \nabla \cdot \nabla f.
\]
- Examples: now that we've got all the machinary in place, let's
look at a couple of examples:
- #12, p. 1121
- #9
- Maxwell's
equations should be the poster child for this section.
From the 11/22/2015 edition of the Guardian: Maxwell's equations: 150 years of light: A century and a half ago, James Clerk Maxwell submitted a long paper to the Royal Society containing his famous equations. Inspired by Michael Faraday's experiments and insights, the equations unified electricity, magnetism and optics. Their far-reaching consequences for our civilisation, and our universe, are still being explored.
Light, electricity, and magnetism are brought together in these equations of physics.