Day 19, Mat128: Calculus A

• Rules that help us avoid having to use the definition each time (with Proofs):
  • The constant multiple rule (makes sense)
    $(cf(x))'=cf'(x)$

  • The sum rule

    works as we'd hope: "The derivative of a sum is the sum of the derivatives."

    $(f(x) + g(x))'=f'(x)+g'(x)$

  • The power rule (via dominoes -- i.e., mathematical induction -- or via the binomial theorem).

    The binomial theorem is another algebra problem for folks, and provides another way to pull a rabbit out of my ... hat!

    $(x^n)'=n x^{n-1}$

  • NOTE: at this point we have all the rules necessary to differentiate all polynomials, without needing to resort to the definition!

    The derivative of the monomial $x^n$ is $nx^{n-1}$, and

    The derivative of the monomial $c x^n$ is $nc x^{n-1}$ (by constant multiple).

    A polynomial is just a sum of these. So we apply the sum rule, and the power rule, and the constant multiple rule to the flight of the eraser, to get

    $s'(t)=(at^2+bt+c)'=2at+b$

    and

    $s''(t)=(2at+b)'=2a$

• Other rules:
  • The product rule (doesn't work the way we'd hope):
    $(f(x) \cdot g(x))'=f'(x)g(x)+f(x)g'(x)$

  • The quotient rule:
    low d high minus high d low,
    over the denominator squared they go.

    $\left(\frac{f(x)}{g(x)}\right)'=\frac{f'(x)g(x)-f(x)g'(x)}{g(x)^2}$

• #13, p. 136
• #22, p. 136 (expand first; then try the product rule)
• #48, p. 137
• #58, p. 137

• Radian measure: there is a linear relationship between radians and degrees: $Radians=\frac{2\pi}{360}Degrees$. In calculus, we almost always use radians. You should try to start thinking in terms of radians, too.
• Length of a sector, related to angle $\theta$ subtending and radius $r$:

  Arc Length: $L = \theta r$

  Again, this is an example of a linear relationship: $L$ is proportional to each of $\theta$ and $r$. So

  • if you double the radius, you double the arc length;
  • if you double the angle, you double the arc length.
  

• Definitions of the trig functions, as functions of $x$, in radians (which is how we think about them in calculus):

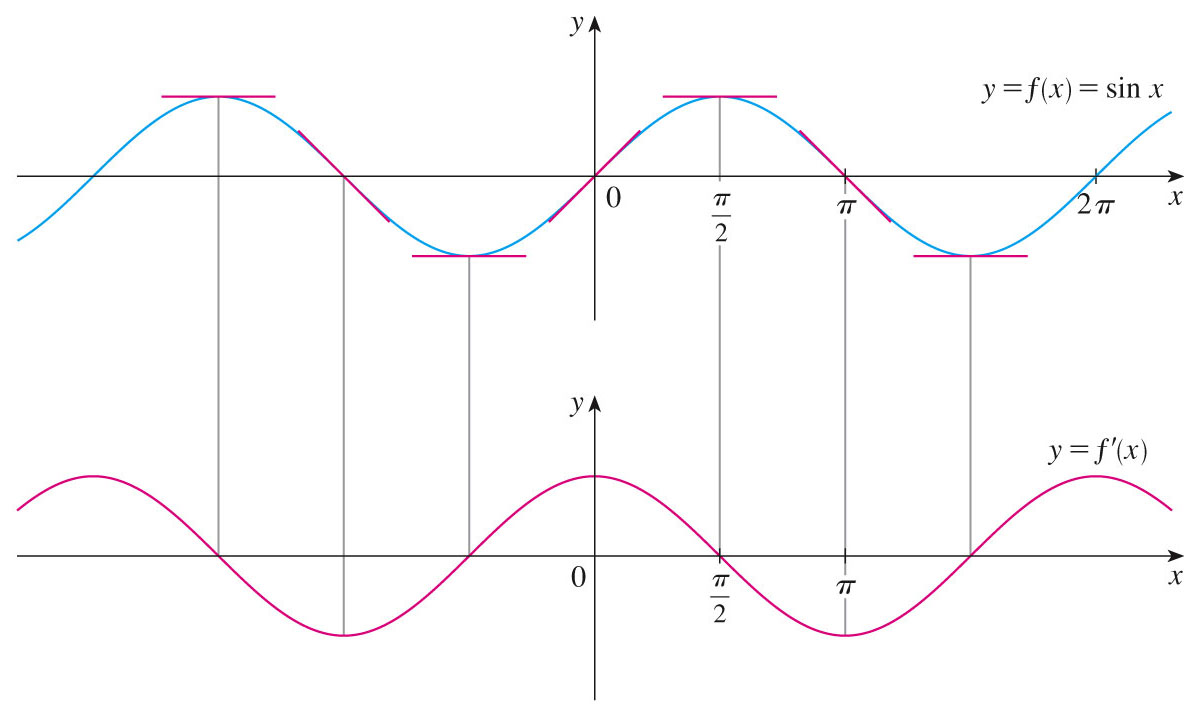
We will derive the derivative of the sine function from the limit definition of the derivative (although we can see the derivative graphically above).

There are exactly three important trig identities one needs to know (all the others can be derived from these three):

• The Pythagorean theorem:
  $\sin^2(x)+\cos^2(x)=1$

• The sine of a sum:
  $\sin(a+b)=\sin(a)\cos(b)+\sin(b)\cos(a)$

• The cosine of a sum:
  $\cos(a+b)=\cos(a)\cos(b)-\sin(a)\sin(b)$

So let's see how to derive the derivative of the sine from the MIDIC -- the limit definition of the derivative -- using the second of these identities.

Then the derivative of the cosine can be derived by simply shifting the sine function, and using the second trig identity above.

• Derivative of tangent (use the quotient rule, of course!)
• Fourth derivatives of sine, cosine
• #7, p. 146
• #26
• #35
• #49