- Your 2.2 homework is returned: graded 10, 42, 55.
- #10 looks like a quartic (although you may not have noticed that).
- #42: d, c, b, a, from $f$ to $f^{(3)}$. You can tell by
the drop in degree, using symmetry, lining up of zeros,
etc. Lots of clues!
By the way, some of you saw two parabolas -- there can only be one (if there are any at all). Think about the derivatives of a quadratic....
- #55 is tough. It involves a bit of subtlety.
- Your 2.3 homework is due.
- There is a quiz at the end of this hour.
-
examples from 2.3:
- #13, p. 136
- #22
- #48, p. 137
- #58
- #64
- Any questions on section 2.3?
- The derivatives of trig functions rely on some basic understanding
of the circle. Indeed, these functions are called "circular
functions" for a reason!
- Radian measure: there is a linear relationship between radians and degrees:
$Radians=\frac{2\pi}{360}Degrees$. In calculus, we almost always use radians. You should try to start thinking in terms of radians, too.
- Length of a sector, related to angle $\theta$ subtending
and radius $r$:
Arc Length: $L = \theta r$
Again, this is an example of a linear relationship: $L$ is proportional to each of $\theta$ and $r$. So
- if you double the radius, you double the arc length;
- if you double the angle, you double the arc length.
- The following diagram is used to prove an important limit
on p. 142:
$\lim_{\theta\rightarrow 0}\frac{\sin\theta}{\theta}=1$
We'll need that limit and some trig identities to compute the derivative of the sine function.

- Definitions of the trig functions, as functions of $x$, in radians (which is how we think about them in calculus):
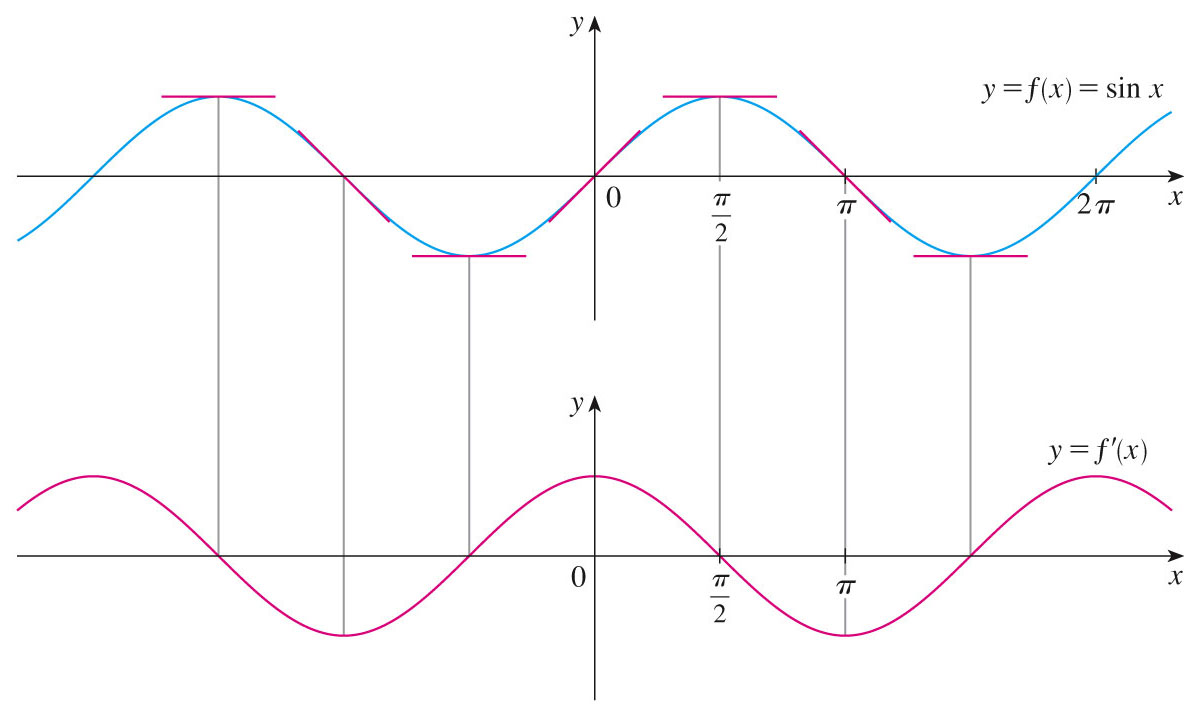
We will derive the derivative of the sine function from the limit definition of the derivative (although we can see the derivative graphically above).
There are exactly three important trig identities one needs to know (all the others can be derived from these three):
- The Pythagorean theorem:
$\sin^2(x)+\cos^2(x)=1$ - The sine of a sum:
$\sin(a+b)=\sin(a)\cos(b)+\sin(b)\cos(a)$ - The cosine of a sum:
$\cos(a+b)=\cos(a)\cos(b)-\sin(a)\sin(b)$
So let's see how to derive the derivative of the sine from the MIDIC -- the limit definition of the derivative -- using the second of these identities.
Then the derivative of the cosine can be derived by simply shifting the sine function, and using the second trig identity above.
- Radian measure: there is a linear relationship between radians and degrees:
- Examples:
- Derivative of tangent (use the quotient rule, of course!)
- Fourth derivatives of sine, cosine
- #7, p. 146
- #26
- #35
- #49