Day 32, Mat129: Calculus I

• Draw pictures!
• #23: Calculus allows us to prove that the square is the best shaped rectangle inscribed in a circle to maximize area.

• First of all, we can't expect our function to be positive all the time, so we define "signed area":
  

  and then we give a name to this area:

  

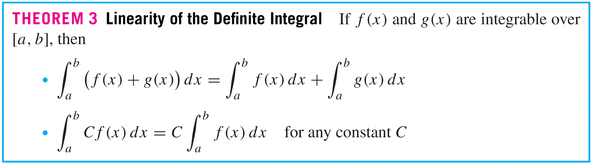
• The area can be calculated by chopping the interval [a,b] up into smaller and smaller chunks, and then using rectangular approximations to the small areas.

  If the size of the intervals goes to zero, then the approximations get better and better, until they're perfect!

  Assuming that we use equal-sized subintervals, the definite integral of $f$ from $a$ to $b$ is defined by \[ \int_{a}^{b}f(x)dx = \lim_{n \to \infty}\sum_{i=1}^{n}f(x_i^*)\Delta x \] where the $x_i^*$ are sample points, one from each of the $n$ rectangles of width $\Delta x = \frac{b-a}{n}$.

  

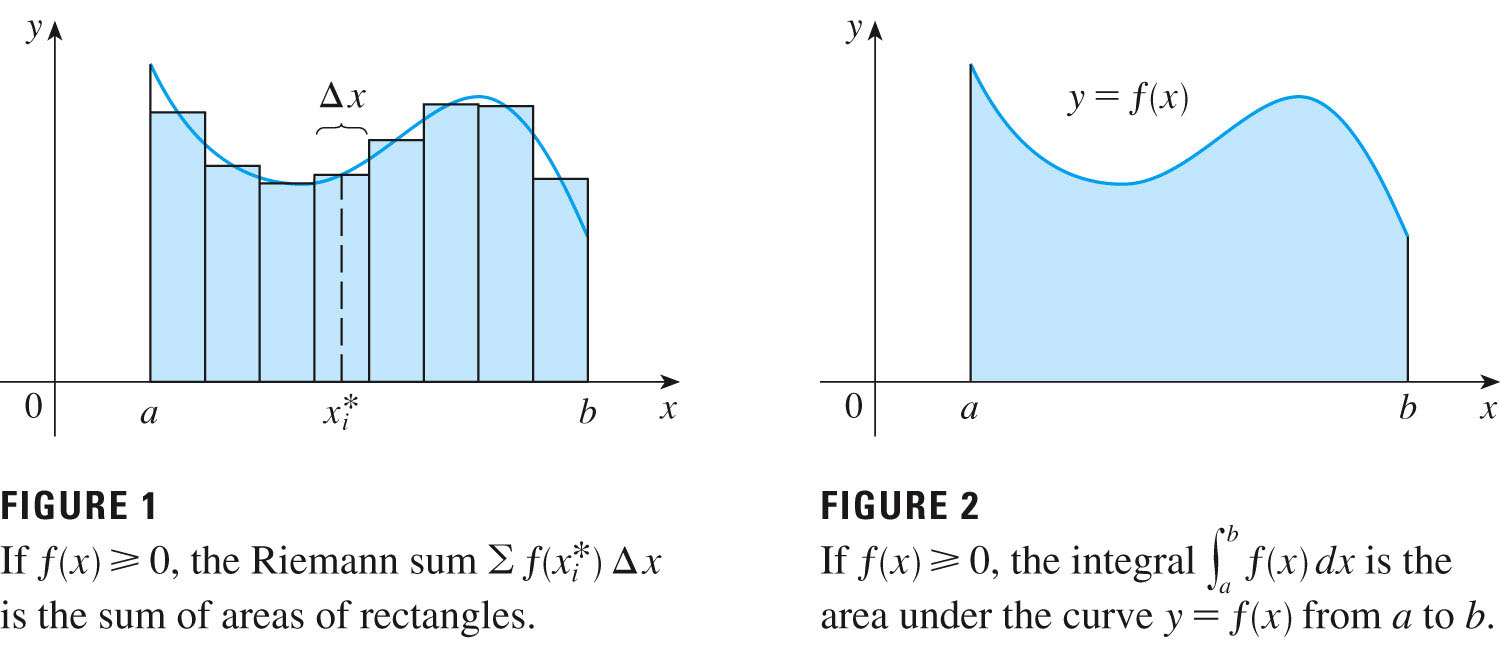
  Theorem 3, p. 297, tells us that this integral will be defined ($f$ is integrable) whenever $f$ is continuous (or has at most a finite set of discontinuities).

  Theorem 4, p. 298, tells us that we can take RRR as our method and be assured of getting the right answer. But we could just as well use the LRR, or midpoint, or trapezoidal, etc.

• Properties of the Definite Integral:
  

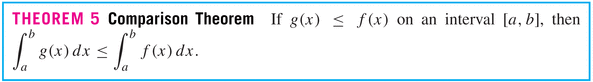
• Here's how we'd rewrite our generalization that Area under the graph of f from a to b =
  width of the interval times the average value =
  $(b-a)\bar{f}$
  where by
  $\bar{f}\mbox{}$
  we mean an average value of f. So
  $\int_{a}^{b}f(x)dx=\bar{f}(b-a)$
  

• Examples:
  • #7, p. 306
  • #10
  • #21 (see p. 298 for the equations you might need, and example 2)
  • #33

1. If $f$ is continuous on $[a,b]$, then

   $\int_{a}^{b}f(x)dx=F(b)-F(a)$ where $F$ is any antiderivative of $f$; that is, a function such that $F'=f$.

2. If $f$ is continuous on $[a,b]$, then the function $F$ defined by \[ F(x)=\int_{a}^{x}f(t)dt \;\;\;\;\; a \le x \le b \] is continuous on $[a,b]$ and differentiable on $(a,b)$, and $F'(x)=f(x)$.

• #3, p. 318
• #8
• #13 (a composition, requiring the chain rule)
• #22
• #23
• #58

where $F(x)$ is any particular anti-derivative.

represent?