Day 8, Mat129: Calculus I

These are tangent lines (places where a line osculates a curve):

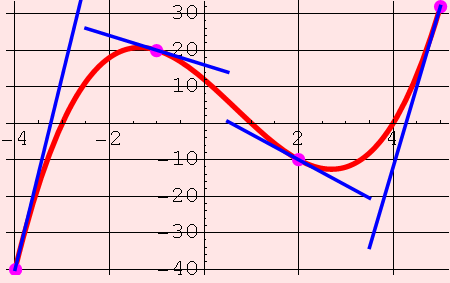
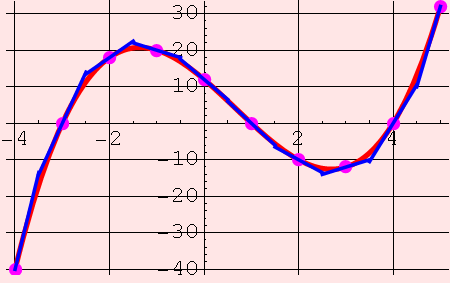
And the slopes of these tangent lines are instantaneous "rates of change" of the function f at the point of tangency. What does that mean?

The thing that tells you how fast a function is changing is its slope, isn't it?

We often represent change in mathematics by a "delta", $\Delta$. So the slope is a change in $y=f(x)$, which we might call $\Delta y$, divided by a change in $x$, or $\Delta x$: \[ slope=\frac{\Delta y}{\Delta x}=\frac{rise}{run} \] So this quantity tells how how fast $y$ is changing given a change in $x$.

• If a function is constant, then it's not changing at all ($\Delta y = 0$).
• If the slope is steep (either up or down), then the function's values are changing dramatically and quickly ($\Delta y$ large).

The rate of change is dictated by the slope. So it should come as no surprise that the derivative of a function, which is the rate of change of a function at a point, is the same as the slope of the tangent line at a point:

We can approximate tangent lines with secant lines:

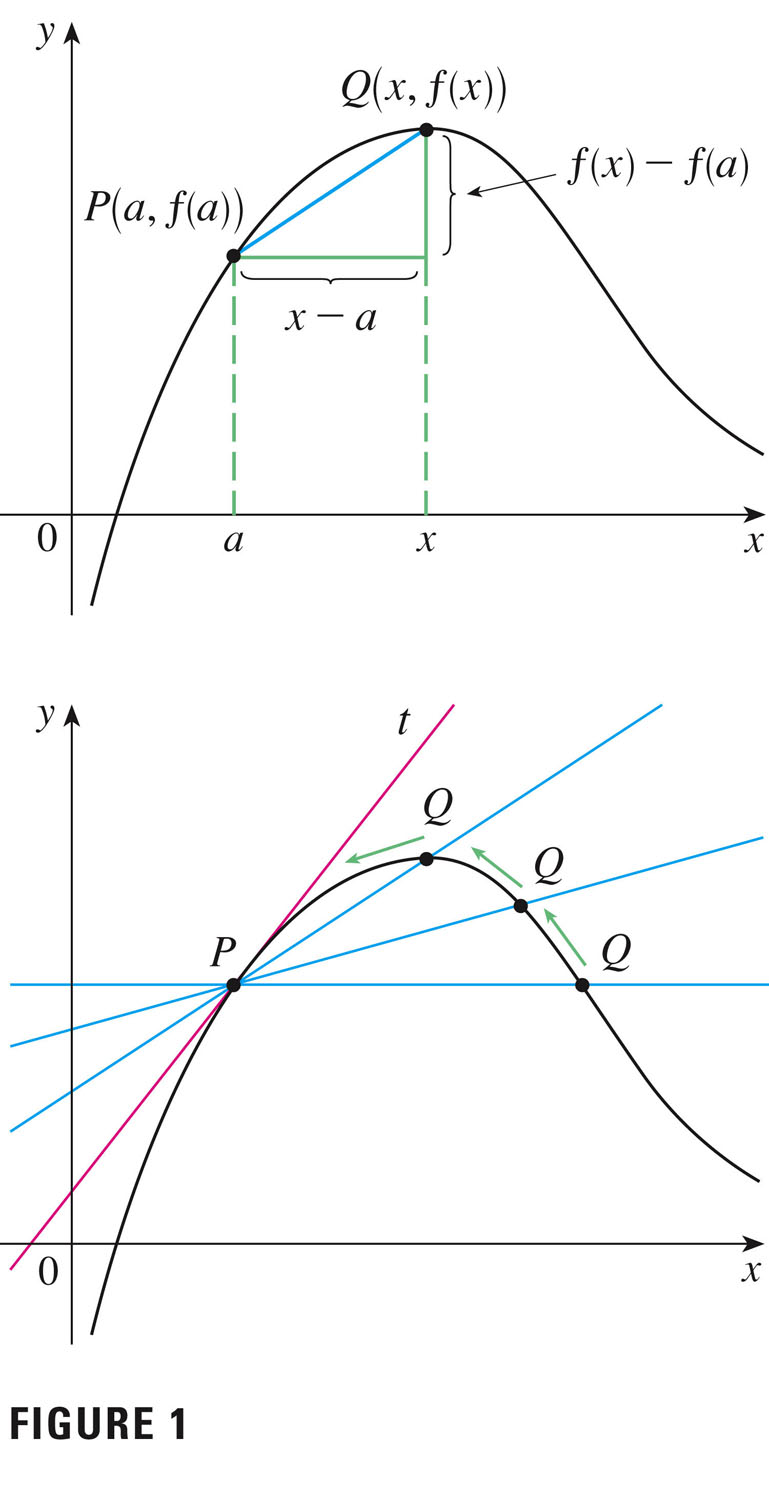
The slope m of the tangent line at P(a,f(a)) is approximated by the slope of the blue line segment (the slope of a secant line), $\frac{f(x)-f(a)}{x-a}=\frac{\Delta y}{\Delta x}$ This is an average rate of change in f over a finite interval. In the limit, this average rate of change becomes an instantaneous rate of change: $m=\lim\limits_{x\to{a}}\frac{f(x)-f(a)}{x-a}$

Here's an alternative notation for the slope: The slope m of the tangent line at P(a,f(a)) is approximated by the slope of the blue line segment, $\frac{f(a+h)-f(a)}{h}$ In the limit, this is $m=\lim\limits_{h\to 0}\frac{f(a+h)-f(a)}{h}$ which I call the most important definition in calculus. This is the formula for the derivative at a point: I've already shared with you this definition of the derivative, at any value of $x$.

$f^\prime(a) = \lim\limits_{h \to 0} \frac{f(a+h)-f(a)}{h} = \lim\limits_{x \to a} \frac{f(x)-f(a)}{x-a}$

The most important definition in calculus! (I just can't say it enough!)

Now let's look at some problems, and see how this concept is connected to real-world problems.

First, however, a word on the examples in this section: you see that, for the most part, we're using the definition(s) of a derivative to compute a slope of a tangent line. The tricky part is often writing the composition correctly -- i.e. just properly using the definition. Then you have to use a little algebra to remove the indeterminacy.

• #3, pp. 110, etc.
• #11, pp. 111
• #13, pp. 111
• #29, pp. 112
• #45, pp. 112
• #51, pp. 113

• If $f$ is differentiable at $a$, then $f$ is continuous at $a$.
• But the converse is not true: just because $f$ is continuous at $a$ does not mean that it is differentiable there.
  

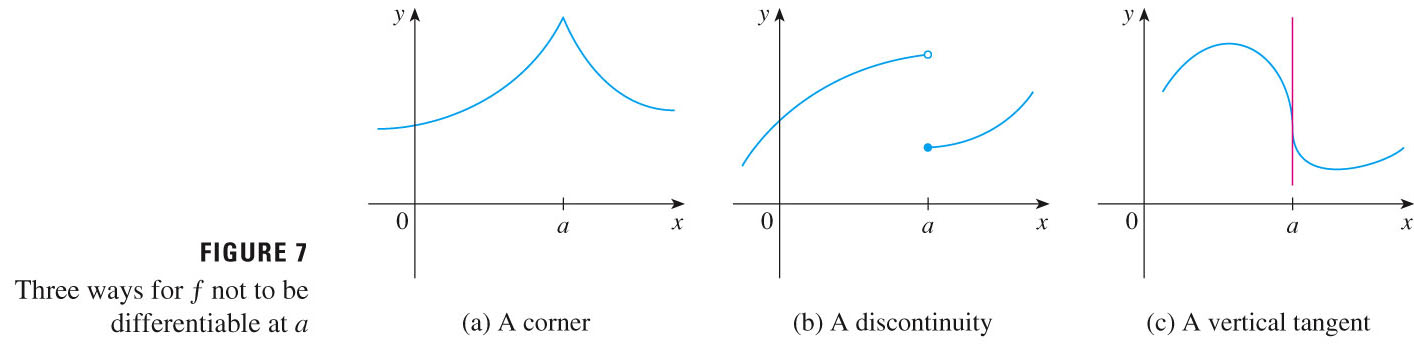
• Differentiability on an interval implies continuity and smoothness.

Let's use the definition of the derivative function,

\[ f^\prime(x) = \lim_{h \to 0} \frac{f(x+h)-f(x)}{h} \]

to determine the derivative functions of these important functions:

1. Constant functions: $f(x)=c$
2. Linear functions: $f(x)=mx+b$
3. Quadratic functions: $f(x)=ax^2+bx+c$

4. An important application of the tangent line

The first two derivatives are easy: each function has a straight line graph, so the tangent line is the graph of the function itself. The slope of each of those graphs is easy to see (either 0 or m). The derivatives are hence on full display. Not so much for the quadratic, and it's the first function that has an explicit dependence on $x$.

How well can you recognize derivatives and the functions that give rise to them? Let's try #3, p. 122.

• What causes breaks in derivative functions?
• How is symmetry preserved in derivatives?
• How does the degree of a polynomial change under differentiation?

Let's think of a quadratic motion, e.g. the motion of an eraser thrown across the room. Let $s(t)$ be the height of the eraser at time $t$:

\[s(t)=at^2+bt+c\]

Each of the coefficients has an important, intuitive role to play:

• $c$ is the initial height, at time $t=0$;
• $b$ is the initial velocity, the rate of change of height at time $t=0$;
• $2a$ is the acceleration (due to gravity) of the height (the derivative of the velocity is acceleration).
  • It is constant across this motion, if we neglect other forces (e.g. air resistance), or the fact that gravity is actually a function of the distance from the center of the Earth.
  • Forces result in accelerations (and vice versa, per Newton's equation $f=ma$).

Think about the parabola which is this function's graph: it's an umbrella. The ball will rise, reach its apex, then fall back to Earth.

The parentheses are supposed to tip you off to the fact that these aren't powers, but rather derivatives.

\[ f^\prime(x) = \frac{dy}{dx} = \frac{df}{dx} = \frac{d}{dx}\left(f(x)\right)=\left(f(x)\right)'=\lim_{h \to 0} \frac{f(x+h)-f(x)}{h} \]

There are several different ways of writing the derivative, and you need to get used to them. The form \(\frac{d}{dx}\left(f(x)\right)\) reminds us that the operation of differentiation is itself a function: it takes a function in its domain and returns another function -- the derivative function.

• #2, p. 122.
• #23, p. 124
• #25
• #48, p. 125.