- Today we will talk about improper integrals (oh my!), after a brief review of numerical schemes.
- Let's head back into section 7.7: numerical integration (Numerical integration
handout). I want to go over one example that Steve highlighted.
- There are various schemes for numerical integration:
- Left-endpoint Rectangular Rule.
- Right-endpoint Rectangular Rule.
- Midpoint Rule.
- Trapezoidal Rule.
- Simpson's Rule.
- Three of the rules are so called "rectangle rules" (LRR, RRR, Midpoint Rule): if the width of each rectangle is $\Delta x = \frac{b-a}{n}$, then
Left-endpoint Rectangle Rule: \[ \int_a^b f(x) dx \approx LRR_n = {\Delta x}[f(x_0)+f(x_1)+f(x_2)+\cdots+f(x_{n-1})] \] Right-endpoint Rectangle Rule: \[ \int_a^b f(x) dx \approx RRR_n = {\Delta x}[f(x_1)+f(x_2)+\cdots+f(x_{n-1})+f(x_n)] \] Midpoint Rule: \[ \int_a^b f(x) dx \approx M_n = \Delta x [f(\overline{x_1})+f(\overline{x_2})+\cdots+f(\overline{x_n}) \] where $\overline{x_i}=\frac{1}{2}(x_{i-1}+x_i)=$ midpoint of $[x_{i-1},x_i]$.
- The Trapezoidal rule is really just the average of the LRR and RRRs. This gives rise to an important observation, which I want to encourage you to remember, and here it is:
When you have two estimates, you have a third. (their arithmetic average, in this case).
Trapezoidal Rule: \[ \int_a^b f(x) dx \approx T_n = \frac{\Delta x}{2} [f(x_0)+2f(x_1)+2f(x_2)+\cdots++2f(x_{n-1})+f(x_n)] \] where \[ \Delta x = \frac{b-a}{n} \] and $x_i=a + i \Delta x$.
- Simpson's rule is a "blend" (or best weighted average) of the Midpoint
and Trapezoidal rules, which perfectly balances the errors of the two
to generate a better rule:
\[
S_{2n}=\frac{2M_{n}+T_{n}}{3}
\]
Notice that the number of subintervals in Simpson's rule must be even.
Now we can go further:
When you have two estimates, you have infinitely many more. (their weighted arithmetic averages).
Here are the error bounds, that illustrate that the errors of midpoint and trapezoidal are related, and suggest how to combine them to create a better method (Simpson's rule):

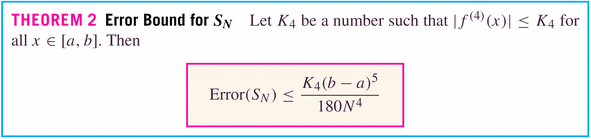
- There are various schemes for numerical integration:
- Let's look at Sect. 7.8: Improper
integrals. This is the last section we'll cover from chapter 7, and the
last to be included on your next exam.
- I'll use my own Integration cheat sheet to do the sample from Steve's page.