- Announcements:
- Your second homework assignment is returned.
- Babylonian numbers:
- 57 = (57)
- 222 = (3 42)
- 817 = (13 37)
- 9432 = (2 37 12)
- 14449 = (4 0 49)
- When you write your Babylonian numbers, don't forget
- to use boxes for positions, so you don't forget spaces for the
places.
- to block your martinis and boomerangs -- fish, fish, fish
- Never have more than 9 martinis in a slot
- Never have more than 5 boomerangs in a slot
- The table is a table of squares -- or square roots.
- We have a competition for the best
short story! I've scanned them in: you should pick your
favorite and we'll vote next time.
- You have an assignment due today (Mayan numbers), and another
due next time ("Fraudini" numbers).
- I've also assigned something for next Thursday, so I hope that
you're keeping up....
Question of the Day: What can we make of Yanghui's triangle??
- Last time we reviewed Mayan mathematical representation, and we
learned about the Great Fraudini and his amazing ability to
read minds. Today we'll review Fraudini, and then check out an
ancient Chinese document that represents one of the most
beautiful mathematical objects that I know!
- Last time I invited my
close friend, the Great Fraudini!
- How does the game work?
- Can you win at the game? You should be able to do
it every time!
- Here's the handout
for the cards, if you want to print off another
copy.
- What is the relationship to the primitive counting we did
earlier?
- Remember, computers only have two fingers (on and off -- thanks to Blake Nelms for that one!).
- A Card Trick!
Hint: this one relies on nothing more than counting.
- Four piles of cards
- My LA (lovely assistant) will bury three favorite cards in
the piles, yet I will find them!
- First card placed on top of the right-most pile
- an arbitrary number of cards cut from the second pile,
placed on the first pile, and
then the second card placed on top of the remaining cards in
the second pile;
- Ditto for the third pile.
- Then I stack them, engage in magic shenanigans, and
discover the cards!
Your task: How does it work?
- Today's new thing, the best new thing in the world, is an old thing. It's called "Yanghui's triangle":
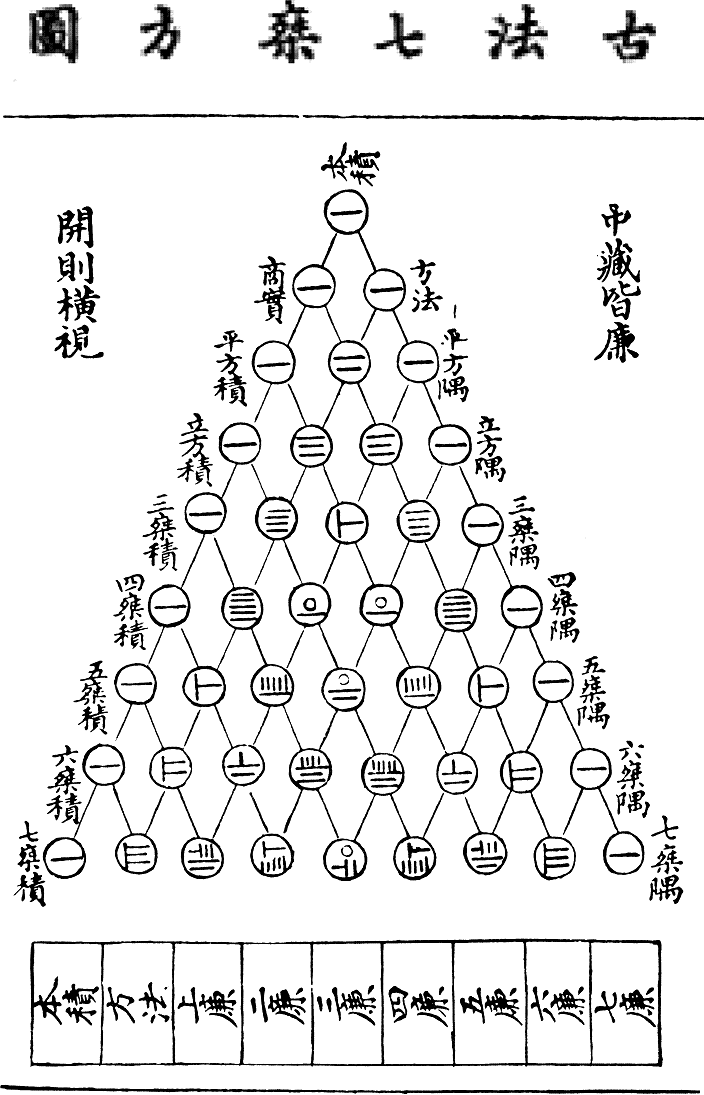
- Mathematicians look for patterns. What do you see?
- What is this? What is its purpose?
- One of your homework problems will be to translate this triangle,
so you need to learn its secrets!
Website maintained by Andy Long.
Comments appreciated.
