- You have a quiz today, on Babylonian and Mayan numbers.
- I'll start your quiz a little early today, because Kamora is
playing a basketball game which starts at 6:00.
I'm going to go over and check it out...:)
- Next week, Thursday, we have our first exam (on
2/27). Study up! Make sure that you do your readings,
as they will be a part of the exam.
- We'll have a review on Tuesday. We'll be wrapping up the number
unit on Monday.
- Sad to report that Vi Hart has gone kind of dark -- can't find binary finger dancing anywhere!:) But I did manage to find the Fibonacci videos that I wanted you to see on Vimeo.
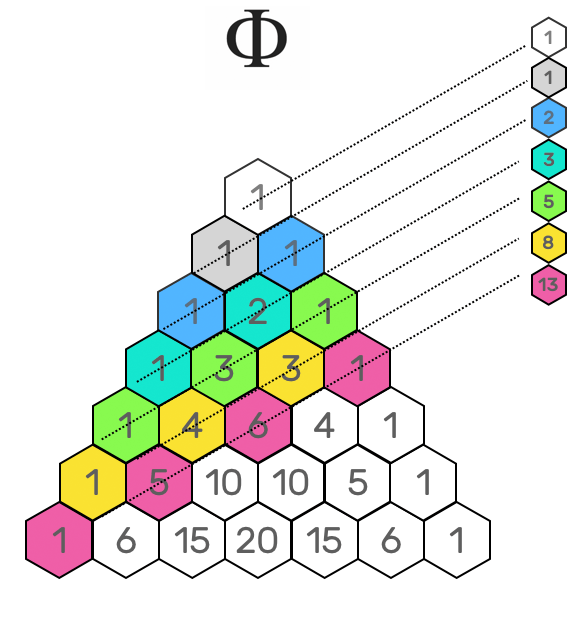
- We went back to Pascal's triangle to see that, in
addition to everything else in there, Pascal's
Triangle contains the Fibonacci numbers! But
they're hidden. Let's recall how to bring them
to light.
- We used the hexagonal paper to create a version of the Pascal triangle.
- Then those Fibonaccis found themselves on the hexagonal diagonals ....
- We figured out the strategy of Fibonacci Nim (remember, don't call
it that if you're going to play it against your opponent, as it will
give a strong hint about the strategy). Just call it "Nim" for example.
- The winning strategy is based on the third of our great
factorizations of the natural (counting) numbers, the Fibonacci
factorization:
Every natural number is either
- Fibonacci, or
- it can be written as a sum of non-consecutive Fibonacci numbers in a unique way.
- Let's start the play for a few games: if the number of counters is
the given number, do you go first or second, and what do you take if
you go first (by choice or by force)? Then do two more plays,
if played according to the strategy given....
- 31
- 41
- 55
- I read a
story a few years ago in the Guardian, about the science behind
Salvador Dali's works (such as The Sacrament of the
Last Supper):

This work features one of the topics we'll be studying down the road -- the Platonic solids. But it is also famously regarded as a painting in which the artist made conscious use of the golden ratio, which we cover today. The dimensions of this painting (the rectangular frame) create a (nearly) "golden rectangle" -- the most beautiful rectangle, according to some of the ancient Greeks.
- Fibonacci numbers also have a connection to this
beautiful concept of the golden ratio, and the golden
rectangle. We'll use ordinary graph paper to
illustrate that.
We're going to create what's known as a "Fibonacci Spiral". Here's the general idea:
- Start with a small square (which is also a rectangle); then
- append the largest square you can to the current rectangle.
- Do it again, and again, proceeding in a consistent sense (e.g. clockwise)
- From this we will migrate toward the so-called "golden mean" and
"golden rectangle". Now let's get down and dirty and create a
nice Fibonacci spiral (see this site for
more on these spirals).
Ordinarily we build the Fibonacci spiral by building bigger and bigger rectangles. The shapes of the rectangles change as we go along, in such a way that the ratio of side lengths are Fibonacci numbers. Let's look at the sequence of the ratios....
So let's break down the spiral building process, with a focus on those side ratios.
- Start with a piece of graph paper (the wide way), and darken the square in the 10,10 spot (ten from the left, ten down from the top). You might want several colors, if you've got them -- might as well make this look great!
- Rule: attach the largest square you can: first left, then down, and then continue in a counter-clockwise fashion.
- As you add each square you create a new rectangle. Compute the ratio of side lengths (larger over smaller).
- Do it again, do it again, do it again! (You could do it forever -- but we run out of paper.)
- What do you notice about the dimensions of the rectangle? It's the
ratio of those dimensions that interests us most -- the ratio
of dimensions (larger to smaller) dictates the shape of the
rectangle. Two rectangles that have the same ratio have the
same shape.
1x1 1/1= 2x1 2/1= 3x2 3/2= 5x3 5/3= 8x5 8/5= 13x8 13/8= 21x13 21/13= 34x21 34/21= 
- The thing we are approaching is the so-called golden rectangle,
whose side-lengths are in the ratio called "the golden
ratio". But we will derive the golden rectangle in a different
way -- the way that the Greeks did it! When we're done, we'll
have also defined the golden mean, aka the golden ratio.
- Begin with a rectangle.
- Remove the largest square possible from the rectangle, to leave a rectangle.
- If the rectangle that results has exactly the same shape as the original
rectangle, then that rectangle is golden:
However if we look back at the Fibonacci spiral sequence of rectangles as they're growing, we see that they're tending toward a "golden rectangle".
-
If you did your reading (and of course you did!), you know that the
ratios of successive Fibonacci numbers tend toward a certain number,
which is called the "golden mean" or "golden ratio":
\[
\varphi=\frac{1+\sqrt{5}}{2} (\approx 1.618033988749895)
\]
Let's show that this golden ratio is, in fact, the side-length ratio of the golden rectangle described above. And our secret weapon will be your old friend, that old favorite, the quadratic formula!
While we might think about the Fibonacci spirals as being created by attaching squares, the golden rectangle is created by removing squares. We just do things backwards....
We can build beautiful rectangles by pasting squares together, or we can define beautiful rectangles by taking them away....
- Now what can we do with these ideas? Here is a somewhat silly
example -- A Fibonacci spiral fractal comic I made recently, which I
have titled "Flirting with Death Spiral". Infinite fun!

(sidelengths: 466x288, with ratio 1.6180555555555556!)
You'll be making these, too, soon, using your own photos. Let's do one in class now (if we're lucky this will work!)
- By the way, Salvador Dali's image is sized 334x208: side ratio, 1.6057692307692308
- Fibonacci
Spiral Fractal maker, to make images like these:

- How to win at Nim
- Logo Design using the Golden Ratio
- A review of the Mayan calendar we study, Unearthing the Heavens: Classic Maya Murals and Astronomical Tables at Xultun, Guatemala , including the reference to this article from the journal Science: Ancient Maya Astronomical Tables from Xultun, Guatemala, and this more "popular" interpretation of the calendar.
- Do math like an Egyptian! (Walk Like An Egyptian/Michael Jackson Mash-up!)
- Hexagonal Paper
- Pascal's triangle (wikipedia)
- American Bandstand dances to Gimme Some Lovin', a hit by The Spencer Davis Group: here's their version.
- List of musical works in unusual time signatures
- Blue Rondo a
la Turk, by Dave Brubeck: illustrating
how to play a piece in 9/8.
Watch Dave Brubeck's leg, counting out the rhythm....