Day 11, MAT115

• This session will be captured on Zoom, if I remember to turn it on, and record it.

  Our page of zooms and the play-by-plays.

• Roll

• The Perfect Valentine? A Math Formula: Nothing says "I love you" like a customizable algebraic equation.

• You have a new assignment.

• Your second IMath homework is due today.

• As I write this (Friday night), no idea how the Bengals are going to do. But I do know this:

  "Now the Joe Burrow Hunger Relief fund at the nonprofit Foundation for Appalachian Ohio has grown to \$1.5 million, with fans donating nearly \$100,000 in the days since the Bengals clinched their Super Bowl berth. Fans often donate in multiples of nine -- Burrow's jersey number, the foundation said." (my emphasis; source)

We answered the question

• It's the thing that makes the Fraudini's trick work.

• This is the second great factorization of the counting numbers. So it turns out that the counting numbers and sums of distinct powers of two can be put into one-to-one correspondence (if we count single powers of two as "sums"...).

• Here are the first few powers of 2:

  \[ \begin{array}{l} 2^0 = 1 \cr 2^1 = 2 \cr 2^2 = 4 \cr 2^3 = 8 \cr 2^4 = 16 \cr 2^5 = 32 \cr 2^6 = 64 \cr 2^7 = 128 \cr 2^8 = 256 \end{array} \]

  (Do you see why the Great Fraudini can only read numbers up to 63?)

• So in base 10 we think of "93" as \[ 93 \textrm{ (nine tens and three ones) } = 9*10 + 3*1 = 9*10^1 + 3*10^0 \]

  That last bit on the right shows 93 as a sum of "weighted" powers of 10 -- "weighted" because we have to say just how many tens we need (nine in this case) and how many ones we need (three of them in this case). For binary numbers the "weights" are easy: 1 or 0: \[ 93=64+16+8+4+1=2^6+2^4+2^3+2^2+2^0 \] Or we could write \[ 93=1*2^6+0*2^5+1*2^4+1*2^3+1*2^2+0*2^1+1*2^0 \] or, better yet, $93_{10} = 1011101_2$ -- 93 base 10 is equal to 1011101 base 2.

• Much like the prime factorization, we can use a tree to break down a number into its binary representation. Let's give it a go for a few examples:
  • Now let's use a tree to find the binary factors of 437.

• Trinity noticed something when we were doing the Fraudini trick that's important: each card showed a different pattern;
  1. the "1" card is all the odds -- every odd number will have to include a 1. You can think of that as a skip of one.
  2. The "2" card starts 2,3, then skips two (because 4 picks up the slack!).
  4. The "4" card starts 4,5,6,7, then skips four (because 8 picks up the slack).
  8. The "8" card starts 8,9,10,11,12,13,14,15, then skips eight (because 16 picks up the slack).
  16. The "16" card starts 16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31, then skips sixteen (because 32 picks up the slack).
  32. The "32" card starts (and ends), with 32 numbers from 32 to 63 -- at which point 64 would have picked up the slack, had we wanted to use more than six cards.

• What else can you do with binary numbers? binary hand dance, of course!

  Vi Hart has the most amazing ways of showing us interesting mathematics. She shows us that we can count to 31 on one hand. And, if you'll use the 10 fingers of two hands, you can get all the way up to $2^{10}-1=1023$...

  Some people can only count to 10 with their fingers....

• Today's new thing, the best new thing in the world, is an old thing. It's called "Yanghui's triangle", and you should look yours over carefully:
  

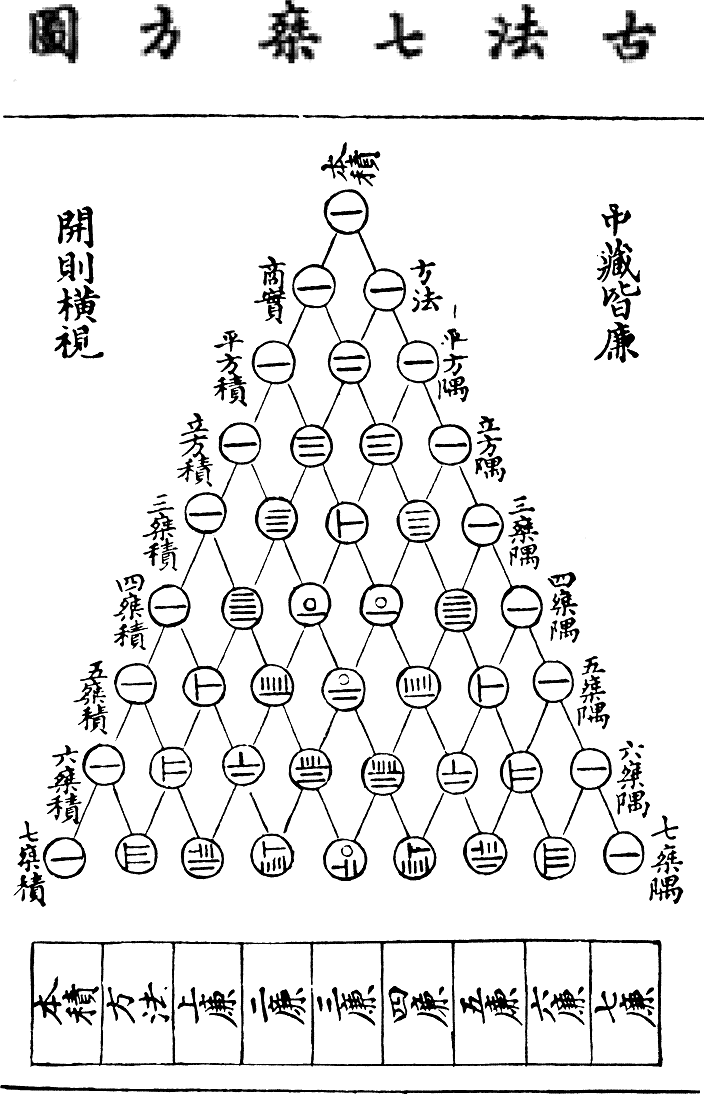
  Let's try to answer some questions: Mathematicians look for patterns.

  • What do you notice? What catches your attention?
  • Can you find any number patterns?
  • Can you understand the writing system for numbers?
  • What is this thing? What is its purpose?

• If we have time, we're going to create a more modern version of this triangle on the back of your sheet.

  There's a rule that we want to decipher for this triangle....

• The Perfect Valentine? A Math Formula: Nothing says "I love you" like a customizable algebraic equation.