Day 22, MAT115

• This session will be captured on Zoom, if I remember to turn it on, and record it.

  Our page of zooms and the play-by-plays.

• You have an IMath assignment due today, 3/18.

• This is the last time we must wear the masks in class; and then I think that I, at least, will be maskless. You will be welcome to mask or not in class, in the future.

• Your Egyptian multiplication and division homework is due Wednesday, 3/23 (submit on Canvas)

• I just graded your Babylonian and Mayan translations, and there were two general problems I saw that need a comment:
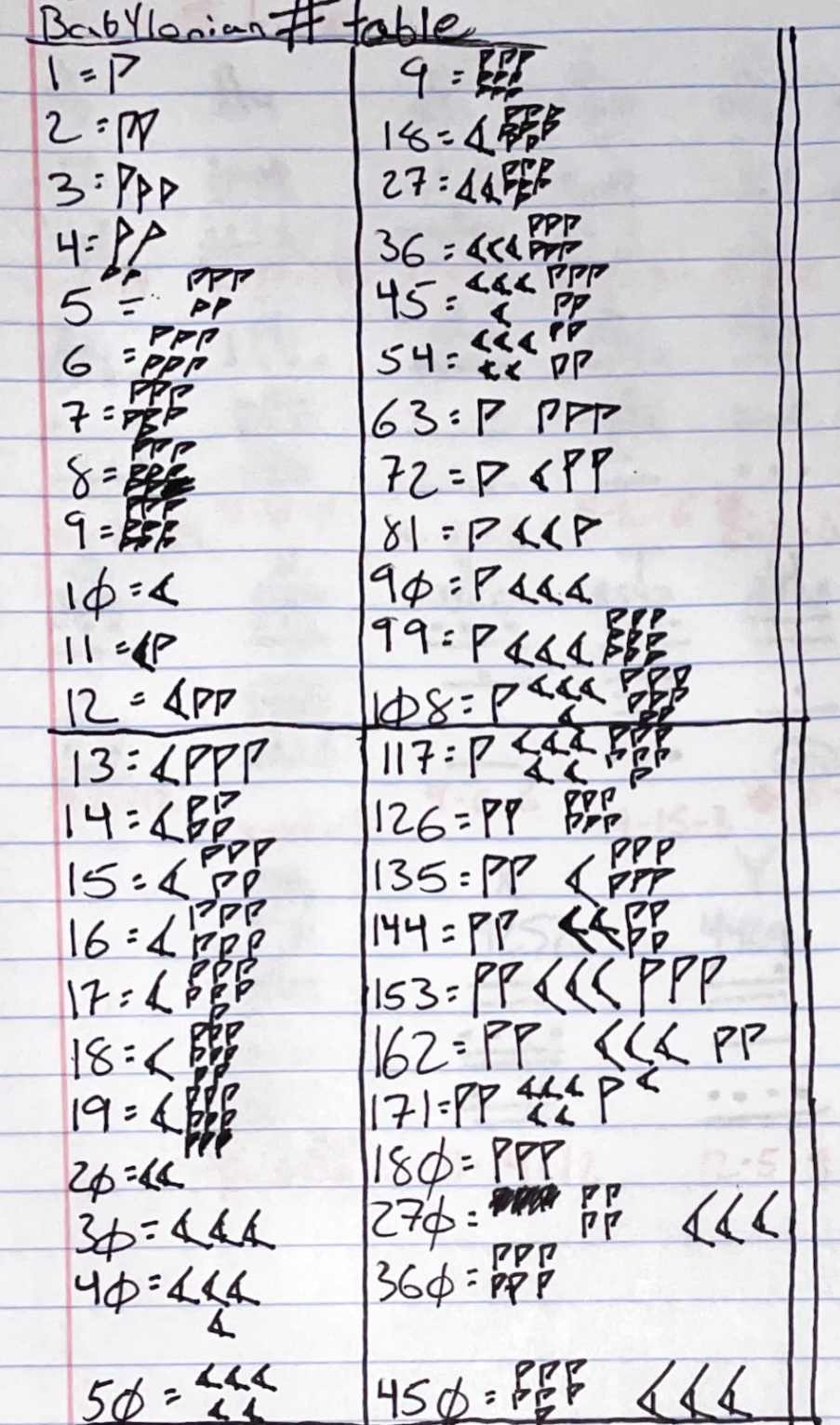
  1. Babylonians: this is a nine times table: on the left is a number, and on the right is its multiple of 9.

     Once you figure that out, you can use that pattern to fill in the table. But you should check your work to make sure that everything is consistent.

     

  2. Mayans: some of you noticed a pattern -- that the numbers increased by 177 each time. And you went with it. It's great until it fails... at G.

     It's sort of the Mayan version of a leap year -- an extra day every now and then to keep the calendar on track.

• Review of Egyptian multiplication, followed by a start to division.

We began with Egyptian multiplication, which is based on the fact that the Egyptians didn't mind doubling things: neither did they mind halving things, and that explains their first tricks with division.

Successive doublings means powers of two, so this reduces to the Fraudini trick! That's the good news.

• Let's begin again with a simple example: multiply 57*73.

  We double the larger of the two, generally, and use the binary factorization of the other to choose which rows to include in the final answer (indicated by the asterix):

  57=binary factorization = 32+16+8+1	1 73 * 2 4 8 * 16 * 32 * 64 Too big!	appropriate sum of doublings = 4161
  Multiplication:
  Build on the left	Doubling in the middle	Answers on the right
  Division:
  Answers on the left	Doubling in the middle	Build on the right

• But we could just as well think of this as a division problem:

  We could say to ourselves "Clearly $\frac{4161}{73}=57$" -- we might have just as well doubled 73, then formed 4161 with multiples of 73, and deduced the answer 57 (working "right to left", so to speak).

• What mucks it up is when things don't come out even.

  Let's look at another example: divide 30 by 7.

  1	7
  2	14
  4	28	*
  1/7	1
  2/7	2	*

  Note how we've doubled the 1/7 fraction -- those Egyptians loved doubling things! And that's why the table has "2" in the numerators. Those are doubled unit fractions -- that is, fractions with a numerator of 1, which can be converted back to unit fractions.

  So the answer is 4 + 2/7. But that won't suit the Egyptians: they only want unit fractions in their answers. So we consult the unit fraction table (their cheat sheet): \[ 4 + 2/7 = 4 + 1/4 + 1/28 {\textrm{ (and that's the answer they want!)}} \] Now isn't that handy?

  Remember that the Egyptians wouldn't accept answers with repeated fractions -- so it's not an option to write \[ 4 + 2/7 = 4 + 1/7 + 1/7 \] While it's true, they wouldn't have accepted that as a valid answer.

• The Egyptians restricted themselves to the so-called "unit fractions", which are fractions of the form 1/m: unit fraction table, which is found on the Rhind Papyrus (which dates to around 1650 BCE).

  Last time we did this example, somewhat painfully: divide 6 by 7.

  1	7
  1/2	3+1/2	*
  1/4	1+1/2+1/4	*
  1/7	1
  1/14	1/2	*
  1/28	1/4	*

  So the answer is 1/2+1/4+1/14+1/28 (we usually order them from largest to smallest).

  Notice that the Egyptians didn't use decimals -- you shouldn't either!

  Why did Egyptians do things this way? (an example division problem, 3/5):

  Dominic Olivastro, "Ancient Puzzles", suggests a third reason why 
  this use of unary fractions is good. Consider the problem Ahmes poses 
  of dividing 3 loaves of bread between 5 people. We would answer "each
  person gets 3/5-ths of a loaf". If we implemented our solution, we might
  then cut 2 loaves into 3/5 | 2/5 pieces, with bread for 3 people; then
  cut one of the smaller pieces in half, giving the other two people 
  2/5 + 1/5 pieces. Mathematically acceptable, but try this with kids and
  they will insist that it is not an even division. Some have larger pieces,
  some have smaller.
  

  

  Ahmes would calculate 3/5 as :
     3/5 = ()3 + ()5 + ()15    [ = 1/3 + 1/5 + 1/15 ]
  Now cut one loaf into fifths, cut two more into thirds, then take one of
  the 1/3-rd pieces and cut it into 5-ths (for the 1/15-th pieces), and you
  can now distribute everyone's 3/5-ths share in a way that _looks_ equal,
  since they will have exactly the same size pieces. (And no, I don't want
  to argue about the crust.)
  

• There is another way to get these answers, using the Fraudini trick and the Unit Fraction Table. So let's try those same examples but using the unit fraction table rather than our doubling tables.
  1. $\displaystyle \frac{3}{5}=\frac{2+1}{5}=\frac{2}{5}+\frac{1}{5} =\frac{1}{3} + \frac{1}{15} +\frac{1}{5} =\frac{1}{3} + \frac{1}{5} +\frac{1}{15} $

  2. $\displaystyle \frac{35}{8}=\frac{32+2+1}{8}=4+\frac{1}{4}+\frac{1}{8}$

  3. $\displaystyle \frac{6}{7}=\frac{4+2}{7}=\frac{4}{7}+\frac{2}{7} =2\frac{2}{7}+\frac{2}{7}$

     so we look up $\frac{2}{7}$, and find that $\frac{2}{7}=\frac{1}{4}+\frac{1}{28}$. Therefore,

     \[ \displaystyle \frac{6}{7}= 2*(\frac{1}{4}+\frac{1}{28})+\frac{1}{4}+\frac{1}{28} = \frac{1}{2}+\frac{1}{14}+\frac{1}{4}+\frac{1}{28} = \frac{1}{2}+\frac{1}{4}+\frac{1}{14}+\frac{1}{28} \]

  which is exactly what we got before.

  

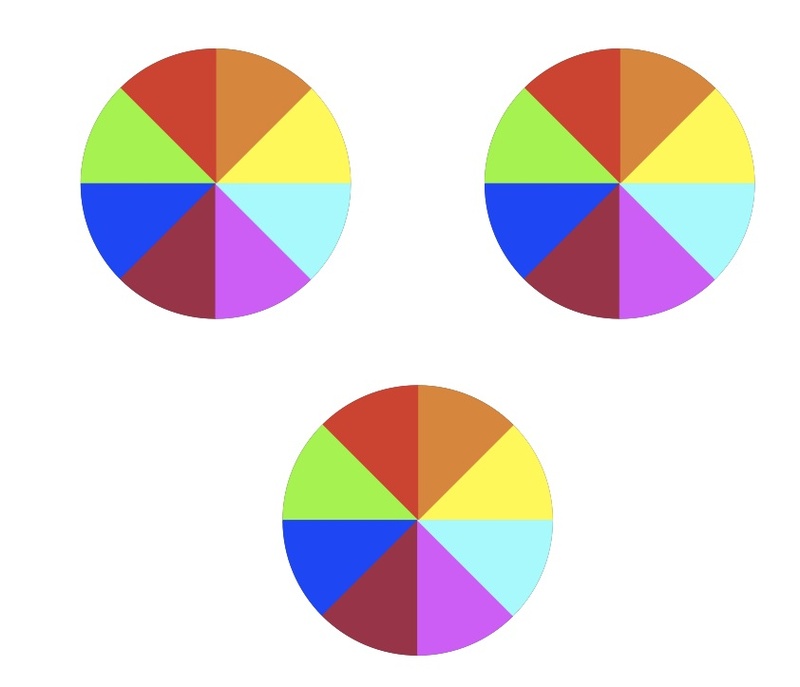
• Here's a relatively easy one: Suppose Fatima had 3 pies to share between 4 people. How would she do it? (Think about what the answer means, in terms of pie, and keeping kids happy.)

  This image suggests a strategy, but it's not optimal -- it requires cutting your pies into smaller parts than necessary -- so that we have a multiple of 4 of pieces. How should you make your cuts?

  

• A little trickier:
  1. How would you divide 5 by 7?

     1	7
     1/2	3+1/2	*
     1/4	1+1/2+1/4
     1/7	1	*
     1/14	1/2	*

  2. How can we use the unit fraction table to get the same answer?

     \[ \frac{5}{7} = \frac{4+1}{7} = \frac{4}{7}+\frac{1}{7}=2\frac{2}{7}+\frac{1}{7} = 2\left(\frac{1}{4}+\frac{1}{28}\right)+\frac{1}{7} = \frac{1}{2}+\frac{1}{14}+\frac{1}{7} \]

     You don't have to play by the book: for example, you might write this: \[ \frac{5}{7} = \frac{3+1/2+1+1/2}{7} = \frac{3+1/2}{7}+\frac{1}{7}+\frac{1/2}{7} = \frac{1}{2}+\frac{1}{7}+\frac{1}{14} \]

• How would you like to do story problems like this one?!