Day 39, MAT115

• This session will be captured on Zoom, if I remember to turn it on, and record it.

  Our page of zooms and the play-by-plays.

• You have an IMath assignment on the go, covering symmetry, and Platonic solids, and fractals (due today, 4/27 -- revised).

• A reminder that your logo projects are due this coming Monday. Be ready to present!

  You all need to be here on Monday.

  If you are not here on Monday, then you have been pre-selected to present on Monday -- i.e., your logo will receive a zero.

  Here are some galleries of logos from years past. How would you appraise them?

• You have a couple of reading assignments:
  1. Read Chapter 30: The Hilbert Hotel (for next class!)
  2. Check out this summary of our work today: Knots: a handout for math circles.
  and a new assignment to hand in (due Friday of next week).

• Read an interesting opinion piece in the Washington Post yesterday, and wanted to share what this writer (Damon Young) said:

  You would also know -- if you were better at this than I am -- that sentences are music. And that both sentences and music are math. Equations. Beats separated by pauses. Microbursts of energy clustered and cut and culled to find balance. You would know that sometimes "ain't" just fits in a way that "isn't" or "is not" does not. Same with "them" instead of "those." You would know that even the choice of "does not" at the end of the above sentence instead of "doesn't" was intentional, because of the repetitious rhythm of "does not" existing immediately after "is not." You would know that short phrases lead to shorter sentences, which punch in a way that longer ones sometimes can't. Like this just did.

• was a golden rectangle set of Borromean rings, whose corners form an icosahedron: we created the RCO -- Really Cool Object -- which brings together the Borromean rings, golden rectangles, and Platonic solids (an icosahedron).

  This is the most beautiful piece of mathematics I know:

  

  To take three rings (the cards are "rings"), and to turn them into Borromean rings, we had to make a cut -- just one cut -- but we had to make a cut, because we're changing between two topologically different things.

• There are two different ways to think about making the trefoil knot: as a
  1. torus knot, and as a
  2. twist knot.
  Let's draw it in these two different ways.

• The figure-eight knot has four crossings, as does Solomon's "knot", but it's a link, not a knot. This is the first (interesting) instance of a knot and link with the same (minimal) number of crossings.

• We know that there are two distinct 5-crossing knots:
• To help you, we have the Rolfsen Knot Table
• The cinquefoil knot
• The five-crosssing twist knot is actually known (somewhat confusingly) as the 3-twist knot
• How can we tell them apart? Are they really different? For that we need to study knot equivalence, and our primary tool, the Reidemeister moves.

How do we show that two knot projections ("squiggles") are the same knot ("knot equivalence")? That's the job of the Reidemeister moves.

1. What are the Reidemeister moves?
   • Tools for showing that two projections (pictures) of knots really show the same knot.
   • Names of the moves reflect the number of strands involved. A Strand is a piece of a knot picture that goes from undercrossing to undercrossing, with only overcrossings inbetween.
   • Reidemeister moves preserve the knot -- they don't change the knot. We're not cutting -- we're just going to shove parts of the knot around.

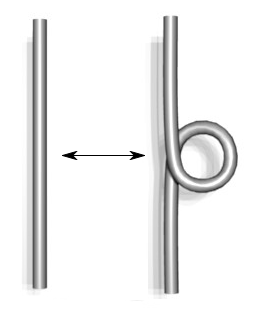
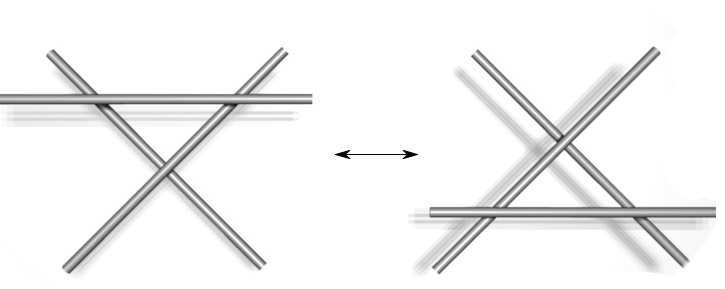
   • Type I	Type II	Type III

2. Using the Reidemeister moves
   1. to turn a projection of an unknot into another more complicated appearing unknot:

      We'll just try a few with a string, to see what we can learn.

   2. Now let's use these three moves to create equivalent versions of the trefoil knot, but using each of the three Reidemeister moves.

   3. A figure-eight knot is equivalent to its mirror reflection (a trefoil knot is not equivalent to its mirror!)

   4. Turning a more complicated appearing unknot into a simple circle unknot. (Here it is as a powerpoint presentation.)

3. Reidemeister moves and Tricolorability
   1. Tricolorability: "A knot is tricolorable if each strand of the knot diagram can be colored one of three colors, subject to the following rules:
      1. At least two colors must be used,
      2. at most three colors are used, and
      3. at each crossing, the three incident strands are either all the same color or all different colors.

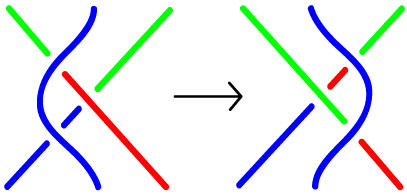
   2. Tricolorability is preserved by Reidemeister moves:

      Reidemeister Move I is tricolorable.	Reidemeister Move II is tricolorable.	Reidemeister Move III is tricolorable.

   3. Therefore, if the projection of one knot is tricolorable and the other isn't, they're different knots (because we can't get from one to the other via Reidemeister moves).

   4. Theorem: there are at least two different knots.
      1. The unknot is not tricolorable ("At least two colors must be used");
      2. The trefoil knot is tricolorable:
      3. The figure-eight knot is not tricolorable (it requires four colors):
      4. Danger: many knots are tricolorable -- being tricolorable doesn't mean that your knot is the trefoil knot -- but it does mean that your knot is not the unknot!

         Links can be tricolorable, too -- for example, the unlink is tricolorable! (That's just two circles, one lying on top of the other, as in Borromean rings.)