Day 26, MAT115R

It turns out that they can all be drawn without any edges intersecting each other. This is one of the important concepts we continue to discuss today.

We also saw that we could draw the dual platonic solids from a drawing of a Platonic solid, but connecting vertices drawn into the center of each face in the appropriate ways -- e.g. the octahedron inside of the cube.

• This problem, solved by Leonhard Euler (1735) in the negative (spoiling all the fun of the good people of Konigsberg), was the beginning of graph theory.

• Euler's solution:
  • Extract out only the important information into a graph (which, to that point, hadn't been invented!):
    

  • Euler's Konigsberg solution: To be traversable (i.e. have an "Euler path"), a graph must have at most two vertices of odd degree.

    And since the graph of Konigsberg has four odd-degreed vertices, it can't be traversed with an Euler path.

  • Euler also discovered the "hand-shaking theorem": in any graph, the number of vertices with odd degree must be even.

    That means either 0 or 2 odd vertices.

  • If there are 0 odd vertices, you can start from any vertex and find an Euler path, which comes back to the original departure vertex; if there are two odds, then you must start at one odd, and end at the other.

    An alternative solution, to illustrate.

    Is the pentagram gram traversable? Is there an Euler path?

    
  • "Floor plans" of houses either have an Euler path, or they don't. The rooms are like land masses, and the doors are like bridges.
    • Let's try one! These sorts of puzzles often show up in "ACT-like" tests.
    • Draw a floor plan of your home (or one level of it, if there are multiples). Is there an Euler path passing through each door exactly once? (Bedrooms often mess things up; add windows as bridges!)

The tetrahedron can be drawn as a Mercedes Benz symbol

• Draw all the distinctly different simple graphs with three vertices.
  • You don't need to repeat graphs with a single edge, because one can be obtained from another by rotation. They're the same graph!
  • A cycle is a route from a node back to itself that doesn't retrace steps.
  • One of these graphs is a (non-rooted) tree: a connected graph that has no cycles.
  • Duality -- again!

• Draw all the distinctly different simple graphs with four vertices.
  • Have you ever seen any of these before? Could we give any of them names?
  • Sometimes it's hard to tell if two graphs are actually the same... We say "isomorphic".
  • Can you draw them in a way that emphasizes symmetry?
  • Can you draw them in a way that emphasizes duality?

• These drawings represent all the structurally distinct kinds of Facebooks you can have with a given number (three or four) of individuals.

  (I.e., where we don't give labels to the individuals)

• Every Platonic graph can be drawn this way; they are, in fact, simple planar graphs.

• As a "homework" (for your own good!), I have asked you to cut out and create Platonic solids from paper, using this template.

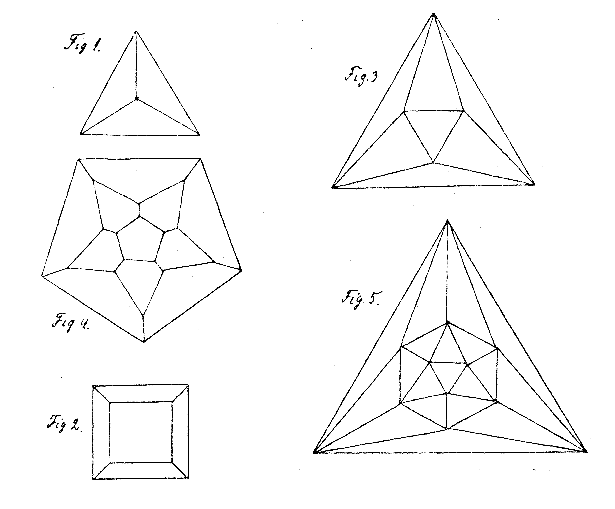
  Let's inspect these drawings, and see why these five configurations of polygons work: while two of these polygons can tile the plane (the square and the triangle), we throw out enough neighbors of each of the polygons so that there's room to fold up the cut-outs.

  The pentagon won't tile the plane -- there must be space between some pentagons. Any polygon with more than five sides will be "too cramped" to fold up....

  Note: these are not graphs of the Platonic solids, because many of the "edges" are redundant, and there are too many vertices.

  Last time we showed that, although these graphs are not projections of the Platonic solids, we can use them to create graphs of the Platonic solids -- by "connecting vertices drawn into the center of each face in the appropriate ways -- e.g. the octahedron inside of the cube."

  And notice that we could draw all of them without any false intersections -- we could draw them as planar graphs.

• Euler also discovered a formula for connected, planar graphs: \[ F-E+V=2 \] where F is the number of faces, E the number of edges, and V the number of vertices.

  A reminder that a graph is connected if there is a path (a means of moving from one vertex to another along edges) from each vertex to every other vertex.

  If we check our table for the Platonic solids, we'll see that they all hold up.

  It turns out that it also works for soccer balls, and other polyhedra. The countries on a globe, for example. Actually anything that can be projected onto planar graph!

  Euler's formula is also what's wrong with six-sided polyhedra (and beyond).

  The argument against six-sided polyhedra involves Euler's formula, as Weyl discusses.

• Which of the Platonic solid graphs can be traversed without backtracking?

• We can show that the complete graph with four vertices is actually planar. We may have to bend an edge, however....
  

• Let's see if we can try to show why the complete graph with five vertices, \(K_5\), is not planar:
  

  Why isn't it planar? What goes wrong?

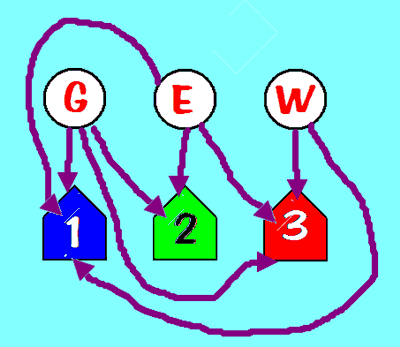
• The three utilities and three houses graph is not planar:
  

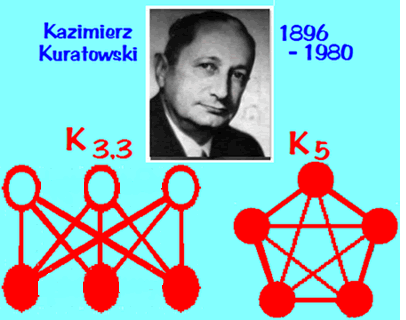
• Any non-planar graph has a copy of one of these two graphs in it somewhere, as a subgraph:
  

I've assigned you a reading from The Joy of X by Steven Strogatz. Let's talk about is The Enemy of My Enemy (complete graphs, labelled graphs)

• One focus of this reading is negative numbers. It was the Indians (of Asia) who put negative numbers on a firm foundation (as well as zero), in about the 6th century AD.

  "...you can't see negative 4 cookies and you certainly can't eat them -- but you can think about them...." ("and you have to", says our author).

• One of the confusing aspects of negative numbers from Strogatz's perspective is that the product of two negative numbers is a positive number.

• But then Strogatz goes on to use the context of social interactions (negative and positive) to discuss how we maintain stable relationships.

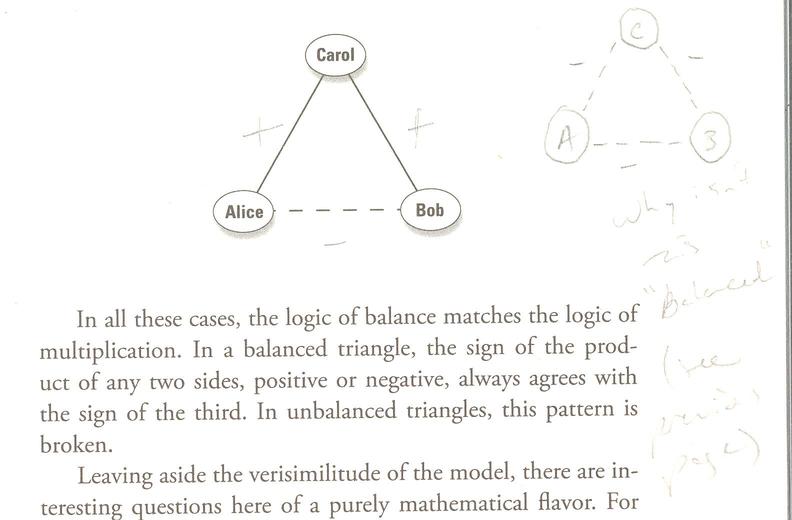
  And the key to understanding stability in three-way social relationships is that the product of two interactions (signified by either +1 or -1) must be equal to the other: so that if two legs are positive, the third in the triangle must be positive; if one leg positive, and the other negative, then the third leg must be negative as well.

  

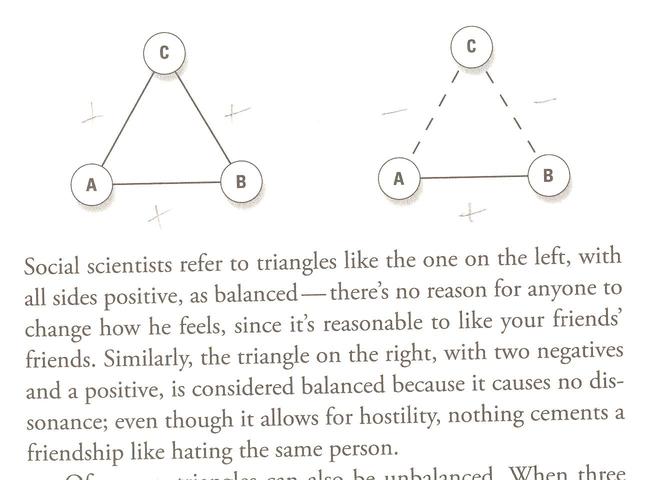
  Strogatz sums up the second case above in the familiar saying that "The enemy of my enemy is my friend".

  The following (two) graphs are unbalanced:

  
  Why is the all negative graph also unbalanced? Because "the enemy of my enemy is my friend!"

  Finally Strogatz shows how historical relationships settled down into this pattern of stability: in "...the run-up to World War I. The diagram that follows shows the shifting alliances among Great Britain, France, Russia, Italy, Germany, and Austria-Hungary between 1872 and 1907."

  

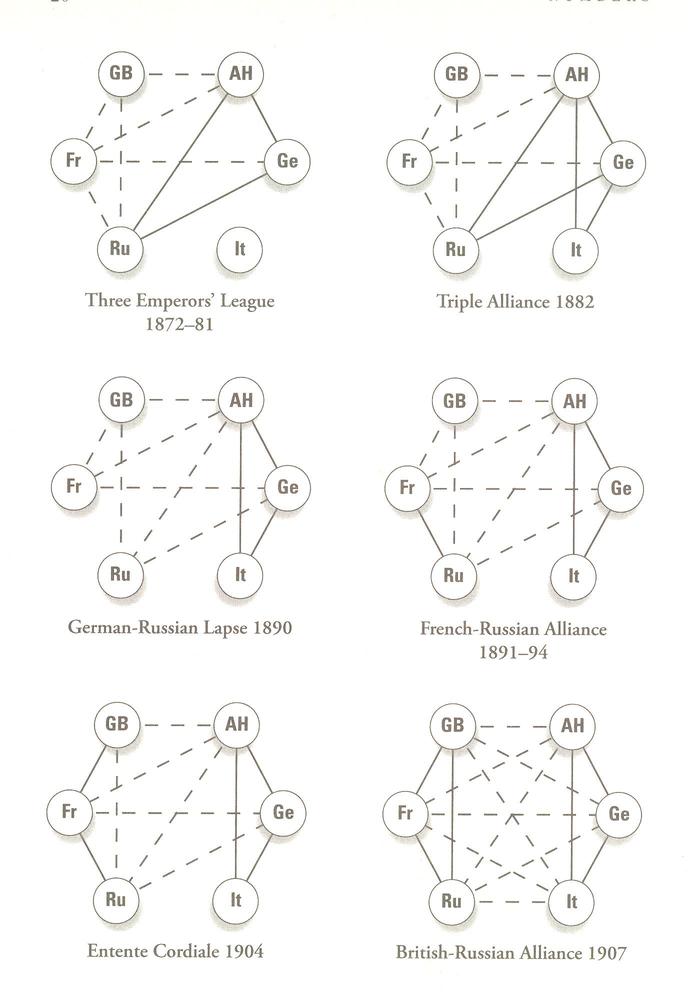
  The bottom right graph (complete!) is the only stable configuration, "...balanced, but on the brink of war."

  Notice that Italy, which was not bothered by any of the powers of GB, F, or Ru, became a target -- because the friend of my enemy is my enemy, too!

  How do we know that the final graph is non-planar?

This paper contains a lot of references, and on-line resources to document their work.

"Because the human brain is so good at detecting faces, we sometimes see them where they do not exist. Were you ever scared as a child by strange faces popping up from an abstract wallpaper design or formed by shadows in the semidarkness of your bedroom? Ever notice that cars seem to have faces, with the headlights as eyes and the grilles as mouths? These effects result from the face-recognition circuits of our brains, which are constantly trying to find a face in the crowd."