Day 39, MAT115R

Oops! Sorry folks, I must have left your quizzes at home....:(

• the quiz
• the key

I'll collect those now....

Clearly, though, some of you are still struggling with overs and unders. You have to show the bridges!

Among our collection of knots and links, only the trefoil and the unlink are tricolorable.

Let's do one more example (or a few) from our Tricolor These Handout: just two of the knots/links are not tricolorable; all the rest are!

John Newton 1725-1807 (stanza 6 Anon); here's the Snopes fact-checked story of the author, a slave trader.

In the bulb there is a flower,
in the seed, an apple tree,
in cocoons, a hidden promise:
butterflies will soon be free!
In the cold and snow of winter
there's a spring that waits to be,
unrevealed until its season,
something God alone can see.

In our end is our beginning,
in our time, infinity;
in our doubt there is believing,
in our life, eternity,
in our death, a resurrection,
at the last, a victory,
unrevealed until its season,
something God alone can see.

"Alice laughed. 'There's no use trying,' she said. 'One can't believe impossible things.'

I daresay you haven't had much practice,' said the Queen. 'When I was your age, I always did it for half-an-hour a day. Why, sometimes I've believed as many as six impossible things before breakfast."

• Achilles and the Tortoise (the Tortoise beats Achilles.)
  

• The Dichotomy paradox (Motion is impossible.)
  

• "I hate you."
• "I hate you twice as much as you hate me!"
• "I hate you twice what you hate me, plus one!"
• Eventually it gets to infinity, right?

Natural numbers are things which have a size, and if you add one to a natural number, you get a bigger number.

You don't get "a bigger number" -- more hate -- when you hate someone "infinity plus one".

In part because you don't have a number to begin with; but trying to add a number (1) to NaN doesn't work to give you a bigger number.

Two sets have the same cardinality if there is a one-to-one correspondence between them.

Intuitively: We will say that two sets have the same size if they have the same cardinality.

And there's the fact that Hilbert himself introduced the analogy of the infinitely roomed hotel...:)

• Hilbert's Hotel: "it's always booked solid, but there's always a vacancy."
• Hilbert's Hotel and the Really Big High School Band
• Hilbert's Hotel and the Really Big Really Big High School Band Competition (RBRBHSBC)

1. The place is packed, and one person shows up;

   This result informs us that when someone on the playground hollers "I hate you infinity plus 1!", they really haven't hated any more than a simple infinity.

   Alternatively, if your lover says they love you infinitely much, you can't impress them by saying that you love them infinitely plus one. They will scoff, and perhaps leave you for a better mathematician! So take note....

2. The place is packed, and an infinite school bus shows up.

   This result informs us that when someone on the playground hollers "I hate you 2*infinity!", they really haven't hated any more than a simple infinity.

3. The place is packed, and an infinite number of infinite school buses show up! (Buses numbered 1, 2, .... with seats labelled 1, 2, ....)

   This result proves that there are just as many rational numbers (ratios of integers) as there are integers.

4. But if a bus containing the irrational numbers shows up -- now there's an infinite set! It's too big for the hotel...:( Finally the Hilbert Hotel meets its match!

   The real numbers (containing both the rational and irrational numbers) is just too big for Hilbert's Hotel. Mathematicians' guts lead them to believe (generally) that the real numbers are the next largest infinity (the first "uncountable" one).

5. The Hilbert Hotel shines a light on a strange fact about infinity: that when you get to sets that are infinitely sized, you find that their subsets can be exactly the same size as they are. This is very strange, and doesn't work with finite sets. So it's a very mysterious property for those of us accustomed to working with finite things....

1. Some mathematical facts:

   Mathematical fact zero:

   A set S is just a collection of objects; and a subset of S is just what you'd expect: some members of S. It may be all the members, in which we call it an "improper" subset, or it may be none of them (in which case we call it the empty set).

   We often denote a set by using braces, e.g. \(S=\{1,2,3\}\) is the set of the first three natural numbers.

   We say that \(a\) is an element of \(S\) if \(a\) is contained in \(S\), and we write \(a \in S\). So \(1 \in S\), \(2 \in S\), and \(3 \in S\). We deny that an object is in \(S\) this way: \(4 \notin S\).

   Mathematical fact one:

   A subset is never bigger in size than the set itself.

   And if the sets are finite, the proper subset is always smaller, but if the set is infinite, we may actually be able to throw away elements of a set and not change the size of the set!

   Mathematical fact two:

   Let's call the set of all of a set S's subsets its power set, \(P(S)\). Pascal's triangle (that rascal!) shows you how many of each type of subset you have (for finite sets). Let's look at "line 3" (that is, 1 3 3 1):

   

   It's about how many ways we have of choosing \(r\) things from \(n\), right? That's exactly what a subset of \(r\) elements is: a choice of \(r\) things from \(n\). Here are the four different groups of subsets represented by that line:

   

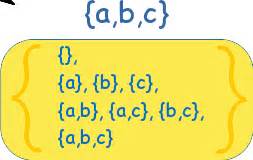
   Mathematical fact three:

   The power set of a set of $n$ elements contains $2^n$ elements.

   Imagine that each of the \(n\) elements is a person, who decides if they're going to stand up and join a subset, or not. Each person has two choices, and they make them independently. Thus we have \(2 \cdot 2 \cdot \ldots 2\) -- \(n\) times -- ways of making a choice of a subset, of choosing.

   (We know that since each row of Pascal's triangle adds to a power of 2, and the elements in each row represent all the different subsets of each size possible, from 0 up to \(n\).)

2. Even the set containing no elements has one subset -- the set itself. So the power set has one element -- has size bigger than the set itself.

   This property holds true for all finite sets -- and it turns out to be true for infinite sets, too!

   Here's a silly video to illustrate how the power set grows with sets of increasing size. (Thanks to Dr. Towanna Roller (Asbury University) and her daughter Kristyn Roller (UK) for this one!)

3. This means that, although the natural numbers $1,2,3,\ldots$ is infinite as a set, there's a bigger set (the power set of the natural numbers).

   And the power set of that set is bigger yet, and so on forever, forever, Hallelujah, Hallelujah!

4. Upshot: infinity comes in various sizes! Infinitely many, in fact. Crazy sounding, I know -- but it's true.

   That symbol that you've been familiar with for all your lives, $\infty$: you thought it stood for a single thing; but it stands for a whole collection of monstrously big things, all too big to really think about properly. (Well, Cantor did!:)

5. That's too crazy, it seems; but, nonetheless, mathematicians know that it's true, because we can prove it! The key to determining if two sets have the same size is the one-to-one correspondence -- and that is key for infinite sets, as well.

6. So, in the end, here's what you say on the playground, when you want to love your friend more:

   "I love you more than the power set of your set of infinite love."

   

   Amen!