Day 4, Mat129: Calculus I

By the way, the quiz will be over section 1.5.

1. wrap up section 1.5
2. start section 1.6

I pushed back your 1.5 assignment, now due Thursday.

The most important definition in calculus is the derivative function (here is the derivative of $f$ at $x$):

And that's why we're so concerned about limits! Memorize it. Be able to write it at a moment's notice.

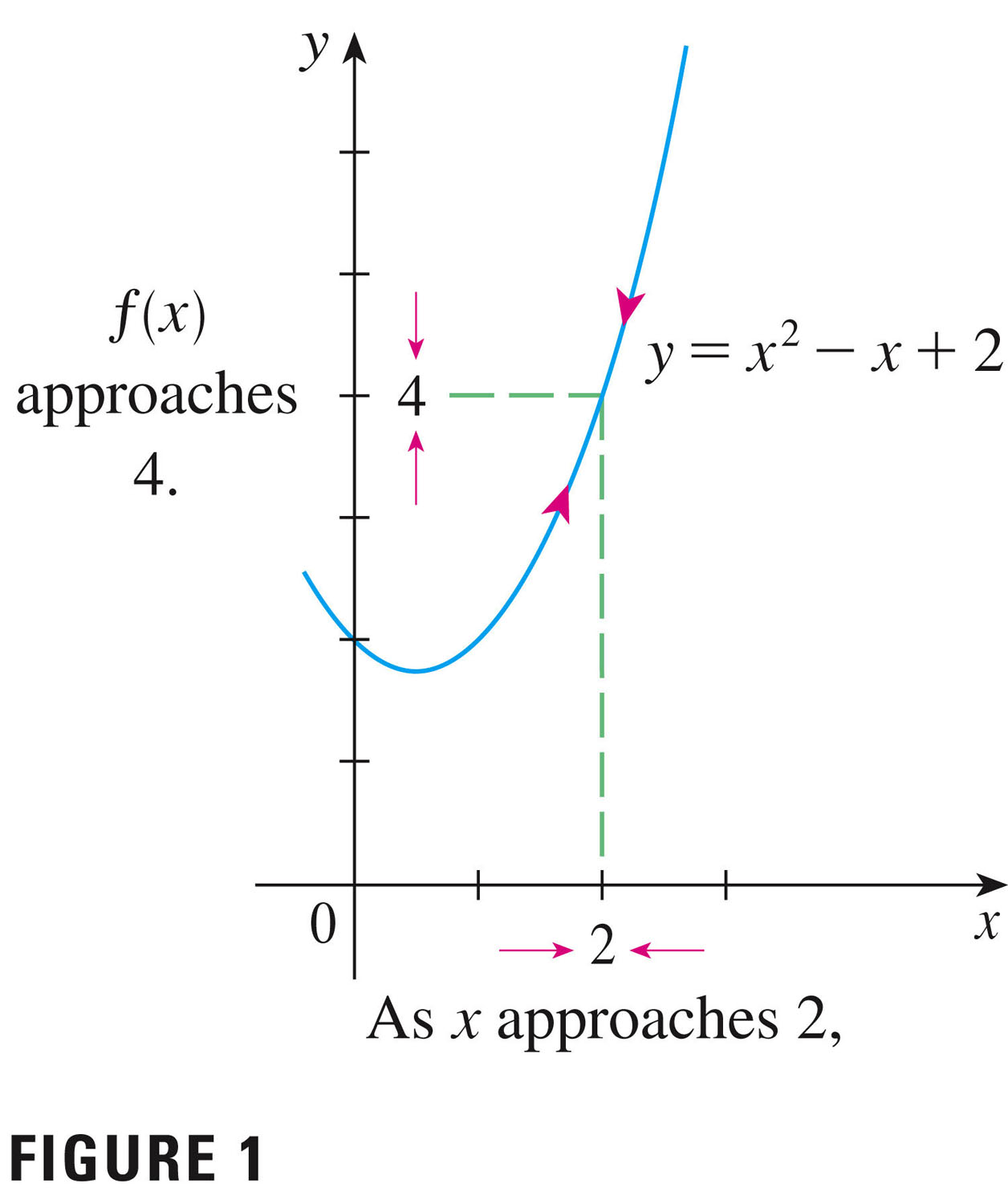
We can approach $x=a$ from the left or from the right. We define limits from the left and from the right, and then say that the limit exists as $x$ approaches $a$ if and only if the limits from the left and right exist, and agree: if \[ \lim\limits_{x \to a^-}f(x) = L \] and \[ \lim\limits_{x \to a^+}f(x) = L \] then \[ \lim\limits_{x \to a}f(x) = L \]

We had a look at a few problems from the text (pp. 59--), and discovered that, if we have the graph of a function, then the limits are pretty easy to see.

Let's check out some Mathematica examples from section 1.5, and check out not only the limits, but also what dangers lurk, even when we have very good technology (see Example 2, Figure 5, p. 52). You can't always trust your calculator; trust your brain first.

We'll be using Mathematica extensively in this class. Have I told you that you have the right to a free copy of Mathematica?

• Let's check the last of the examples from section 1.5, to get started. Mathematica tells one more lie!

  So limits may be infinite (one-sided, perhaps). Here's how we define that:

  infinite limits for $\displaystyle f(x)$ as $\displaystyle x$ approaches $\displaystyle a$: \[ \lim\limits_{x \to a}f(x) = \infty \] means that the values of $\displaystyle f(x)$ can be made arbitrarily large (as large as we please) by taking $\displaystyle x$ sufficiently close to $\displaystyle a$ (but not equal to $\displaystyle a$). Similarly we can define \[ \lim\limits_{x \to a}f(x) = -\infty \] and one-sided limits such as \[ \lim_{x \to a^-}f(x) = \infty {\hspace{1.5in}} \lim\limits_{x \to a^+}f(x) = \infty \]

  

  In any of these cases, we define a vertical asymptote of the curve $\displaystyle y=f(x)$ at $\displaystyle x=a$.

• #18, p. 60 -- be creative!
• #26, p. 60
• #35, p. 61
• #42, p. 61 -- one more dirty rotten lie!

  Note the symmetry, which allows us to check only one side.

  Symmetry is a very important (and under-discussed) aspect of mathematics. Keep an eye on even and odd functions.

• We start with two especially trivial special limits:
  • Constant rule $(f(x)=c)$: $\lim\limits_{x\to{a}}c=c$

    Here the function $f(x)=1$ is plotted. As $x \to 0$, the $y$-values head to 1. Well, more to the point, they never vary from 1! Quite a boring function....

  • Identity rule $(f(x)=x)$: $\lim\limits_{x\to{a}}x=a$

    Here the function $f(x)=x$ is plotted. As $x \to 0$, the $y$-values head to 0. And the closer $x$ gets to 0, the closer $y$ gets to 0. That's the way it's supposed to work! Also a rather boring function: so predictable!

  What can we do with those? We need more horsepower....

• Sum law: "the limit of a sum is the sum of the limits"

  So what is this limit:

  $\lim\limits_{x\to{a}}(x+c)$

  This sum (and many other operations) satisfy the same pattern:

  "The limit of a STUFF is the STUFF of the limits."

  So, in particular,

  "The limit of a sum is the sum of the limits."
  $\lim\limits_{x\to{a}}(x+c)=\lim\limits_{x\to{a}}x+\lim\limits_{x\to{a}}c=a+c$

• Difference law
• Product law
• Constant multiple law:
• Quotient law (slight caveat here -- no zero denominators)

  Suppose that $\lim_{x \to a} f(x)$ and $\lim_{x \to a} g(x)$ exist. Then \[ \lim_{x \to a} [f(x)+g(x)] = \lim_{x \to a} f(x) + \lim_{x \to a} g(x) \] \[ \lim_{x \to a} [f(x)-g(x)] = \lim_{x \to a} f(x) - \lim_{x \to a} g(x) \] \[ \lim_{x \to a} [cf(x)] = c\lim_{x \to a} f(x) \] \[ \lim_{x \to a} [f(x)g(x)] = \lim_{x \to a} f(x) \lim_{x \to a} g(x) \] \[ \lim_{x \to a} \frac{f(x)}{g(x)} = \frac{\lim\limits_{x \to a} f(x)}{\lim\limits_{x \to a} g(x)} {\text{ if }} \lim_{x \to a} g(x) \ne 0 \]

• Power law \[ \lim_{x \to a} [f(x)]^n = [\lim_{x \to a} f(x)]^n \] where $n$ is a positive integer (the limit of a power is the power of the limit).

• Root law: \[ \lim_{x \to a} \sqrt[n]{f(x)} = \sqrt[n]{\lim_{x \to a} f(x)} \] where $n$ is a positive integer. Again, we can ``pass the limit inside''. Again, a caveat: why can't we compute

  \[ \lim\limits_{x\to{0}}\sqrt{x} \]

  Because the function isn't even defined as x approaches 0 from the left (square roots of negative numbers are imaginary). So we have only a one-sided limit, that \[ \lim\limits_{x\to{0^+}}\sqrt{x}=0 \] Of course we can say that \[ \lim\limits_{x\to{1}}\sqrt{x}=1 \] Limits exists everywhere else on the domain of the sqrt function.

• Putting these laws together
  • Now that we've got the sum and product and constant multiple rules, what can we deduce?
  • "Affine" rule, for one:
    \[ \lim\limits_{x\to{a}}(mx+b)=ma+b \]

  • Then we can proceed to deduce that all polynomials have limits, and furthermore that
    $\lim\limits_{x\rightarrow{a}}P(x)=P(a)$

    This is the conclusion of Exercise #55, p. 71, which says that polynomials are continuous, which we'll consider more in section 1.8.

    Exercise #56 says it's also true for rational functions, for every element of their domain.

• Take a look at a couple of the examples in the text, examples 5 and 6 on page 66. These both illustrate the limiting process applied to the most important definition in calculus:
  $f'(x)=\lim\limits_{h\rightarrow{0}}{\frac{f(x+h)-f(x)}{h}}$

  In this case, both show examples of a slightly different version of this most important definition, the derivative at a point $x=a$:

  $f'(a)=\lim\limits_{h\to 0}{\frac{f(a+h)-f(a)}{h}}$

• Other Laws
  • The squeeze theorem What can we say about the limit of g as x approaches a?

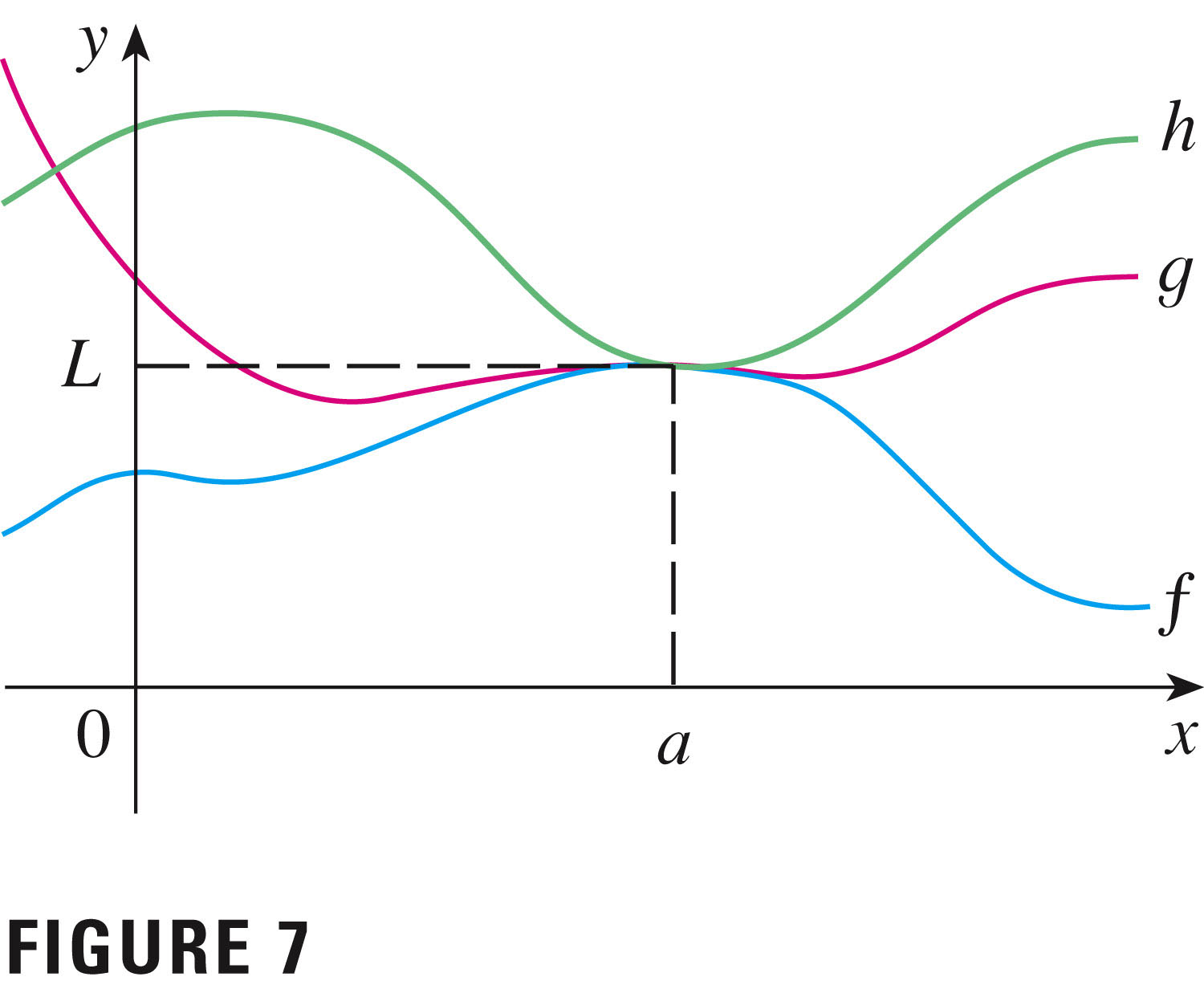
    What's happening to the $y$-values?

• Now let's take a look at some of the examples from the section exercises.
  • Example 3, p. 65
  • #7, p. 69
  • #10
  • #25
  • #33
  • #47
  • #54

• Additional examples:
  • #63, p. 71
  • #59, p. 71 (squeeze it!)