Day 46 in MAT229

Last time

Next time

Today:

Announcements:
- Your exams are returned. You have two grades now, which are averaged (although the basement is 50, so the function (!) I used to compute your score is ${f(x,y)=(max(50,x)+y)/2}$
- Your 11.5 homework is returned.
- Today we're going to get into 11.8, on power series.
Section 11.7: Strategies for testing series
- Let's take a look at some problems. Pick examples from p. 764-765 which look interesting.
Section 11.8: Power series
- Summary:
  Now, rather than simply consider infinite sums of numbers, we're going to consider an infinite sum of functions in what are known as power series. They're like polynomials, but they don't have finite degree.
  The terms will be monomials -- functions with are simply constants times powers of x.
  Now we realize that, with functions for terms, each value of x specified gives rise to an infinite series, and we might immediately wonder if the series is convergent for a particular choice of ${x}$ .
  So what kind of function has an infinite number of terms? How do you evaluate such a thing? What kinds of function are these? It turns out that a lot of our old friends can be expressed this way
  ${F(x)={e^x}}$ , for example, as we've seen.
  Now what did we assert about ${F(x)={e^x}}$ ? That
  ${e^x=\sum_{n=0}^{\infty}{\frac{x^n}{n!}}}$
  For what values of x would this converge?
  
  Let's take a look at how well a truncated power series -- a partial sum -- approximates the real function.
  1. Graph ${e^x}$ on the interval [-2,2].
  2. Graph ${p_1(x)=\sum_{n=0}^{1}\frac{x^n}{n!}}$
  3. Graph ${p_2(x)=\sum_{n=0}^{2}\frac{x^n}{n!}}$
  4. Graph ${p_3(x)=\sum_{n=0}^{3}\frac{x^n}{n!}}$
  What do you see happening?
  
  Apply the ratio test for arbitrary x, and what do you discover?
  
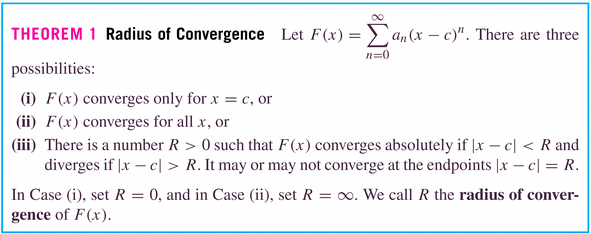
  So ${F(x)={e^x}}$ presents us with a case where we have an "infinite radius of convergence".
  
  Interesting sidelight: you may have encountered Euler's Formula at some point in your life:
  
  ${e^{i\theta}=\cos(\theta)+i\sin(\theta)}$
  where ${i=\sqrt{-1}}$ . From this (and the power series for ${e^x}$ ) we can now deduce power series for the functions cosine and sine. Let's do that.
  Excuse me for saying, but this is very cool. It illustrates for you, as perhaps nothing ever has, the value of using imaginary (and complex) numbers.
  
  Let's look at an example where our radius of convergence is not so nice: consider ${F(x)=\frac{1}{1-x}}$ . One can show that
  
  ${\frac{1}{1-x}=\sum_{n=0}^{\infty}{\qquad{x^n}}}$ In fact we deduced this for a particular case when we looked at geometric series: that ${\frac{1}{1-r}=\sum_{n=0}^{\infty}{\qquad{r^n}}}$ But now we're talking about a function of x. One can think of x as representing allowable values of r (that is, values of r for which this geometric series converges). We know that this is for values of r such that .
  
  Now: for what values of x do you think that this will converge?
  
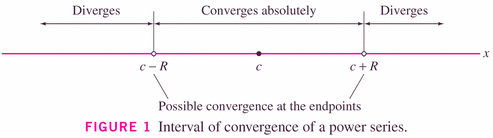
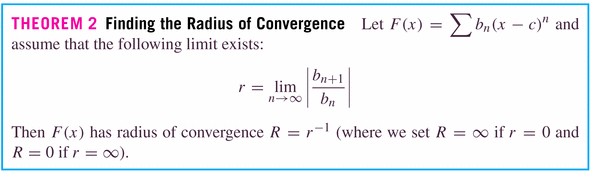
  Note that this is a variation on the theme of the ratio test: we don't consider the power terms, but only the coefficients.
- Examples:
  - What does Theorem 2 say will be the radius of convergence of the series for ${F(x)=\frac{1}{1-x}}$ ?
  - What about the endpoints?
  - How well do the truncated partial sum functions approximate ${F(x)=\frac{1}{1-x}}$ ?
  - #7, p. 769
  - #19
  - #27

Website maintained by Andy Long. Comments appreciated.
Updated on 09/30/2014 23:35:07