- Your Egyptian Multiplication (and Facebooks) homeworks have been
graded (key
here).
I thought that I'd use this homework as something of a motivation for why symmetry is important and useful, so let's look at that Facebook problem, and see how to use symmetry to help organize our Facebook world....
- Your Egyptian division homework is due tonight. One thing that
spooked one of your classmate was that there is no unique way
to represent a given fraction as a sum of unit fractions!
So, for example, \[ \frac{1}{2} = \frac{1}{3}+\frac{1}{6} \] and it turns out that, since \[ \frac{1}{a} = \frac{1}{a+1}+\frac{1}{a(a+1)} \] for any positive integer $a$, we can replace the $\frac{1}{6}$ above as \[ \frac{1}{2} = \frac{1}{3}+\left(\frac{1}{7}+\frac{1}{6*7}\right) = \frac{1}{3}+\frac{1}{7}+\frac{1}{42} \] and (do it again, do it again) you have an infinite number of ways of writing any fraction as a sum of unit fractions!
This was no doubt a source of consternation for the Egyptians. We don't know exactly how they came up with their representations in the Rhid papyrus, but we speculate that they tried to find the representation with the fewest number of terms.
Important: You can always check that you're right when you do an Egyptian division by simply computing both sides and seeing if they're equal!
Upshot: uniqueness is really helpful; uniqueness is a big deal.
- Materials for today:
- Here are a couple of videos:
- Here are a couple of docs:
Hermann Weyl (German Mathematician; 1885 - 1955)
- You have been assigned parts of this
worthy handout for homework (see the assignment page). It covers two kinds of
symmetry that are very important: rotational and reflective.
- Are
humans symmetric? Humans are purported to have a "good side"
and an "evil side"!
- Symmetry appears everywhere in nature. We'll see
three-dimensional versions of that today.
But Weyl's classic Symmetry gives us some beautiful examples (e.g. pages 59, 75).
- Are
humans symmetric? Humans are purported to have a "good side"
and an "evil side"!
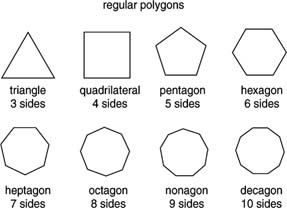
- We begin our discussion with the convex regular
polygons:
"A regular polygon is a polygon which is equiangular (all angles are equal in measure) and equilateral (all sides have the same length). Regular polygons may be convex or star."
- Last time we answered the following questions in the plane of 2-Dimensions:
- What is the most symmetric rectangle?
- What are regular polygons?
- How many regular polygons are there?
- What kinds of symmetry do they possess?
- Which regular polygons can be used to tile (tessellate) the plane?
- What could you do with a heptagon?
- As an example, consider regular convex hexagonal
graph paper, which represents a tiling of the plane by a
regular convex polygon. You see these kinds of tilings in bathrooms.
Bees have the same idea:

- The honeycomb is actually solid -- three-dimensional. The tubes
have hexagonal walls. But the comb is not Platonic.
A cube is an example of a Platonic solid. It's the one we're most familiar with, so let's start with that.
A Platonic solid is a solid for which
- All faces are congruent (identical) convex regular polygons, and
- Each face, each edge, and each vertex is exactly equivalent to every other face, edge, or vertex (respectively).
The Five Convex Regular Polyhedra (Platonic solids) -- thanks Wikipedia! Tetrahedron Hexahedron
or CubeOctahedron Dodecahedron Icosahedron 



fire earth air universe water - What do these have to do with Plato? According to Weyl, "Plato, in the
dialogue Timaeus, associates the regular pyramid, octahedron,
cube, icosahedron, with the four elements of fire, air, earth and water
(in this order), while in the pentagondodecahedron he sees in some sense
the image of the universe as a whole."
- As a "homework" (for your own good!), I am asking you to cut and
create Platonic solids out of paper, using this template. You
may use these as a cheat sheet for the next exam. You must have put
them together, however, and you must use only your own.
- What's wrong with six sided polygons, and beyond? Consider the
hexagonal graph paper, of the tiling of the plane: why can't it be folded up
into a solid?
- What can you do with these solids?
- Play basketball? Three Sides to This Story: Knicks Dust Off
Phil Jackson's Triangle Offense, from the New York Times:

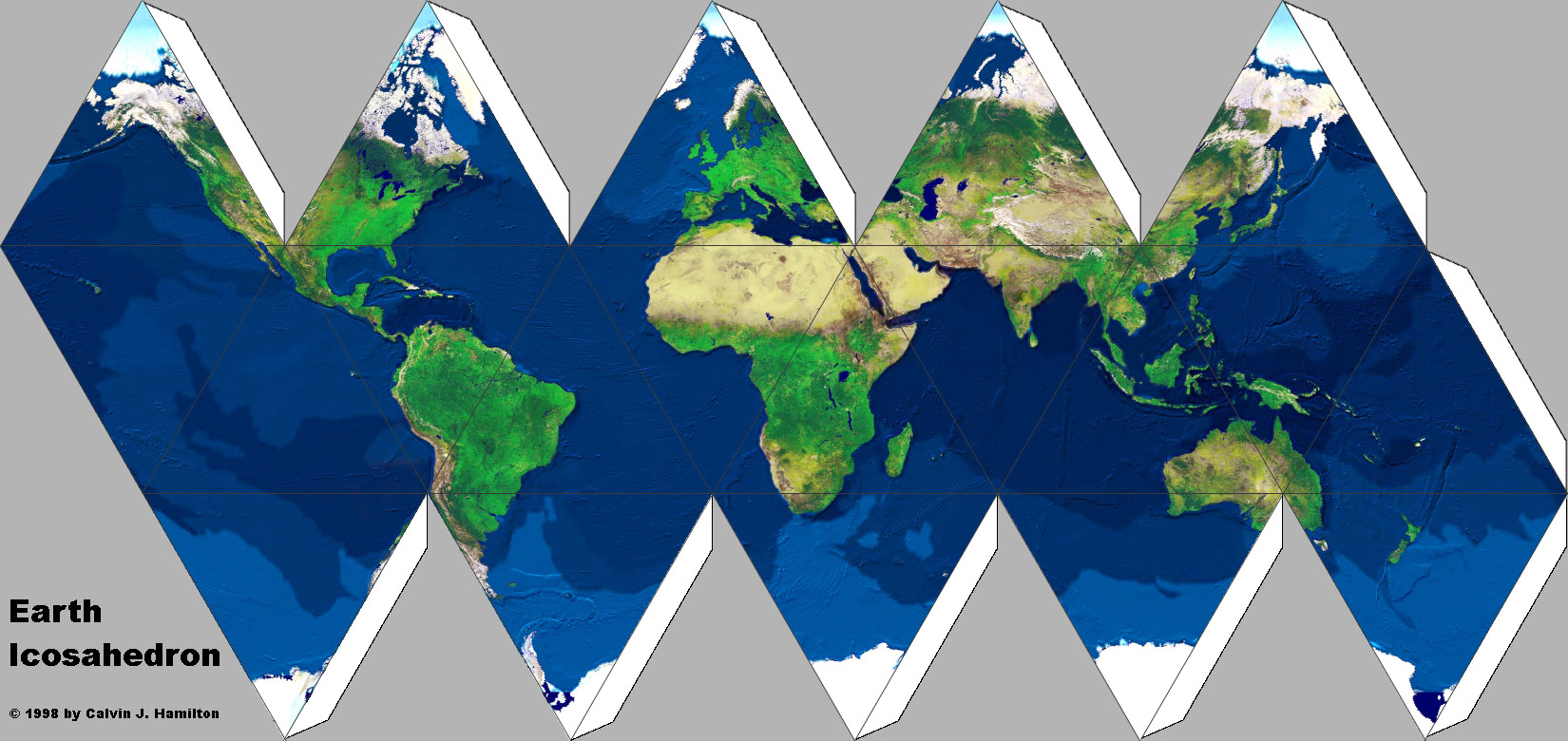
- Map the Earth?


The Platonic solids allow us to make "flat spheres" (sort of!):
- Johannes Kepler thought that the orbits of the planets (of which
five were known at the time) were somehow related to the various
Platonic solids, as shown in
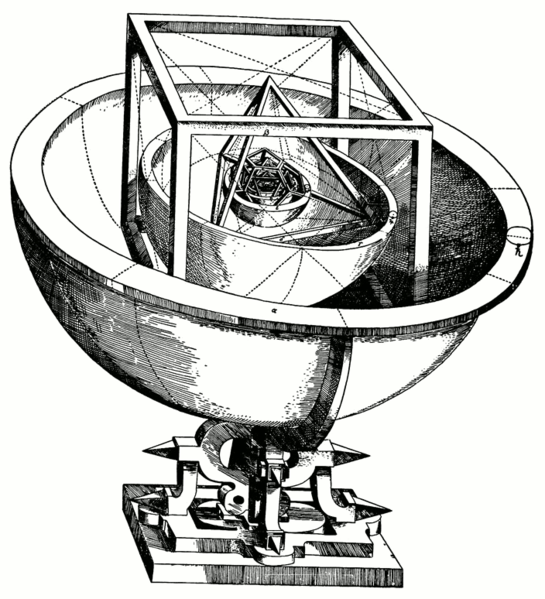
"The six spheres each corresponded to one of the planets (Mercury, Venus, Earth, Mars, Jupiter, and Saturn). The solids were ordered with the innermost being the octahedron, followed by the icosahedron, dodecahedron, tetrahedron, and finally the cube. In this way the structure of the solar system and the distance relationships between the planets was dictated by the Platonic solids."
And then Uranus was discovered, and there was not sixth Platonic solid. And so science evolves....
- Something interesting to share: great images from Linus Pauling's and Roger Hayward's The Architecture of Molecules (a book created especially for young people, and based off of Pauling's article in the 1964 Proceedings of the National Academy of Sciences.
These are some higher quality images than my scans:
- So what can you do with a dodecahedron? Look like a super-brainy, cool scientist!
From the October 7th, 2011 New York Times
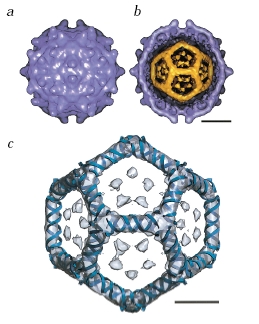
- Icosahedron
- amoeboid protozoa Circogonia icosahedra
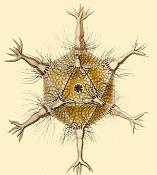
- Viruses:
- The Cliff Notes Version...

"An array of viruses. (a) The helical virus of rabies. (b) The segmented helical virus of influenza. (c) A bacteriophage with an icosahedral head and helical tail. (d) An enveloped icosahedral herpes simplex virus. (e) The unenveloped polio virus. (f) The icosahedral human immunodeficiency virus with spikes on its envelope."
- Bacteriophages and Virions
- The Cliff Notes Version...
- Dodecahedral
- amoeboid protozoa Circogonia icosahedra
- More critters:
- Play basketball? Three Sides to This Story: Knicks Dust Off
Phil Jackson's Triangle Offense, from the New York Times:
- Now let's use what we can deduce of Platonic solids to fill in the
following table:
# of Vertices Edges Faces faces at each vertex sides at each face Tetrahedron Cube Octahedron Dodecahedron Icosahedron What conclusions can we draw from this data? Is there a pattern? (Of course there is!:) The pattern leads to the concept of "Duality":
- Each Platonic solid has a "twin" -- called its dual -- which we can discover from the table.

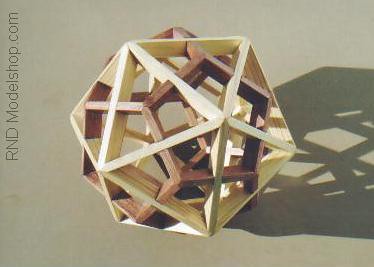
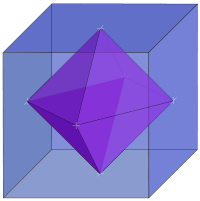
- Each Platonic solid has a "twin" -- called its dual -- which we can discover from the table.