- This session will be captured on Zoom, if
I remember to turn it on, and record it. Please help me to remember.
Here's a menu of our past zooms...
I'll post the link to today's later. These zooms will continue through February 7th, for those who can't attend in person.
- For today, you were to read Section 1.3 in
our text, and carry out Preview Activity 1.3.1.
Bring the work on the Preview Activities to class. I just want to get a look at your work.
- You were also asked to carry out the following two exercises (due next today,
at 2:00 pm (before class), to submit on Canvas): Section 1.1,
#8; Section 1.2, #7.
We'll talk about those on Wednesday, after I've got them graded.
- For next time: no new reading. We're going to continue discussing Section
1.3: The derivative of a function at a point.
Your "preview" for Wednesday is to "Explore" two of the "GeoGebra" examples mentioned in this section (there are questions at the end of each); these are the work of Marc Renault, at Shippensburg University:
- I'd like you to use Desmos in class next time (or something
similar, so that you can do some graph); bring a laptop or
tablet, if you can. Otherwise, maybe you can lean over the
shoulder of someone who's got such a device -- from six feet
away, of course!:)
- Roll
- I've graded your homeworks. If you didn't submit a preview, I
dinged you a couple of points.
If you left sections of your worksheet blank, you might have been dinged more.
At this point, I'm really just hoping that you'll get going! :) Please try to keep up.
- Let's talk about your
- preview, and
- worksheets that I graded. Here is my attempt....
I'll have you start by bringing out your preview activity, and perhaps chatting with a neighbor about it for a few minutes. If any questions arise, we'll address those in five minutes.
That didn't get done! (I should have realized when why you all didn't recognize this notation: \[ \lim_{x \to 5^-}f(x) \] and \[ \lim_{x \to 5^+}f(x) \] etc.
These are called "one-sided limits". Let's look at Definition 1.2.2, and the example 1.2.3 that follows, to understand these limits (or at least start to get some intuition).
This figure presents us with the basic idea:
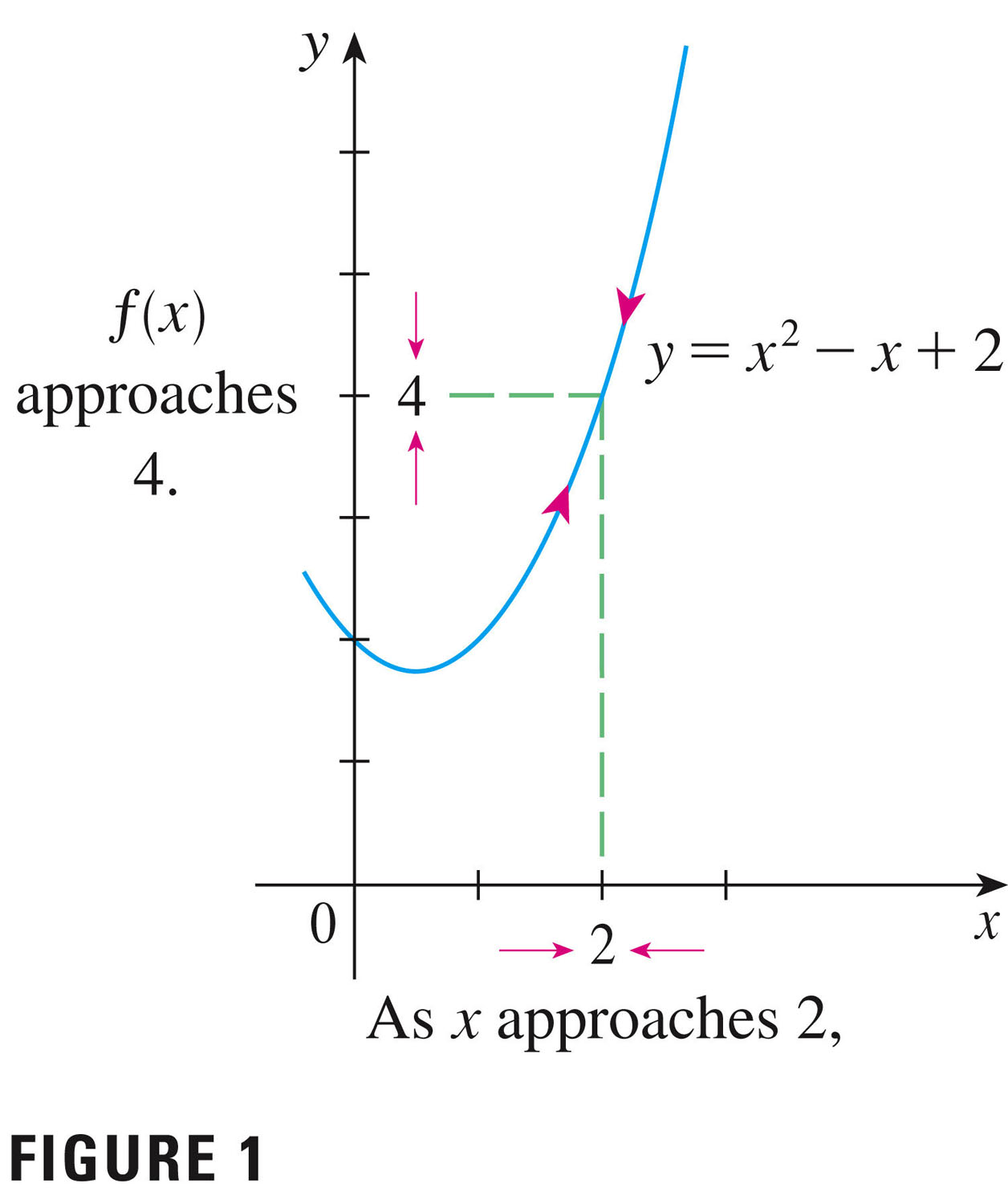
limit of $f(x)$ as $x$ approaches $a$: Suppose function $f(x)$ is defined when $x$ is near the number $a$ (this means that $f$ is defined on some open interval that contains $a$, except possibly at $a$ itself.) Then we write \[ \lim_{x \to a}f(x) = L \] if we can make the values of $f(x)$ arbitrarily close to $L$ by taking $x$ to be sufficiently close to $a$ but not equal to $a$. We say that ``the limit of $f(x)$ as $x$ approaches $a$ equals $L$.'' The intuitive idea is that in the neighborhood of $a$, the function $f$ takes on values close to $L$.
Questions:
- In the figure above ("FIGURE 1"), what is $a$, and what is $L$?
- To what class of functions does $f$ belong?
- Do limits (i.e. do we) even care about what happens exactly at $a$?
We can approach $x=a$ from the left or from the right. We define limits from the left and from the right, and then say that the limit exists as $x$ approaches $a$ if and only if the limits from the left and right exist, and agree: if the limit as $x$ approaches $a$ from the left, \[ \lim_{x \to a^-}f(x) = L \] and the limit as $x$ approaches $a$ from the right, \[ \lim_{x \to a^+}f(x) = L \] then the limit as $x$ approaches $a$ exists, and \[ \lim_{x \to a}f(x) = L. \]
- Why are we concerned with "limits"? Here's why:
The most important definition in calculus is the definition of the derivative (here is the derivative of $f$ at $a$):
$f'(a)=\lim_{h\to 0}{\frac{f(a+h)-f(a)}{h}}$
And that's why we're so concerned about limits! Memorize this definition. Be able to write it at a moment's notice.We've seen this in another form as well:
$f'(a)=\lim_{x\to a}{\frac{f(x)-f(a)}{x-a}}$ This was defined as the slope of the tangent line to a curve (provided the tangent line exists).
- First point: the derivative may fail to exist at a point (and we
talked last time about the various ways it could fail).
In particular, from a graphical perspective, if there's no way to assign a tangent line, then the derivative cannot exist at a point.
One of the interesting problems we've seen is that of a discontinuity in a function: not all functions are smooth, or even connected! What that means is that the definition above may not make sense in some cases. What can go wrong? Three problems have become apparant:
- The limit as $x$ approaches $a$ may not exist from left or right;
- The limits may exist, but disagree;
- The function value may not exist at $x=a$, or may not be equal to the limit.
- Let's Review a few things, stemming from this "limit definition of the derivative":
- Function representation -- tables, graphs, algebraic expressions
- Variables -- independent and dependent. [In the first limit above, the independent variable is actually $h$ for a fixed value of $x$.]
- Domains and Ranges
- Function composition (remember this in the case of "frequency modulation" of a sine wave in our "sound functions".
- Ratios!
- Indeterminate things... (division by 0)
In particular, we're only going to have indeterminacy in our derivative if the numerator goes to 0 when the denominator goes to 0. But that only happens if \[ \lim_{h\to 0}(f(a+h)-f(a))=0 \] This happens when the limit exists, and is equal to the function value. This is equivalent to \[ \lim_{h\to 0}f(a+h)=f(a) \] If this is true, then we say that $f$ is continuous at $a$.
We see, therefore, that a derivative exists at $a$ only if the function is continuous there. But not vice versa. A function continuous everywhere does not necessarily have a derivative everywhere -- can you think of one?
- Now what is our first practical illustration of limits? The
calculation of slopes (and thus equations) of tangent lines.
The derivative of a function at a point gives the slope of the tangent line there -- provided it exists.
We've already seen how the secant lines approach the tangent line for a smooth curve. It's one of the first important problems we'll want to address in calculus. It's why we're interested in limits of things at the outset.
We usually find the equation of a line using two points, or a point and a slope. The secant line method approaches the tangent line at a point by using a succession of nearby points that are ever closer to the point of tangency: see, for example, this code suggested by our authors, the work of David Austin of Grand Valley State University:
The secant line method approaching the tangent line
The tangent ("touching") line osculates ("kisses") the curve at this point.
Notice the focus on linear functions: linear functions are the most important functions in calculus.
Once we have the derivative at $x=a$, we can write the equation of the tangent line, graph of linear function $T(x)$, using "point-slope" form: \[ T(x) - f(a) = f'(a)(x-a), \] or \[ T(x) = f'(a)(x-a) + f(a). \] It's that simple!
- Infinite limits
- Limits may be infinite (one-sided, perhaps). Here's how we define that:
infinite limits for $\displaystyle f(x)$ as $\displaystyle x$ approaches $\displaystyle a$: \[ \lim_{x \to a}f(x) = \infty \] means that the values of $\displaystyle f(x)$ can be made arbitrarily large (as large as we please) by taking $\displaystyle x$ sufficiently close to $\displaystyle a$ (but not equal to $\displaystyle a$). Similarly we can define \[ \lim_{x \to a}f(x) = -\infty \] and one-sided limits such as \[ \lim_{x \to a^-}f(x) = \infty {\hspace{1.5in}} \lim_{x \to a^+}f(x) = \infty \]

In any of these cases, we define a vertical asymptote of the curve $\displaystyle y=f(x)$ at $\displaystyle x=a$.
- Limits may be infinite (one-sided, perhaps). Here's how we define that:
- Then it's on to today's activities, which involve solidifying your
understanding of these ideas. Your
worksheet for today.
- My freshman physics professor at Miami University, Prof. Don C. Kelly wrote The Little Book of Calculus. Self-Help Guide to Calculus. I just found this yesterday, on his website, and it's a neat "little" (50 some pages) resource produced by him and his kids/grandkids. Check out page 12, limits....