- 2.3 homework was returned last Thursday: graded the first two
problems.
- You have an assignment to hand in a week from now (section
2.4). You also have some IMath due Friday, so please keep an eye on
that.
- Please put your rectangles away.
- The chain rule is so critically important because of the
importance of compositions of functions: for
$F(x) = f(g(x))$,
$F^\prime(x) = f^\prime(g(x))g^\prime(x)$ I personally think this to myself: "f prime of stuff times the derivative of the stuff"; or "f prime of stuff times stuff prime.",
where $f$ is the "outside" function:
$F^\prime(x) = f^\prime(stuff)stuff^\prime$ You can see that the rule is fairly simple, once you've identified the composition -- that is, once you've torn apart $F$ to find $f$ and $g$.
You might take a look at this summary from my pre-calc class to review compositions.
- In Leibniz notation, if $y=f(u)$ and $u=g(x)$ are both differentiable
functions, then
\[
\frac{dy}{dx} = \frac{dy}{du} \frac{du}{dx}
\]
This alternative version of the formula helps us to remember that $x$
is going on a journey, passing first through $g$ to become something
new ($u=g(x)$), and then continues on as $u$ through the function $f$
to become $y=f(u)$. It's a metamorphosis. It should be called
the "metamorphosis rule", if there were any justice in the world....
- The difficulty is often identifying the composition. We'll try
some easy ones first, then get to some ugly ones.
Before we do that, however, I'd like to motivate (rather than prove) the chain rule, using the limit definition of the derivative. Everything comes from that!
Basically, however, it relies on the tangent line: we want to use the fact that
$f(x+h) \approx f(x) + hf'(x)$ Why does that make sense? Because it comes straight out of the limit definition, where we throw away the limit. That's why we have to write "$\approx"$:
$f'(x) \approx \frac{f(x+h)-f(x)}{h}$ - #9, p. 154
- #16
- #29 -- the chains can get longer!
- #77
- A Chain Rule tutorial
- A sample problem involving compositions: model the seasonal variation in Keeling's CO2 data:
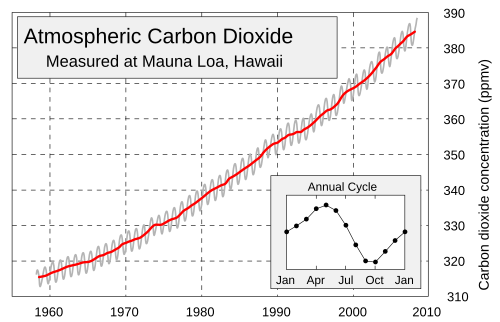
- Keeling data with models:
If time were measured in years from January, would
$V(t)=A\sin(2\pi{t})$ be a good model? What would be a good choice for the parameter $A$?