- There's a major/minor/calculus student lunch this Friday, 11:30-1:30, upstairs by the elevators. Make sure that you stop and get something to eat. You don't even have to talk about math!
- Theorems:

- if the series of the
if finite, so is the smaller series of
;
- if the series of the
if infinite, so is the larger series of
.
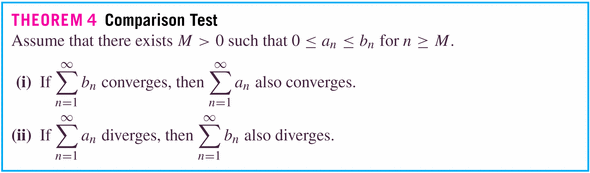
- geometric series, and
- p series, including
- the harmonic series (to show divergence).
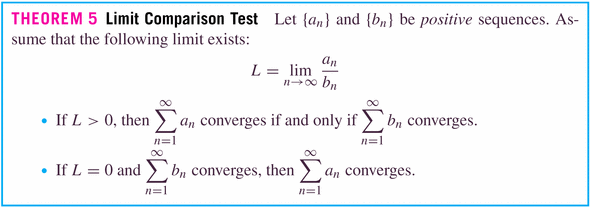
This theorem says that, in the long run, one series has terms which are simply a constant times the terms in the other series.
In "the long run" means that we only really need to worry about the "tails" of series: we can throw away any finite number of terms for issues of convergence.
- if the series of the
- Examples:
- #6, p. 750
- #16
- #28
- #34 (draw a picture)
- #46 (this is a cool argument -- pay attention!)
-
Once again, our mission here is not necessarily the value of the
series, but may be simply knowledge of whether it converges or
not.
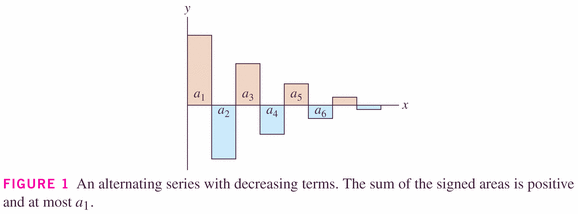
- How does the author of this image reach the conclusion that "the sum is positive and at most
"?
- How about the partial sums --
-- what are they doing?
- What are the even partial sums doing?
- What are the odd partial sums doing?
- Examples:
- The alternating harmonic series is convergent
(Example 1, p. 753).
We need to show that the terms
of the series satisfy the Leibniz test:
- the terms are positive,
- the terms are decreasing in size,
- the terms have limit of 0.
- #5, p. 755
Identify the terms
, and decide whether they satisfy the Leibniz test.
- #13
- #17
Remember that for convergence, the conditions of the test need be true only eventually: convergence is all about the tail, not the head of the sequence.
- #23
For this one, we need the "Alternating Series Estimation Theorem" (p. 754):
- #27
- #34
- The alternating harmonic series is convergent
(Example 1, p. 753).
That being said, a theorem below does give us some help in determining the value of (or at least bounds on the value of) the series of a type called an "alternating" series. In particular, we want to look at alternating series like the one below:
So it turns out that if the terms are converging to 0, then the alternating sequence converges to a limit.
Now we usually write our alternating series in the following way, illustrated by Leibniz's test: we assume that the are positive, and the term
takes care of the "alternation". (Our textbook switches to
for the positive part of the term.)
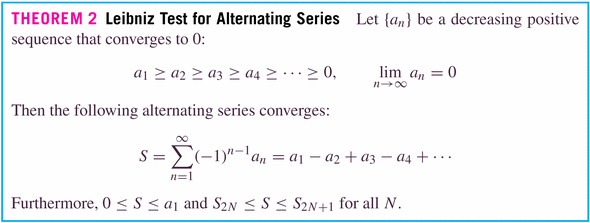
Our textbook calls this the "Alternating Series Test" (p. 751). The "Furthermore" part our textbook calls the "Alternating Series Estimation Theorem" (p. 754).