- Your quiz this week will cover section1.8: the tangent line approximation.
- You have a new assignment, corresponding to Section 2.1.
- I summarized key points from 1.7, continuity and limits, and
- showed off a graphic from the current issue of Science:
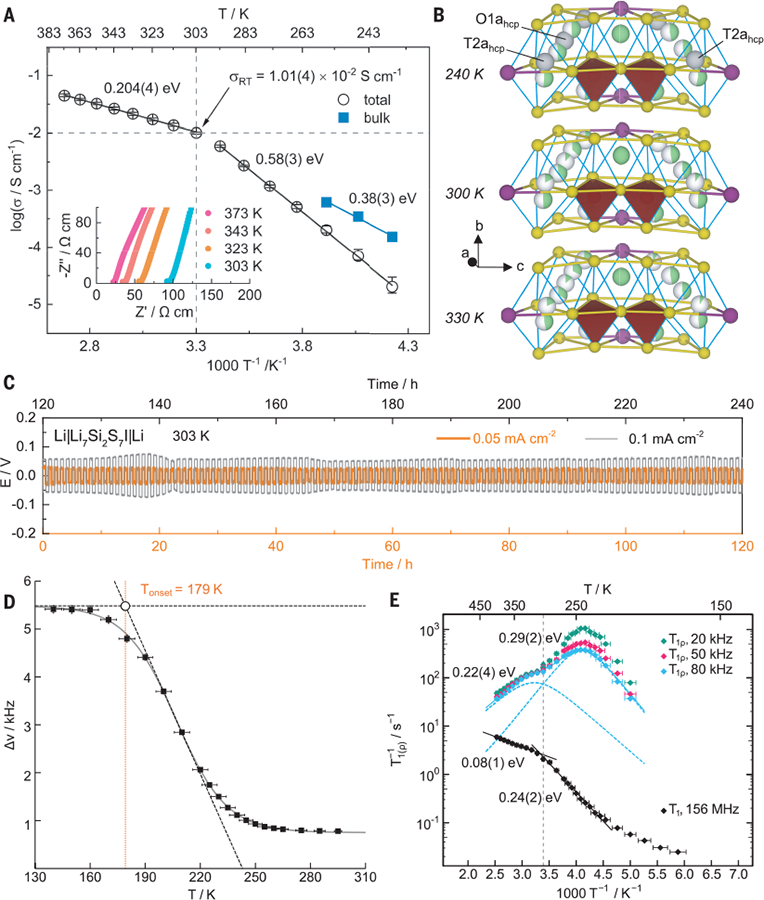
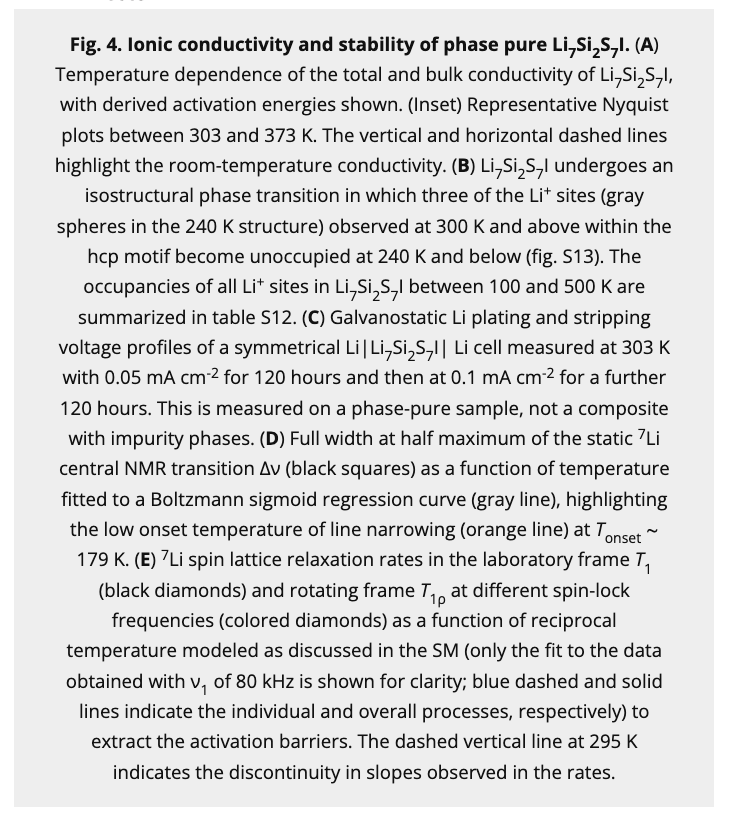
illustrating important aspects of our course so far, including an important inflection point, concavity, the use of the tangent line, and also shows how well a tangent line can approximate a function over quite a wide interval. The function is "locally linear", and "locally' extends pretty far....
I grabbed some of the Supplementary material from the Science website, and found the model for the function shown above:
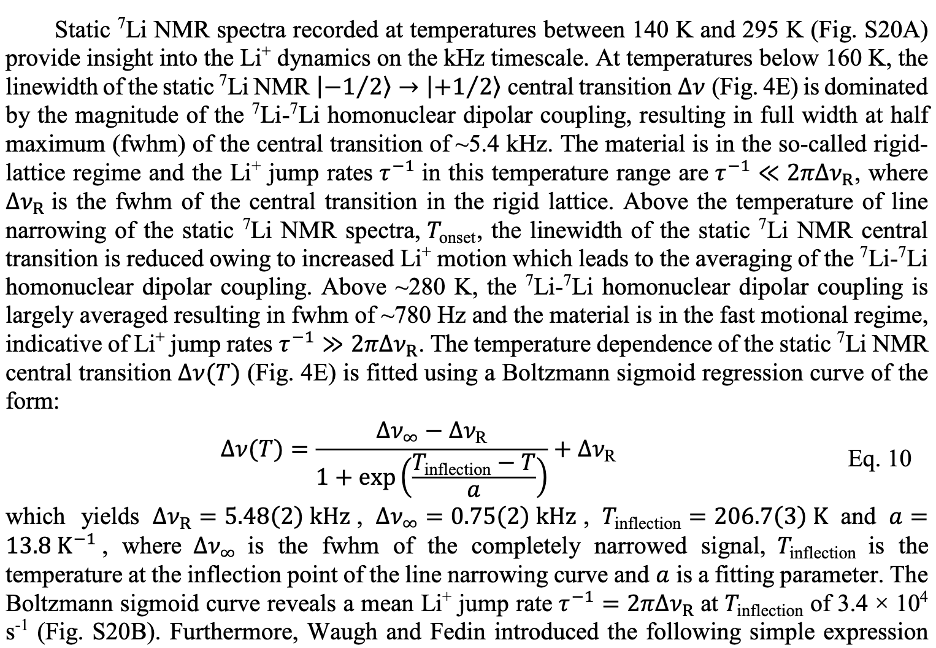
Is it easier to compute that or a straight line, do you think?
- We started Section
1.8: The Tangent Line Approximation: linear functions are
good approximations to smooth functions (functions with
derivatives), if you zoom in close enough:
\[ f(x) \approx L(x) \equiv f'(a)(x-a)+f(a) \]
- Your quizzes from last time....
- Today we continue with Section 1.8.
We ended last time on an example: we were trying to get a good approximation to \(\sqrt{15.96}\), and leveraging our knowledge of what's going on just a little down the road ("locally"), at \(x=16\):
So back to our example: compute the derivative of $f(x)=\sqrt{x}$ at $x=16$: \[ f'(x)=\frac{1}{2\sqrt{x}} \] so \(f'(16)=\frac{1}{8}\), and we can write down the tangent line equation: \[ L(x) = \frac{1}{8}(x-16) + 4 \]
- What's the approximate value? (3.995000)
- What's the actual value? (3.994996) -- (we're off by about 4 millionths)
- We use the tangent line for our approximation, and, when
we're done, we can ask "is our answer an over- or an under-estimate?"
The answer takes us back to the second derivative:
- Let's have a look at today's worksheet (you'll be excited to know
that there are more potato problems!:): we'll try some activities and homework problems
from section 1.8.
- Leftover Potatoes
- Your quizzes from last time....
